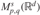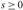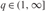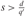Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chaichenets, L
and
Pattakos, N
2023.
Higher-order NLS with anisotropic dispersion and modulation spaces: a global existence and scattering result.
The Quarterly Journal of Mathematics,
Vol. 74,
Issue. 3,
p.
1015.