Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-12-03T00:36:34.170Z Has data issue: false hasContentIssue false
  • English
  • Français

Factorization of Matrices and Minkowski's Conjecture

Published online by Cambridge University Press:  18 May 2009

A. M. Macbeath
A. M. Macbeath
Affiliation:
Queen's College, Dundee
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let Rn denote real Euclidean space of n dimensions. If

define 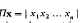, and (as usual) 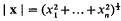so that, by the inequality of arithmetic and geometric means,

Let Λ0 be the integer lattice, consisting of those points in Rn whose co-ordinates are integers. A non-singular n × n matrix M will be called a Minkowski matrix, if, for any point a ∈ Rn, there exists a point x ∈ Λ0 such that

It was shown by Minkowski that, when n = 2, every non-singular matrix is a Minkowski matrix, and that, for general n, every rational non-singular matrix is a Minkowski matrix. Minkowski is also said to have conjectured that every non-singular matrix is a Minkowski matrix, whatever the value of n. For n = 3, this was proved by Remak [5], and a much simpler proof was given later by Davenport [2]. For n = 4, it was proved by Dyson [3], who used a method similar to that of Remak and Davenport, but required the methods of algebraic topology to deal with some of the complications which arise in the higher dimension. Since this method depends also on the reduction of quadratic forms, it is quite likely that it might fail for higher values of n even if the topological difficulties could be overcome. Therefore, an alternative proof for n = 3, due to Birch and Swinnerton-Dyer [1], is of some interest, though in this dimension it is more complicated than Davenport's proof. A good deal of their analysis applies to general n, and they showed that for all n there is a neighbourhood of the unit matrix I that consists entirely of Minkowski matrices.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 5 , Issue 2 , July 1961 , pp. 86 - 89
Copyright
Copyright © Glasgow Mathematical Journal Trust 1961

References

1.Birch, B. J. and Swinnerton-Dyer, H. P. F., On the inhomogeneous minimum of the product of n linear forms, Mathematika 3 (1956), 25–39.CrossRefGoogle Scholar
2.Davenport, H., A simple proof of Remak's theorem on the product of three linear forms, J. London Math. Soc. 14 (1938), 47–51.Google Scholar
3.Dyson, F. J., On the product of four non-homogeneous linear forms, Ann. of Math. (2) 49, (1948), 82–109.CrossRefGoogle Scholar
4.Hardy, G. H. and Wright, E. M., An introduction to the theory of numbers (Oxford, 1938).Google Scholar
5.Remak, R., Verallgemeinerung eines Minkowskischen Satzes II, Math. Z. 18 (1923), 173–200.CrossRefGoogle Scholar