No CrossRef data available.
Article contents
EXPLICIT CONSTRUCTION OF COMPANION BASES
Part of:
Algebraic combinatorics
Arithmetic rings and other special rings
Lie algebras and Lie superalgebras
Published online by Cambridge University Press: 21 July 2015
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
A companion basis for a quiver Γ mutation equivalent to a simply-laced Dynkin quiver is a subset of the associated root system which is a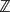 
$\mathbb{Z}$-basis for the integral root lattice with the property that the non-zero inner products of pairs of its elements correspond to the edges in the underlying graph of Γ. It is known in type A (and conjectured for all simply-laced Dynkin cases) that any companion basis can be used to compute the dimension vectors of the finitely generated indecomposable modules over the associated cluster-tilted algebra. Here, we present a procedure for explicitly constructing a companion basis for any quiver of mutation type A or D.
MSC classification
Primary:
13F60: Cluster algebras
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2015
References
REFERENCES
1.Barot, M., Geiss, C. and Zelevinsky, A., Cluster algebras of finite type and positive symmetrizable matrices, J. London Math. Soc. 73 (3) (2006), 545–564.CrossRefGoogle Scholar
2.Barot, M. and Marsh, R., Reflection group presentations arising from cluster algebras, Trans. Amer. Math. Soc. 367 (2015), 1945–1967.Google Scholar
3.Bastian, J., Holm, T. and Ladkani, S., Derived equivalences for cluster-tilted algebras of Dynkin type D, J. Algebra. 410 (2014), 277–332.CrossRefGoogle Scholar
4.Buan, A., Marsh, R. and Reiten, I., Cluster-tilted algebras, Trans. Amer. Math. Soc. 359 (2007), 323–332.CrossRefGoogle Scholar
5.Buan, A., Marsh, R. and Reiten, I., Cluster mutation via quiver representations, Comment. Math. Helv. 83 (2) (2008), 143–177.Google Scholar
6.Buan, A., Marsh, R. and Reiten, I., Cluster-tilted algebras of finite representation type, J. Algebr. 306 (2) (2006), 412–431.Google Scholar
7.Buan, A. and Vatne, D., Derived equivalence classification for cluster-tilted algebras of type An, J. Algebr. 319 (7) (2008), 2723–2738.Google Scholar
8.Butler, M. C. R. and Ringel, C. M., Auslander-Reiten sequences with few middle terms and applications to string algebras, Comm. Algebr. 15 (1987), 145–179.Google Scholar
9.Caldero, P., Chapoton, F. and Schiffler, R., Quivers with relations arising from clusters (An case), Trans. Amer. Math. Soc. 358 (3) (2006), 1347–1364.Google Scholar
10.Caldero, P., Chapoton, F. and Schiffler, R., Quivers with relations and cluster-tilted algebras, Algebr. Represent. Theory 9 (4) (2006), 359–376.CrossRefGoogle Scholar
11.Derksen, H., Weyman, J. and Zelevinsky, A., Quivers with potentials and their representations I: Mutations, Sel. Math. (N.S.) 14 (1) (2008), 59–119.Google Scholar
12.Fomin, S. and Zelevinsky, A., Cluster algebras II: Finite type classification, Invent. Math. 154 (1) (2003), 63–121.Google Scholar
13.Nájera, A.Chávez, C-vectors and dimension vectors for cluster-finite quivers, Bull. London Math. Soc. 45 (3) (2013), 1259–1266.Google Scholar
14.Nakanishi, T. and Stella, S., Diagrammatic description of c-vectors and d-vectors of cluster algebras of finite type, Electron. J. Combin. 21 (1) (2014), #P1.3.Google Scholar
15.Parsons, M. J., On indecomposable modules over cluster-tilted algebras of type A, PhD Thesis (University of Leicester, 2007).Google Scholar
16.Parsons, M. J., Companion bases for cluster-tilted algebras, Algebr. Represent. Theory 17 (3) (2014), 775–808. doi:10.1007/s10468-013-9418-y.Google Scholar
18.Vatne, D. F., The mutation class of Dn quivers, Comm. Algebr. 38 (3) (2010), 1137–1146.Google Scholar
You have
Access