No CrossRef data available.
Article contents
Bilinear forms on vector Hardy spaces
Published online by Cambridge University Press: 18 May 2009
Abstract
Let φ: 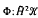 ℋ →
ℋ →  be a bilinear form on vector Hardy space. Introduce the symbol φ of Φ by (φ (Z1, Z2), a ⊗ b) = Φ (K21 ⊗ a, K22 ⊗ b ), where Kw is the reproducing kernel for w ∈ D. We show that Φ extends to a bounded bilinear form on
be a bilinear form on vector Hardy space. Introduce the symbol φ of Φ by (φ (Z1, Z2), a ⊗ b) = Φ (K21 ⊗ a, K22 ⊗ b ), where Kw is the reproducing kernel for w ∈ D. We show that Φ extends to a bounded bilinear form on  provided that the gradient
provided that the gradient 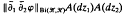 defines a Carleson measure in the bidisc D2. We obtain a sufficient condition for Φ to extend to a Hilbert space. For vectorial bilinear Hankel forms we obtain an analogue of Nehari's Theorem.
defines a Carleson measure in the bidisc D2. We obtain a sufficient condition for Φ to extend to a Hilbert space. For vectorial bilinear Hankel forms we obtain an analogue of Nehari's Theorem.
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 1997


