Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-28T17:42:14.920Z Has data issue: false hasContentIssue false
  • English
  • Français

Abelian actions on compact nonorientable Riemann surfaces

Part of: Riemann surfaces Other groups of matrices Low-dimensional topology Special aspects of infinite or finite groups

Published online by Cambridge University Press:  02 December 2021

Jesús Rodríguez Open the ORCID record for Jesús Rodríguez [Opens in a new window]
Jesús Rodríguez*
Affiliation:
Departamento de Matemáticas Fundamentales, Facultad de Ciencias Universidad Nacional de Educación a Distancia, 28040 Madrid, Spain
*
Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Given an integer 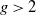$g>2$ , we state necessary and sufficient conditions for a finite Abelian group to act as a group of automorphisms of some compact nonorientable Riemann surface of genus g. This result provides a new method to obtain the symmetric cross-cap number of Abelian groups. We also compute the least symmetric cross-cap number of Abelian groups of a given order and solve the maximum order problem for Abelian groups acting on nonorientable Riemann surfaces.

Keywords

Nonorientable surfaceKlein surfaceAutomorphism groupSymmetric cross-cap number

MSC classification

Primary: 57M60: Group actions in low dimensions
Secondary: 20F05: Generators, relations, and presentations 20H10: Fuchsian groups and their generalizations 30F50: Klein surfaces
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 64 , Issue 3 , September 2022 , pp. 634 - 648
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of Glasgow Mathematical Journal Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alling, N. L. and Greenleaf, N., Foundations of the theory of Klein surfaces , Lecture Notes in Mathematics, vol. 219 (Springer-Verlag, Berlin-New York, 1971).Google Scholar
Breuer, T., Characters and automorphism groups of compact Riemann surfaces, vol. 280, London Mathematical Society Lecture Note Series (Cambridge University Press, Cambridge, 2000).Google Scholar
Bujalance, E., Cyclic groups of automorphisms of compact nonorientable Klein surfaces without boundary, Pacific J. Math. 109(2) (1983), 279–289.CrossRefGoogle Scholar
Bujalance, E., A note on the group of automorphisms of a compact Klein surface, Rev. Real Acad. Cienc. Exact. Fís. Natur. Madrid 81(3) (1987), 565–569.Google Scholar
Bujalance, E., Cirre, F. J., Etayo, J. J., Gromadzki, G., and Martínez, E., A survey on the minimum genus and maximum order problems for bordered Klein surfaces, in Groups St Andrews 2009 in Bath. Volume 1, vol. 387, London Math. Soc. Lecture Note Ser. (Cambridge Univ. Press, Cambridge, 2011), 161–182.CrossRefGoogle Scholar
Bujalance, E., Cirre, F. J., Etayo, J. J., Gromadzki, G., and Martínez, E., Automorphism groups of compact non-orientable Riemann surfaces, in Groups St Andrews 2013, vol. 422, London Math. Soc. Lecture Note Ser. (Cambridge Univ. Press, Cambridge, 2015), 183–193.Google Scholar
Bujalance, E., Cirre, F. J., and Gromadzki, G., A survey of research inspired by Harvey’s theorem on cyclic groups of automorphisms, in Geometry of Riemann surfaces, vol. 368, London Math. Soc. Lecture Note Ser. (Cambridge Univ. Press, Cambridge, 2010), 15–37.CrossRefGoogle Scholar
Bujalance, E., Etayo, J. J., Gamboa, J. M., and Gromadzki, G., Automorphism groups of compact bordered Klein surfaces. A combinatorial approach, vol. 1439, Lecture Notes in Mathematics (Springer-Verlag, Berlin, 1990).CrossRefGoogle Scholar
Gromadzki, G., Abelian groups of automorphisms of compact nonorientable Klein surfaces without boundary, Comment. Math. Prace Mat. 28(2) (1989), 197–217.Google Scholar
Harvey, W. J., Cyclic groups of automorphisms of a compact Riemann surface, Quart. J. Math. Oxford Ser. (2) 17 (1966), 86–97.CrossRefGoogle Scholar
Magnus, W., Karrass, A., and Solitar, D., Combinatorial group theory, Second revised ed. (Dover Publications Inc., New York, 1976).Google Scholar
Newman, M., Integral matrices (Academic Press, New York-London, 1972). Pure and Applied Mathematics, Vol. 45.Google Scholar
Rodríguez, J., Some results on abelian groups of automorphisms of compact Riemann surfaces, in Riemann and Klein surfaces, automorphisms, symmetries and moduli spaces, vol. 629. Contemp. Math. (Amer. Math. Soc., Providence, RI, 2014), 283–297.CrossRefGoogle Scholar
Rodríguez, J., Abelian actions on compact bordered Klein surfaces. Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Math., 111(1) (2017), 189–204.CrossRefGoogle Scholar
Singerman, D., Automorphisms of compact non-orientable Riemann surfaces. Glasgow Math. J. 12 (1971), 50–59.Google Scholar
Smith, H. J. S., On systems of linear indeterminate equations and congruences. Philos. Trans. R. Soc. Lond. 151(1861), 293–326.Google Scholar