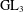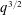Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jiang, Yujiao
and
Lü, Guangshi
2021.
Cancellation in algebraic twisted sums on GL_
m
.
Forum Mathematicum ,
Vol. 33,
Issue. 4,
p.
1061.
Kowalski, Emmanuel
Lin, Yongxiao
and
Michel, Philippe
2023.
Rankin-Selberg coefficients in large arithmetic progressions.
Science China Mathematics,
Vol. 66,
Issue. 12,
p.
2767.
Lin, Yongxiao
and
Michel, Philippe
2024.
On algebraic twists with composite moduli.
The Ramanujan Journal,
Vol. 63,
Issue. 3,
p.
803.
Kumar, Sumit
Munshi, Ritabrata
and
Kumar Singh, Saurabh
2024.
Subconvexity bound for GL(3) ×GL(2)L-functions : Hybrid level aspect.
Algebra & Number Theory,
Vol. 18,
Issue. 3,
p.
477.