Article contents
KAROUBI’S RELATIVE CHERN CHARACTER, THE RIGID SYNTOMIC REGULATOR, AND THE BLOCH–KATO EXPONENTIAL MAP
Published online by Cambridge University Press: 20 August 2014
Abstract
We construct a variant of Karoubi’s relative Chern character for smooth separated schemes over the ring of integers in a 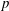 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$-adic field, and prove a comparison with the rigid syntomic regulator. For smooth projective schemes, we further relate the relative Chern character to the étale
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$-adic field, and prove a comparison with the rigid syntomic regulator. For smooth projective schemes, we further relate the relative Chern character to the étale 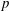 $p$-adic regulator via the Bloch–Kato exponential map. This reproves a result of Huber and Kings for the spectrum of the ring of integers, and generalizes it to all smooth projective schemes as above.
$p$-adic regulator via the Bloch–Kato exponential map. This reproves a result of Huber and Kings for the spectrum of the ring of integers, and generalizes it to all smooth projective schemes as above.
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/3.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author 2014
References
- 1
- Cited by


