Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-26T07:33:16.507Z Has data issue: false hasContentIssue false
  • English
  • Français

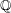HODGE IDEALS FOR $\mathbb{Q}$-DIVISORS, 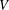$V$-FILTRATION, AND MINIMAL EXPONENT

Part of: Families, fibrations Surfaces and higher-dimensional varieties (Co)homology theory Singularities

Published online by Cambridge University Press:  17 April 2020

MIRCEA MUSTAŢĂ  and
MIHNEA POPA
MIRCEA MUSTAŢĂ
Affiliation:
Department of Mathematics, University of Michigan, Ann Arbor, MI 48109, USA; [email protected]
MIHNEA POPA
Affiliation:
Department of Mathematics, Northwestern University, 2033 Sheridan Road, Evanston, IL 60208, USA; [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

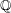We compute the Hodge ideals of $\mathbb{Q}$-divisors in terms of the 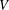$V$-filtration induced by a local defining equation, inspired by a result of Saito in the reduced case. We deduce basic properties of Hodge ideals in this generality, and relate them to Bernstein–Sato polynomials. As a consequence of our study we establish general properties of the minimal exponent, a refined version of the log canonical threshold, and bound it in terms of discrepancies on log resolutions, addressing a question of Lichtin and Kollár.

MSC classification

Primary: 14F10: Differentials and other special sheaves; D-modules; Bernstein-Sato ideals and polynomials 14J17: Singularities 32S25: Surface and hypersurface singularities 14D07: Variation of Hodge structures
Type
Algebraic and Complex Geometry
Information
Forum of Mathematics, Sigma , Volume 8 , 2020 , e19
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2020

References

Bernšteĭn, I. N., ‘Analytic continuation of generalized functions with respect to a parameter’, Funkcional. Anal. Priložen. 6(4) (1972), 26–40.Google Scholar
Budur, N., ‘Multiplier ideals, V-filtration, and spectrum’, J. Algebraic Geom. 14(2) (2005), 269–282.CrossRefGoogle Scholar
Björk, J.-E., Rings of Differential Operators, North-Holland Mathematical Library, 21 (North-Holland Publishing Co., Amsterdam-New York, 1979).Google Scholar
de Fernex, T., Ein, L. and Mustaţă, M., ‘Shokurov’s ACC conjecture for log canonical thresholds on smooth varieties’, Duke Math. J. 152(1) (2010), 93–114.CrossRefGoogle Scholar
Dimca, A., Maisonobe, P., Saito, M. and Torrelli, T., ‘Multiplier ideals, V-filtrations and transversal sections’, Math. Ann. 336(4) (2006), 901–924.Google Scholar
Ein, L., Lazarsfeld, R., Smith, K. E. and Varolin, D., ‘Jumping coefficients of multiplier ideals’, Duke Math. J. 123(3) (2004), 469–506.CrossRefGoogle Scholar
Hotta, R., Takeuchi, K. and Tanisaki, T., D-Modules, Perverse Sheaves, and Representation Theory, (Birkhäuser, Boston, 2008).CrossRefGoogle Scholar
Jung, S.-J., Kim, I.-K., Saito, M. and Yoon, Y., ‘Hodge ideals and spectrum of isolated hypersurface singularities’, Preprint, arXiv:1904.02453, 2019.Google Scholar
Kashiwara, M., ‘B-functions and holonomic systems. Rationality of roots of B-functions’, Invent. Math. 38(1) (1976/77), 33–53.CrossRefGoogle Scholar
Kashiwara, M., ‘Vanishing cycle sheaves and holonomic systems of differential equations’, inAlgebraic Geometry, Lecture Notes in Mathematics, 1016 (Springer, Berlin, 1983), 134–142.CrossRefGoogle Scholar
Kollár, J., ‘Singularities of pairs’, inAlgebraic Geometry, Santa Cruz 1995, Proc. Sympos. Pure Math., 62 (American Mathematical Society, Providence, RI, 1997), 221–287.CrossRefGoogle Scholar
Lazarsfeld, R., Positivity in Algebraic Geometry II, Ergebnisse der Mathematik und ihrer Grenzgebiete, 49 (Springer, Berlin, 2004).CrossRefGoogle Scholar
Lichtin, B., ‘Poles of |f (z, w)|2s and roots of the b-function’, Ark. Mat. 27(2) (1989), 283–304.CrossRefGoogle Scholar
Loeser, F., ‘Exposant d’Arnold et sections planes’, C. R. Acad. Sci. Paris Sér. I Math. 298(19) (1984), 485–488.Google Scholar
Malgrange, B., ‘Polynômes de Bernstein–Sato et cohomologie évanescente’, inAnalysis and Topology on Singular Spaces, II, III (Luminy, 1981), Astérisque, 101 (Soc. Math. France, Paris, 1983), 243–267.Google Scholar
Maxim, L., Saito, M. and Schürmann, J., ‘Thom–Sebastiani theorems for filtered 𝒟-modules and for multiplier ideals’, Int. Math. Res. Not. IMRN (1) (2020), 91–111.CrossRefGoogle Scholar
Mustaţă, M. and Popa, M., ‘Restriction, subadditivity, and semicontinuity theorems for Hodge ideals’, Int. Math. Res. Not. IMRN (11) (2018), 3587–3605.CrossRefGoogle Scholar
Mustaţă, M. and Popa, M., ‘Hodge ideals’, Mem. Amer. Math. Soc. 262(1268) (2019), v+80 pp.Google Scholar
Mustaţă, M. and Popa, M., ‘Hodge ideals for ℚ-divisors: birational approach’, J. École Polytechnique (6) (2019), 283–328.CrossRefGoogle Scholar
Sabbah, C., ‘𝒟-modules et cycles évanescents’, inGéométrie algébrique et applications III, La Rábida (1984), (eds. Malgrange, B. and Kashiwara, M.) Traveaux en Cours, 24 (Hermann, Paris, 1984), 53–98.Google Scholar
Saito, M., ‘Hodge filtrations on Gauss-Manin systems. I’, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 30(3) (1984), 489–498.Google Scholar
Saito, M., ‘Modules de Hodge polarisables’, Publ. Res. Inst. Math. Sci. 24(6) (1988), 849–995.CrossRefGoogle Scholar
Saito, M., ‘Mixed Hodge modules’, Publ. Res. Inst. Math. Sci. 26(2) (1990), 221–333.CrossRefGoogle Scholar
Saito, M., ‘On b-function, spectrum and rational singularity’, Math. Ann. 295(1) (1993), 51–74.Google Scholar
Saito, M., ‘On microlocal b-function’, Bull. Soc. Math. France 122(2) (1994), 163–184.CrossRefGoogle Scholar
Saito, M., ‘On the Hodge filtration of Hodge modules’, Mosc. Math. J. 9(1) (2009), 161–191.Google Scholar
Saito, M., ‘Hodge ideals and microlocal $V$-filtration’, Preprint, arXiv:1612.08667, 2016.Google Scholar
Steenbrink, J. H. M., ‘Semicontinuity of the singularity spectrum’, Invent. Math. 79(3) (1985), 557–565.CrossRefGoogle Scholar
Zhang, M., ‘Hodge filtration and Hodge ideals for $\mathbb{Q}$-divisors with weighted homogeneous isolated singularities’, Preprint, arXiv:1810.06656, 2018.Google Scholar