GLOBAL ACTIONS AND VECTOR 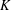$K$-THEORY

Abstract

Purely algebraic objects like abstract groups, coset spaces, and G-modules do not have a notion of hole as do analytical and topological objects. However, equipping an algebraic object with a global action reveals holes in it and thanks to the homotopy theory of global actions, the holes can be described and quantified much as they are in the homotopy theory of topological spaces. Part I of this article, due to the first author, starts by recalling the notion of a global action and describes in detail the global actions attached to the general linear, elementary, and Steinberg groups. With these examples in mind, we describe the elementary homotopy theory of arbitrary global actions, construct their homotopy groups, and revisit their covering theory. We then equip the set 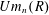$Um_{n}(R)$ of all unimodular row vectors of length $n$ over a ring 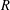$R$ with a global action. Its homotopy groups 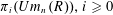$\unicode[STIX]{x1D70B}_{i}(Um_{n}(R)),i\geqslant 0$ are christened the vector $K$-theory groups 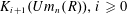$K_{i+1}(Um_{n}(R)),i\geqslant 0$ of 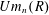$Um_{n}(R)$. It is known that the homotopy groups 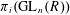$\unicode[STIX]{x1D70B}_{i}(\text{GL}_{n}(R))$ of the general linear group $\text{GL}_{n}(R)$ viewed as a global action are the Volodin 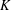$K$-theory groups $K_{i+1,n}(R)$. The main result of Part I is an algebraic construction of the simply connected covering map 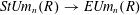$\mathit{StUm}_{n}(R)\rightarrow \mathit{EUm}_{n}(R)$ where 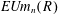$\mathit{EUm}_{n}(R)$ is the path connected component of the vector 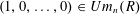$(1,0,\ldots ,0)\in Um_{n}(R)$. The result constructs the map as a specific quotient of the simply connected covering map $St_{n}(R)\rightarrow E_{n}(R)$ of the elementary global action 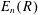$E_{n}(R)$ by the Steinberg global action $St_{n}(R)$. 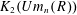As expected, $K_{2}(Um_{n}(R))$ is identified with 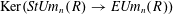$\text{Ker}(\mathit{StUm}_{n}(R)\rightarrow \mathit{EUm}_{n}(R))$. Part II of the paper provides an exact sequence relating stability for the Volodin 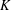$K$-theory groups 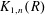$K_{1,n}(R)$ and 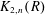$K_{2,n}(R)$ to vector 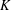$K$-theory groups.