Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T12:15:20.922Z Has data issue: false hasContentIssue false
  • English
  • Français

GENERALIZED EXPLICIT DESCENT AND ITS APPLICATION TO CURVES OF GENUS 3

Part of: Arithmetic problems. Diophantine geometry Arithmetic algebraic geometry Curves

Published online by Cambridge University Press:  17 February 2016

NILS BRUIN ,
BJORN POONEN  and
MICHAEL STOLL
NILS BRUIN
Affiliation:
Department of Mathematics, Simon Fraser University, Burnaby, BC V5A 1S6, Canada; [email protected]
BJORN POONEN
Affiliation:
Department of Mathematics, Massachusetts Institute of Technology, Cambridge, MA 02139-4307, USA; [email protected]
MICHAEL STOLL
Affiliation:
Mathematisches Institut, Universität Bayreuth, 95440 Bayreuth, Germany; [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

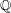We introduce a common generalization of essentially all known methods for explicit computation of Selmer groups, which are used to bound the ranks of abelian varieties over global fields. We also simplify and extend the proofs relating what is computed to the cohomologically defined Selmer groups. Selmer group computations have been practical for many Jacobians of curves over  $\mathbb{Q}$ of genus up to 2 since the 1990s, but our approach is the first to be practical for general curves of genus 3. We show that our approach succeeds on some genus 3 examples defined by polynomials with small coefficients.

MSC classification

Primary: 11G30: Curves of arbitrary genus or genus $ne 1$ over global fields
Secondary: 11G10: Abelian varieties of dimension $> 1$ 14G25: Global ground fields 14H45: Special curves and curves of low genus
Type
Research Article
Information
Forum of Mathematics, Sigma , Volume 4 , 2016 , e6
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2016

References

Atiyah, M. F. and Wall, C. T. C., ‘Cohomology of groups’, inAlgebraic Number Theory (Proc. Instructional Conf., Brighton, 1965) (Thompson, Washington, DC, 1967), 94–115.Google Scholar
Baran, B., ‘An exceptional isomorphism between modular curves of level 13’, J. Number Theory 145 (2014), 273–300.Google Scholar
Baran, B., ‘An exceptional isomorphism between level 13 modular curves via Torelli’s theorem’, Math. Res. Lett. 21(5) (2014), 919–936.Google Scholar
Bhargava, M., Gross, B. H. and Wang, X., ‘Arithmetic invariant theory II: Pure inner forms and obstructions to the existence of orbits’, inRepresentations of Reductive Groups, (eds. Nevins, M. and Trapa, P. E.) Progress in Mathematics, 312 (Springer International Publishing, Boston, 2015), 139–171.CrossRefGoogle Scholar
Bosch, S., Lütkebohmert, W. and Raynaud, M., Néron Models, Ergebnisse der Mathematik und ihrer Grenzgebiete (3) (Results in Mathematics and Related Areas (3)), 21 (Springer, Berlin, 1990).Google Scholar
Bruin, N. and Stoll, M., ‘Two-cover descent on hyperelliptic curves’, Math. Comp. 78(268) (2009), 2347–2370.Google Scholar
Deligne, P. and Mumford, D., ‘The irreducibility of the space of curves of given genus’, Publ. Math. Inst. Hautes Études Sci. (36) (1969), 75–109.Google Scholar
Demazure, M., ‘Résultant, discriminant’, Enseign. Math. (2) 58(3–4) (2012), 333–373.Google Scholar
Dixmier, J., ‘On the projective invariants of quartic plane curves’, Adv. Math. 64(3) (1987), 279–304.Google Scholar
Djabri, Z., Schaefer, E. F. and Smart, N. P., ‘Computing the p-Selmer group of an elliptic curve’, Trans. Amer. Math. Soc. 352(12) (2000), 5583–5597.Google Scholar
Flynn, E. V., Poonen, B. and Schaefer, E. F., ‘Cycles of quadratic polynomials and rational points on a genus-2 curve’, Duke Math. J. 90(3) (1997), 435–463.Google Scholar
Geißler, K., ‘Berechnung von Galoisgruppen über Zahl- und Funktionenkörpern’, PhD Thesis, Technische Universität Berlin, 2003, available at http://www.math.tu-berlin.de/ kant/publications/diss/geissler.pdf.Google Scholar
Geissler, K. and Klüners, J., ‘Galois group computation for rational polynomials’, J. Symbolic Comput. 30(6) (2000), 653–674. Algorithmic methods in Galois theory.Google Scholar
Gelfand, I. M., Kapranov, M. M. and Zelevinsky, A. V., Discriminants, Resultants and Multidimensional Determinants, Modern Birkhäuser Classics (Birkhäuser Boston Inc., Boston, MA, 2008), reprint of the 1994 edition.Google Scholar
Gille, P. and Szamuely, T., Central Simple Algebras and Galois Cohomology, Cambridge Studies in Advanced Mathematics, 101 (Cambridge University Press, Cambridge, 2006).Google Scholar
Gross, B. H. and Harris, J., ‘On some geometric constructions related to theta characteristics’, inContributions to Automorphic Forms, Geometry, and Number Theory (Johns Hopkins University Press, Baltimore, MD, 2004), 279–311.Google Scholar
Hartshorne, R., Algebraic Geometry, Graduate Texts in Mathematics, 52 (Springer, New York, 1977).Google Scholar
Kohel, D. R., Algorithms for elliptic curves and higher dimensional analogues (Echidna). Available at http://echidna.maths.usyd.edu.au/kohel/alg/index.html.Google Scholar
Lang, S., Abelian Varieties (Springer, New York, 1983), reprint of the 1959 original.Google Scholar
Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system. I. The user language’, J. Symbolic Comput. 24(3–4) (1997), 235–265. Computational algebra and number theory (London, 1993). Magma is available at http://magma.maths.usyd.edu.au/magma/.Google Scholar
McCallum, W. and Poonen, B., ‘The method of Chabauty and Coleman’, inExplicit Methods in Number Theory: Rational Points and Diophantine Equations, Panoramas et Synthèses, 36 (Société Mathématique de France, Paris, 2012), 99–117.Google Scholar
Milne, J. S., Arithmetic Duality Theorems, 2nd edn, (BookSurge, LLC, 2006).Google Scholar
Mordell, L. J., ‘On the rational solutions of the indeterminate equations of the third and fourth degrees’, Proc. Cambridge Phil. Soc. 21 (1922), 179–192.Google Scholar
Mumford, D., Abelian Varieties, Tata Institute of Fundamental Research Studies in Mathematics, 5 (Published for the Tata Institute of Fundamental Research, Bombay, 1970).Google Scholar
Mumford, D., ‘Theta characteristics of an algebraic curve’, Ann. Sci. Éc. Norm. Supèr. (4) 4 (1971), 181–192.Google Scholar
Mumford, David, Tata Lectures on Theta. II, Progress in Mathematics, 43 (Birkhäuser Boston Inc., Boston, MA, 1984), Jacobian theta functions and differential equations; with the collaboration of C. Musili, M. Nori, E. Previato, M. Stillman and H. Umemura.Google Scholar
Pauli, S. and Roblot, X.-F., ‘On the computation of all extensions of a p-adic field of a given degree’, Math. Comp. 70(236) (2001), 1641–1659. (electronic).Google Scholar
Poonen, B., ‘Bertini theorems over finite fields’, Ann. of Math. (2) 160(3) (2004), 1099–1127.Google Scholar
Poonen, B. and Schaefer, E. F., ‘Explicit descent for Jacobians of cyclic covers of the projective line’, J. Reine Angew. Math. 488 (1997), 141–188.Google Scholar
Poonen, B., Schaefer, E. F. and Stoll, M., ‘Twists of X (7) and primitive solutions to x 2 + y 3 = z 7 ’, Duke Math. J. 137(1) (2007), 103–158.Google Scholar
Poonen, B. and Stoll, M., ‘The Cassels-Tate pairing on polarized abelian varieties’, Ann. of Math. (2) 150(3) (1999), 1109–1149.Google Scholar
Salmon, G., Lessons Introductory to the Modern Higher Algebra, 3rd edn, (Hodges, Foster, and Co., Dublin, 1876).Google Scholar
Salmon, G., A Treatise on the Higher Plane Curves, 3rd edn, (Hodges, Foster, and Figgis, Dublin, 1879).Google Scholar
Schaefer, E. F., ‘2-descent on the Jacobians of hyperelliptic curves’, J. Number Theory 51(2) (1995), 219–232.Google Scholar
Schaefer, E. F., ‘Class groups and Selmer groups’, J. Number Theory 56(1) (1996), 79–114.Google Scholar
Schaefer, E. F., ‘Computing a Selmer group of a Jacobian using functions on the curve’, Math. Ann. 310(3) (1998), 447–471.Google Scholar
Schaefer, E. F. and Stoll, M., ‘How to do a p-descent on an elliptic curve’, Trans. Amer. Math. Soc. 356(3) (2004), 1209–1231, (electronic).Google Scholar
Serre, J.-P., Local Fields, Graduate Texts in Mathematics, 67 (Springer, New York, 1979), translated from the French by Marvin Jay Greenberg.Google Scholar
Stauduhar, R. P., ‘The determination of Galois groups’, Math. Comp. 27 (1973), 981–996.Google Scholar
Stoll, M., ‘Independence of rational points on twists of a given curve’, Compos. Math. 142(5) (2006), 1201–1214.CrossRefGoogle Scholar
Thorne, J. A., ‘The arithmetic of simple singularities’, PhD Thesis, Harvard University, April, 2012.Google Scholar
Weil, A., ‘L’arithmétique sur les courbes algébriques’, Acta Math. 52(1) (1929), 281–315.Google Scholar