Article contents
CANONICAL REPRESENTATIVES FOR DIVISOR CLASSES ON TROPICAL CURVES AND THE MATRIX–TREE THEOREM
Published online by Cambridge University Press: 26 September 2014
Abstract
Let 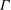 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\Gamma $ be a compact tropical curve (or metric graph) of genus
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\Gamma $ be a compact tropical curve (or metric graph) of genus  $g$. Using the theory of tropical theta functions, Mikhalkin and Zharkov proved that there is a canonical effective representative (called a break divisor) for each linear equivalence class of divisors of degree
$g$. Using the theory of tropical theta functions, Mikhalkin and Zharkov proved that there is a canonical effective representative (called a break divisor) for each linear equivalence class of divisors of degree 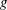 $g$ on
$g$ on 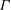 $\Gamma $. We present a new combinatorial proof of the fact that there is a unique break divisor in each equivalence class, establishing in the process an ‘integral’ version of this result which is of independent interest. As an application, we provide a ‘geometric proof’ of (a dual version of) Kirchhoff’s celebrated matrix–tree theorem. Indeed, we show that each weighted graph model
$\Gamma $. We present a new combinatorial proof of the fact that there is a unique break divisor in each equivalence class, establishing in the process an ‘integral’ version of this result which is of independent interest. As an application, we provide a ‘geometric proof’ of (a dual version of) Kirchhoff’s celebrated matrix–tree theorem. Indeed, we show that each weighted graph model 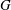 $G$ for
$G$ for 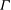 $\Gamma $ gives rise to a canonical polyhedral decomposition of the
$\Gamma $ gives rise to a canonical polyhedral decomposition of the  $g$-dimensional real torus
$g$-dimensional real torus  $\mathrm{Pic}^g(\Gamma )$ into parallelotopes
$\mathrm{Pic}^g(\Gamma )$ into parallelotopes 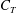 $C_T$, one for each spanning tree
$C_T$, one for each spanning tree 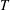 $T$ of
$T$ of 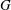 $G$, and the dual Kirchhoff theorem becomes the statement that the volume of
$G$, and the dual Kirchhoff theorem becomes the statement that the volume of 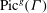 $\mathrm{Pic}^g(\Gamma )$ is the sum of the volumes of the cells in the decomposition.
$\mathrm{Pic}^g(\Gamma )$ is the sum of the volumes of the cells in the decomposition.
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/3.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author(s) 2014
References
- 34
- Cited by


