A generalized Danckwerts transformation

Abstract

Linear Fredholm operators (J, k) of the form u(x, t) = (J, k) v (x, t) ≡ J(t) v (x, t)+ 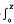k(t, s) v (x, s)ds can be found which map solutions v of the linear matrix system of n partial differential equations ∂u/∂t = DLv + Bv, into solutions u of the like system ∂u/∂t = DLu + Au, when the diagonal matrix D with positive elements and the matrices A and B commute with the linear, scalar operator L. For solution sets in appropriate function spaces, this mapping (J, k) is unique, independent of L, and 1–1 onto if it preserves initial values so that u(x, 0) = v(x, 0). When the set of solutions is restricted to those with zero initial values, this uniqueness aspect of (j, k) breaks down, and there are many linear maps of this Fredholm form which preserve the zero initial conditions and map all such solutions of the first equation in the appropriate function space into solutions of the second. When L is an unbounded operator like the Laplacian ∇², the initial value problems have many solutions depending on the values of u and u on the boundaries of the region of the solutions, as well as their values in the region at time t = 0. Danckwerts introduced the concept of a ‘constant preserving’ map of Volterra form mapping solutions of the scalar diffusion equation ∂u/∂t = D∇²u, which are initially zero, onto solutions of a scalar diffusion equation ∂u/∂t = D∇²u + Au, with a linear, homogeneous, constant coefficient source term. This concept of a ‘constant preserving’ map extends to the nth order matrix Fredholm maps described. A map (J, k∗) is said to be constant preserving if for all constant n x n matrices C, (J, k∗)C = C, and hence J(t) + 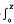k∗(t, s)ds = I, where I is the unit matrix. In the restricted solution spaces where u(x, 0) and v(x, 0) are zero, there is a unique 1–1 onto Danckwerts map of this type transforming solutions of the v equation into solutions of the u equation. In the cases where the coupling matrix A is constant and B is zero, the kernel k∗ of the Danckwerts map can be expressed in terms of the kernel k for the more general Fredholm map associated with the same equations, but mapping the larger solution sets containing elements which need not vanish at time zero. The Danckwerts mapping is used to establish a generalized Ussing flux-ratio theorem for some coupled diffusion problems involving several chemically interacting components.