Dynamic and stochastic propagation of the Brenier optimal mass transport

Abstract

Similar to how Hopf–Lax–Oleinik-type formula yield variational solutions for Hamilton–Jacobi equations on Euclidean space, optimal mass transportations can sometimes provide variational formulations for solutions of certain mean-field games. We investigate here the particular case of transports that maximize and minimize the following ‘ballistic’ cost functional on phase space TM, which propagates Brenier’s transport along a Lagrangian L,

$$b_T(v, x):=\inf\left\{\langle v, \gamma (0)\rangle +\int_0^TL(t, \gamma (t), {\dot \gamma}(t))\, dt; \gamma \in C^1([0, T], M); \gamma(T)=x\right\}\!,$$

where $M = \mathbb{R}^d$, and T >0. We also consider the stochastic counterpart:

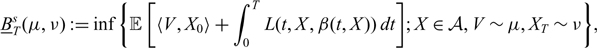\begin{align*} \underline{B}_T^s(\mu,\nu):=\inf\left\{\mathbb{E}\left[\langle V,X_0\rangle +\int_0^T L(t, X,\beta(t,X))\,dt\right]\!; X\in \mathcal{A}, V\sim\mu,X_T\sim \nu\right\}\!, \end{align*}

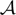where $\mathcal{A}$ is the set of stochastic processes satisfying dX = β_X (t, X) dt + dW_t, for some drift β_X (t, X), and where W_t is σ(X_s: 0 ≤ s ≤ t)-Brownian motion. Both cases lead to Lax–Oleinik-type formulas on Wasserstein space that relate optimal ballistic transports to those associated with dynamic fixed-end transports studied by Bernard–Buffoni and Fathi–Figalli in the deterministic case, and by Mikami–Thieullen in the stochastic setting. While inf-convolution easily covers cost minimizing transports, this is not the case for total cost maximizing transports, which actually are sup-inf problems. However, in the case where the Lagrangian L is jointly convex on phase space, Bolza-type dualities – well known in the deterministic case but novel in the stochastic case – transform sup-inf problems to sup–sup settings. We also write Eulerian formulations and point to links with the theory of mean-field games.