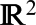Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-03T00:20:29.262Z Has data issue: false hasContentIssue false
  • English
  • Français

Refined stability thresholds for localized spot patterns for the Brusselator model in $\mathbb{R}^2$

Published online by Cambridge University Press:  30 July 2018

Y. CHANG ,
J. C. TZOU ,
M. J. WARD  and
J. C. WEI
Y. CHANG
Affiliation:
Department of Mathematics, University of Washington, Seattle, WA, USA email: [email protected]
J. C. TZOU
Affiliation:
Department of Mathematics, Macquarie University, Sydney, Australia email: [email protected]
M. J. WARD
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC, Canada email: [email protected], [email protected]
J. C. WEI
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC, Canada email: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the singular perturbation limit ε → 0, we analyse the linear stability of multi-spot patterns on a bounded 2-D domain, with Neumann boundary conditions, as well as periodic patterns of spots centred at the lattice points of a Bravais lattice in 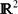$\mathbb{R}^2$, for the Brusselator reaction–diffusion model

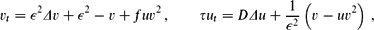$$ \begin{equation*} v_t = \epsilon^2 \Delta v + \epsilon^2 - v + fu v^2 \,, \qquad \tau u_t = D \Delta u + \frac{1}{\epsilon^2}\left(v - u v^2\right) \,, \end{equation*} $$
where the parameters satisfy 0 < f < 1, τ > 0 and D > 0. A previous leading-order linear stability theory characterizing the onset of spot amplitude instabilities for the parameter regime D = ${\mathcal O}$(ν−1), where ν = −1/log ϵ, based on a rigorous analysis of a non-local eigenvalue problem (NLEP), predicts that zero-eigenvalue crossings are degenerate. To unfold this degeneracy, the conventional leading-order-in-ν NLEP linear stability theory for spot amplitude instabilities is extended to one higher order in the logarithmic gauge ν. For a multi-spot pattern on a finite domain under a certain symmetry condition on the spot configuration, or for a periodic pattern of spots centred at the lattice points of a Bravais lattice in 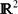$\mathbb{R}^2$, our extended NLEP theory provides explicit and improved analytical predictions for the critical value of the inhibitor diffusivity D at which a competition instability, due to a zero-eigenvalue crossing, will occur. Finally, when D is below the competition stability threshold, a different extension of conventional NLEP theory is used to determine an explicit scaling law, with anomalous dependence on ϵ, for the Hopf bifurcation threshold value of τ that characterizes temporal oscillations in the spot amplitudes.

Keywords

Spot patternsnonlocal eigenvalue problemHopf and competition stability thresholdsBloch Green's function
Type
Papers
Information
European Journal of Applied Mathematics , Volume 30 , Issue 4 , August 2019 , pp. 791 - 828
Copyright
Copyright © Cambridge University Press 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†Michael J. Ward and Juncheng Wei were supported by NSERC Discovery grants. Justin Tzou was partially supported by a PIMS CRG Postdoctoral Fellowship. Yifan Chang was supported by a graduate research stipend while at UBC.

References

[1] Abramowitz, M. & Stegun, I. (1965) Handbook of Mathematical Functions, 9th ed., Dover Publications, New York, NY.Google Scholar
[2] Astrov, Y. A. & Purwins, H. G. (2006) Spontaneous division of dissipative solitons in a planar gas-discharge system with high ohmic electrode. Phys. Lett. A 358 (5–6), 404–408.Google Scholar
[3] Avitabile, D., Brena-Medina, V. & Ward, M. J. (2018) Spot dynamics in a reaction-diffusion model of plant root hair initiation. SIAM J. Appl. Math. 78 (1), 291–319.Google Scholar
[4] Beylkin, G., Kurcz, C. & Monzón, L. (2008) Fast algorithms for Helmholtz Green's functions. Proc. Roy. Soc. A 464 (2100), 3301–3326.Google Scholar
[5] Callahan, T. K. & Knobloch, E. (1997) Symmetry-breaking bifurcations on cubic lattices. Nonlinearity 10 (5), 1179–1216.Google Scholar
[6] Callahan, T. K. & Knobloch, E. (2001) Long-wavelength instabilities of three dimensional patterns. Phys. Rev. E. 64 (3), 036214.Google Scholar
[7] Chen, W. & Ward, M. J. (2011) The stability and dynamics of localized spot patterns in the two-dimensional Gray–Scott model. SIAM J. Appl. Dyn. Sys. 10 (2), 582–666.Google Scholar
[8] Davis, P. W., Blanchedeau, P., Dullos, E. & De Kepper, P. (1998) Dividing blobs, chemical flowers, and patterned islands in a reaction–diffusion system. J. Phys. Chem. A 102 (43), 8236–8244.Google Scholar
[9] FlexPDE6. PDE Solutions Inc. URL: http://www.pdesolutions.com (last accessed May 2018).Google Scholar
[10] Iron, D., Ward, M. J. & Wei, J. (2001) The stability of spike solutions to the one-dimensional Gierer–Meinhardt model. Physica D 150 (1–2), 25–62.Google Scholar
[11] Iron, D., Rumsey, J., Ward, M. J. & Wei, J. (2014) Logarithmic expansions and the stability of periodic patterns of localized spots for reaction–diffusion systems. J. Nonlinear Sci. 24 (5), 857–912.Google Scholar
[12] Kolokolnikov, T., Ward, M. J. & Wei, J. (2009) Spot self-replication and dynamics for the Schnakenberg model in a two-dimensional domain. J. Nonlinear Sci. 19 (1), 1–56.Google Scholar
[13] Kolokolnikov, T., Titcombe, M. & Ward, M. J. (2005) Optimizing the fundamental Neumann eigenvalue for the Laplacian in a domain with small traps. Eur. J. Appl. Math. 16 (2), 161–200.Google Scholar
[14] Kolokonikov, T., Ward, M. J. & Wei, J. (2014) The stability of hot-spot patterns for a reaction–diffusion system of urban crime. DCDS-B 19 (5), 1373–1410.Google Scholar
[15] Knobloch, E. (2015) Spatial localization in dissipative systems. Ann. Rev. Cond. Mat. Phys. 6, 325–359.Google Scholar
[16] Kropinski, M. C. & Quaife, B. D. (2011) Fast integral equation methods for the modified Helmholtz equation. J. Comp. Phys. 230 (2), 425–434.Google Scholar
[17] Lee, K. J., McCormick, W. D., Pearson, J. E. & Swinney, H. L. (1994) Experimental observation of self-replicating spots in a reaction–diffusion system. Nature 369, 215–218.Google Scholar
[18] Muratov, C. & Osipov, V. V. (2001) Spike autosolitons and pattern formation scenarios in the two-dimensional Gray–Scott model. Eur. Phys. J. B 22 (2), 213–221.Google Scholar
[19] Muratov, C. & Osipov, V. V. (2002) Stability of static spike autosolitons in the Gray–Scott model. SIAM J. Appl. Math. 62 (5), 1463–1487.Google Scholar
[20] Nishiura, Y. (2002) Far-From Equilibrium Dynamics, Translations of Mathematical Monographs, Vol. 209, AMS Publications, Providence, Rhode Island.Google Scholar
[21] Pearson, J. E. (1993) Complex patterns in a simple system. Science 216, 189–192.Google Scholar
[22] Prigogine, I. & Lefever, R. (1968) Symmetry breaking instabilities in dissipative systems. II. J. Chem. Phys. 48, 1695.Google Scholar
[23] Rozada, I., Ruuth, S. & Ward, M. J. (2014) The stability of localized spot patterns for the Brusselator on the sphere. SIAM J. Appl. Dyn. Sys. 13 (1), 564–627.Google Scholar
[24] Sewalt, L. & Doelman, A. (2017) Spatially periodic multi-pulse patterns in a generalized Klausmeier–Gray–Scott model. SIAM J. Appl. Dyn. Sys. 16 (2), 1113–1163.Google Scholar
[25] Trinh, P. & Ward, M. J. (2016) The dynamics of localized spot patterns for reaction–diffusion systems on the sphere. Nonlinearity 29 (3), 766–806.Google Scholar
[26] Tzou, J., Xie, S., Kolokolnikov, T. & Ward, M. J. (2017) The stability and slow dynamics of localized spot patterns for the 3-D Schnakenberg reaction–diffusion model. SIAM J. Appl. Dyn. Sys. 16 (1), 294–336.Google Scholar
[27] Tzou, J. & Ward, M. J. (2018) The stability and slow dynamics of spot patterns in the 2D Brusselator model: The effect of open systems and heterogeneities. Physica D 373 (15 June 2018), 13–37.Google Scholar
[28] Tzou, J., Ward, M. J. & Wei, J. (2017) Anomalous scaling of Hopf bifurcation thresholds for the stability of localized spot patterns for reaction–diffusion systems in 2-D. SIAM J. Appl. Dyn. Sys. 17 (1), 982–1022.Google Scholar
[29] Turing, A. (1952) The chemical basis of morphogenesis. Phil. Trans. Roy. Soc. B 327, 37–72.Google Scholar
[30] Vanag, V. K. & Epstein, I. R. (2007) Localized patterns in reaction–diffusion systems. Chaos 17 (3), 037110.Google Scholar
[31] Ward, M. J. (2018) Spots, traps, and patches: Asymptotic analysis of localized solutions to some linear and nonlinear diffusive systems, invited survey article. Nonlinearity 31 (8), R189.Google Scholar
[32] Ward, M. J. & Wei, J. (2003) Hopf bifurcation and oscillatory instabilities of spike solutions for the one-dimensional Gierer–Meinhardt model. J. Nonlinear Sci. 13 (2), 209–264.Google Scholar
[33] Wei, J. & Winter, M. (2001) Spikes for the two-dimensional Gierer–Meinhardt system: The weak coupling case. J. Nonlinear Sci. 11 (6), 415–458.Google Scholar
[34] Wei, J. & Winter, M. (2003) Existence and stability of multiple spot solutions for the Gray–Scott model in $\mathbb{R}^2$. Physica D 176 (3–4), 147–180.Google Scholar
[35] Wei, J. & Winter, M. (2008) Stationary multiple spots for reaction–diffusion systems. J. Math. Biol. 57 (1), 53–89.Google Scholar
[36] Wei, J., (2008) Existence and stability of spikes for the Gierer–Meinhardt system. In: Chipot, M. (editor), Handbook of Differential Equations, Stationary Partial Differential Equations, Vol. 5, Elsevier, Amsterdam, pp. 489–581.Google Scholar
[37] Wei, J. & Winter, M. (2014) Mathematical Aspects of Pattern Formation in Biological Systems, Applied Mathematical Science Series, Vol. 189, Springer, London, Heidelberg, New York, Dordrecht.Google Scholar
[38] Xie, S. & Kolokolnikov, T. (2017) Moving and jumping spot in a two dimensional reaction–diffusion model. Nonlinearity 30 (4), 1536–1563.Google Scholar