Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-24T08:57:45.874Z Has data issue: false hasContentIssue false
  • English
  • Français

Local limit theorems for Brownian additive functionalsand penalisation of Brownian paths, IX

Published online by Cambridge University Press:  15 December 2008

Bernard Roynette  and
Marc Yor
Bernard Roynette
Affiliation:
Institut Elie Cartan, Université Henri Poincaré, BP 239, 54506 Vandoeuvre-lés-Nancy Cedex, France
Marc Yor
Affiliation:
Laboratoire de Probabilités et Modèles Aléatoires, Université Paris VI et VII, 4 place Jussieu, Case 188, 75252 Paris Cedex 05, France Institut Universitaire de France, Paris, France
Get access

Abstract

We obtain a local limit theorem for the laws of a class of Brownian additive functionals and we apply this result to a penalisation problem. We study precisely the case of the additive functional: $(A_t^{-}:= \int_0^t 1_{X_s < 0}{\rm d}s, t\geq 0)$ 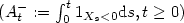. On the other hand, we describe Feynman-Kac type penalisation results for long Brownian bridges thus completing some similar previous study for standard Brownian motion (see [B. Roynette, P. Vallois and M. Yor, Studia Sci. Math. Hung.43 (2006) 171–246]).

Keywords

Limit theorems for additive functionalsFeynman-Kac functionalslong Brownian bridges.
Type
Research Article
Information
ESAIM: Probability and Statistics , Volume 14 , 2010 , pp. 65 - 92
Copyright
© EDP Sciences, SMAI, 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

W. Feller, An introduction to probability theory and its applications, volume II. John Wiley & Sons Inc., New York (1966).
Hariya, Y. and Yor, M., Limiting distributions associated with moments of exponential Brownian functionals. Studia Sci. Math. Hung. 41 (2004) 193–242.
T. Jeulin, Semimartingales et grossissement d'une filtration. Lect. Notes Maths 833. Springer (1980).
T. Jeulin and M. Yor, Eds., Grossissements de filtrations: exemples et applications. Lect. Notes Maths 1118. Springer (1985).
I. Karatzas and S. Shreve, Brownian motion and Stochastic Calculus. Springer (1991).
S. Kotani, Asymptotics for expectations of multiplicative functionals of 1-dimensional Brownian motion. Preprint (2006).
N.N. Lebedev, Special functions and their applications. Dover (1972).
R. Mansuy and M. Yor, Random Times and Enlargement of Filtrations in a Brownian Setting. Lect. Notes Maths 1873. Springer (2006).
J. Najnudel, B. Roynette and M. Yor, A global view of Brownian penalisations. MSJ Memoirs, volume 19. Mathematical Society of Japan, Tokyo (2009).
D. Revuz and M. Yor, Continuous Martingales and Brownian Motion. Third edition. Springer (1999).
B. Roynette and M. Yor, Penalising Brownian paths. Lect. Notes Maths 1969. Springer (2009).
Roynette, B., Vallois, P. and Yor, M., Penalisation of a Brownian motion with drift by a function of its one-sided maximum and its position, III. Periodica Math. Hung. 50 (2005) 247–280. CrossRef
Roynette, B., Vallois, P. and Yor, M., Some penalisations of the Wiener measure. Japan J. Math. 1 (2006) 263–299. CrossRef
Roynette, B., Vallois, P. and Yor, M., Limiting laws associated with Brownian motion perturbed by normalized exponential weights. Studia Sci. Math. Hung. 43 (2006) 171–246.
Roynette, B., Vallois, P. and Yor, M., Limiting laws associated with Brownian motions perturbed by its maximum, minimum, and local time, II. Studia Sci. Math. Hung. 43 (2006) 295–360.
Yor, M., The distribution of Brownian quantiles. J. Appl. Prob. 32 (1995) 405–416. CrossRef