Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-30T21:17:54.034Z Has data issue: false hasContentIssue false
  • English
  • Français

Distortion mismatch in the quantization of probability measures

Published online by Cambridge University Press:  23 January 2008

Siegfried Graf ,
Harald Luschgy  and
Gilles Pagès
Siegfried Graf
Affiliation:
Universität Passau, Fakultät für Informatik und Mathematik, 94030 Passau, Germany; [email protected]
Harald Luschgy
Affiliation:
Universität Trier, FB IV-Mathematik, 54286 Trier, Germany; [email protected]
Gilles Pagès
Affiliation:
Laboratoire de Probabilités et Modèles aléatoires, UMR 7599, Université Paris 6, case 188, 4, pl. Jussieu, 75252 Paris cedex 5, France; [email protected]
Get access

Abstract

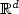We elucidate the asymptotics of the Ls -quantization error induced by a sequence of Lr -optimal n-quantizers of aprobability distribution P on $\mathbb{R}^d$ when s > r. In particular we show that under natural assumptions, the optimal rate is preserved aslong as s < r+d (and for everys in the case of a compactly supported distribution). We derive some applications of these results to the error bounds for quantization based cubatureformulae in numerical integration on $\mathbb{R}^d$ 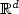and on the Wiener space.

Keywords

Optimal quantizationZador Theorem
Type
Research Article
Information
ESAIM: Probability and Statistics , Volume 12 , 2008 , pp. 127 - 153
Copyright
© EDP Sciences, SMAI, 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bally, V. and Pagès, C., A quantization algorithm for solving discrete time multidimensional optimal stopping problems. Bernoulli 9 (2003) 1003–1049. CrossRef
Bally, V., Pagès, C. and Printems, J., First order schemes in the numerical quantization method. Mathematical Finance 13 (2001) 1–16. CrossRef
J.A. Bucklew and G.L. Wise, Multidimensional asymptiotic quantization theory with r-th power distortion measure. IEEE Trans. Inform. Theory, 28, Special issue on quantization, A. Gersho & R.M. Grey Eds., (1982) 239–247.
Delattre, S., Graf, S., Luschgy, H. and Pagès, G., Quantization of probability distributions under norm-based distortion measures. Statist. Decisions 22 (2004) 261–282. CrossRef
Delattre, S., Fort, J.C. and Pagès, G., Local distortion and µ-mass of the cells of one dimensional asymptotically optimal quantizers. Comm. Statist. Theory Methods 33 (2004) 1087–1117. CrossRef
S. Graf and H. Luschgy, Foundations of Quantization for Probability Distributions. Lect. Notes in Math. 1730, Springer, Berlin (2000).
Graf, S. and Luschgy, H., Rates of convergence for the empirical quantization error. Ann. Probab. 30 (2002) 874–897.
Luschgy, H. and Pagès, G., Functional quantization of stochastic processes. J. Funct. Anal. 196 (2002) 486–531. CrossRef
Luschgy, H. and Pagès, G., Sharp asymptotics of the functional quantization problem for Gaussian processes. Ann. Probab. 32 (2004) 1574–1599.
P. Mattila, Geometry of Sets and Measures in Euclidean Spaces. Cambridge University Press (1995).
Pagès, G., A space vector quantization method for numerical integration. J. Comput. Appl. Math. 89 (1997) 1–38. CrossRef
Pagès, G. and Printems, J., Functional quantization for numerics with an application to option pricing. Monte Carlo Methods & Applications 11 (2005) 407–446. CrossRef
A. Sellami, Quantization based filtering method using first order approximation. Pré-pub. LPMA-1009 (2005). To appear in SIAM J. Numerical Analysis.
P.L. Zador, Development and evaluation of procedures for quantizing multivariate distributions. Ph.D. thesis, Stanford University (1963).
P.L. Zador, Asymptotic quantization error of continuous signals and the quantization dimension. IEEE Trans. Inform. Theory 28, Special issue on quantization, A. Gersho & R.M. Grey Eds. (1982) 139–149.