Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-30T23:18:56.026Z Has data issue: false hasContentIssue false
  • English
  • Français

Directed polymer in random environment and last passage percolation*

Published online by Cambridge University Press:  29 October 2010

Philippe Carmona
Philippe Carmona*
Affiliation:
Laboratoire Jean Leray, UMR 6629 Université de Nantes, BP 92208, 44322 Nantes Cedex 03, France
*
Corresponding author: [email protected]
Get access

Abstract

The sequence of random probability measures ν n that gives a path of length n, $\frac{1}{n}$ 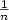times the sum of the random weights collected along the paths, is shown to satisfy a large deviations principle with good rate function the Legendre transform of the free energy of the associated directed polymer in a random environment.Consequences on the asymptotics of the typical number of paths whose collected weight is above a fixed proportion are then drawn.

Keywords

Directed polymerrandom environmentpartition functionlast passage percolation
Type
Research Article
Information
ESAIM: Probability and Statistics , Volume 14 , 2010 , pp. 263 - 270
Copyright
© EDP Sciences, SMAI, 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

*

The author acknowledges the support of the French Ministry of Education through the ANR BLAN07-2184264 grant.

References

Alexander, K.S., Approximation of subadditive functions and convergence rates in limiting-shape results. Ann. Probab. 25 (1997) 30–55. MR MR1428498.
C. Ané, S. Blachère, D. Chafaï, P. Fougères, I. Gentil, F. Malrieu, C. Roberto and G. Scheffer, Sur les inégalités de Sobolev logarithmiques, Panoramas et Synthèses [Panoramas and Syntheses], volume 10. Société Mathématique de France, Paris (2000). With a preface by Dominique Bakry and Michel Ledoux. MR MR1845806.
Bolthausen, E., A note on the diffusion of directed polymers in a random environment. Commun. Math. Phys. 123 (1989) 529–534. CrossRef
P. Carmona and Y. Hu, On the partition function of a directed polymer in a Gaussian random environment, Probab. Theory Relat. Fields 124 (2002) 431–457. MR MR1939654.
Carmona, P. and Fluctuation, Y. Hu exponents and large deviations for directed polymers in a random environment. Stoch. Process. Appl. 112 (2004) 285–308. CrossRef
Carmona, P. and Strong di, Y. Husorder implies strong localization for directed polymers in a random environment. ALEA 2 (2006) 217–229.
Comets, F. and Yoshida, N., Directed polymers in random environment are diffusive at weak disorder. Ann. Probab. 34 (2006) 1746–1770. CrossRef
Comets, F. and Vargas, V., Majorizing multiplicative cascades for directed polymers in random media. ALEA Lat. Am. J. Probab. Math. Stat. 2 (2006) 267–277 (electronic). MR MR2249671.
Comets, F., Popov, S. and Vachkovskaia, M., The number of open paths in an oriented $\rho$ -percolation model. J. Stat. Phys. 131 (2008) 357–379. MR MR2386584. CrossRef
A. Dembo and O. Zeitouni, Large deviations techniques and applications. Second edition. Volume 38 of Applications of Mathematics (New York). Springer-Verlag, New York (1998). MR MR1619036.
J. Feng and T.G. Kurtz, Large deviations for stochastic processes, in Mathematical Surveys and Monographs, volume 131. American Mathematical Society, Providence, RI (2006). MR MR2260560.
H. Kesten and V. Sidoravivius, A problem in last-passage percolation, preprint (2007), http://arxiv.org/abs/0706.3626.
Varadhan, S.R.S., Large deviations for random walks in a random environment. Commun. Pure Appl. Math. 56 (2003) 1222–1245. Dedicated to the memory of Jürgen K. Moser. MR MR1989232. CrossRef