Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-15T01:30:30.698Z Has data issue: false hasContentIssue false
  • English
  • Français

Finite element approximation of kinetic dilutepolymer models with microscopic cut-off

Published online by Cambridge University Press:  15 April 2010

John W. Barrett  and
Endre Süli
John W. Barrett
Affiliation:
Dept. of Mathematics, Imperial College London, London SW7 2AZ, UK. [email protected]
Endre Süli
Affiliation:
Mathematical Institute, University of Oxford, 24–29 St Giles', Oxford OX1 3LB, UK. [email protected]
Get access

Abstract

We construct a Galerkin finite element method for the numerical approximation of weak solutions to a coupled microscopic-macroscopic bead-spring model that arises from the kinetic theory of dilute solutionsof polymeric liquids with noninteracting polymer chains. The model consists of the unsteady incompressible Navier–Stokes equations in a bounded domain Ω ⊂ $\mathbb{R}^d$ , d = 2 or 3, for the velocity andthe pressure of the fluid, with an elastic extra-stress tensor as right-hand side in the momentum equation. The extra-stress tensor stems from the random movement of the polymer chains and is defined through the associated probability density function that satisfies a Fokker–Planck type parabolic equation, crucial features of which are the presence of a centre-of-mass diffusion term and a cut-off function $\beta^L(\cdot) :=\min(\cdot,L)$ 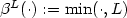in the drag and convective terms, where L ≫ 1. We focus on finitely-extensible nonlinear elastic, FENE-type, dumbbell models. We perform a rigorous passage to the limit as the spatial and temporal discretization parameters tend to zero, and show that a (sub)sequence of these finite element approximations converges to a weak solution of this coupled Navier–Stokes–Fokker–Planck system. The passage to the limit is performed under minimal regularity assumptions on the data. Our arguments therefore also provide a new proof of global existence of weak solutions to Fokker–Planck–Navier–Stokes systems with centre-of-mass diffusion and microscopic cut-off. The convergence proof rests on several auxiliary technical results including the stability, in the Maxwellian-weighted H 1 norm, of the orthogonal projector in the Maxwellian-weighted L 2 inner product onto finite element spaces consisting of continuous piecewise linear functions.We establish optimal-order quasi-interpolation error bounds in the Maxwellian-weighted L 2 and H 1 norms,and prove a new elliptic regularity result in the Maxwellian-weighted H 2 norm.

Keywords

Finite element methodpolymeric flow modelsconvergence analysisexistence of weak solutionsNavier–Stokes equationsFokker–Planck equationsFENE
Type
Research Article
Information
ESAIM: Mathematical Modelling and Numerical Analysis , Volume 45 , Issue 1 , January 2011 , pp. 39 - 89
Copyright
© EDP Sciences, SMAI, 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antoci, F., Some necessary and some sufficient conditions for the compactness of the embedding of weighted Sobolev spaces. Ric. Mat. 52 (2003) 55–71.
Arnold, A., Markowich, P., Toscani, G. and Unterreiter, A., On convex Sobolev inequalities and the rate of convergence to equilibrium for Fokker–Planck equations. Comm. PDE 26 (2001) 43–100. CrossRef
Barrett, J.W. and Nürnberg, R., Convergence of a finite-element approximation of surfactant spreading on a thin film in the presence of van der Waals forces. IMA J. Numer. Anal. 24 (2004) 323–363. CrossRef
Barrett, J.W. and Süli, E., Existence of global weak solutions to some regularized kinetic models of dilute polymers. Multiscale Model. Simul. 6 (2007) 506–546. CrossRef
Barrett, J.W. and Süli, E., Existence of global weak solutions to dumbbell models for dilute polymers with microscopic cut-off. Math. Models Methods Appl. Sci. 18 (2008) 935–971. CrossRef
Barrett, J.W. and Süli, E., Numerical approximation of corotational dumbbell models for dilute polymers. IMA J. Numer. Anal. 29 (2009) 937–959. CrossRef
Barrett, J.W., Schwab, C. and Süli, E., Existence of global weak solutions for some polymeric flow models. Math. Models Methods Appl. Sci. 15 (2005) 939–983. CrossRef
R. Bird, C. Curtiss, R. Armstrong and O. Hassager, Dynamics of Polymeric Liquids, Vol. 2: Kinetic Theory. John Wiley and Sons, New York (1987).
Bobkov, S. and Ledoux, M., From Brunn–Minkowski to Brascamp–Lieb and to logarithmic Sobolev inequalities. Geom. Funct. Anal. 10 (2000) 1028–1052. CrossRef
Brandts, J., Korotov, S., Křížek, M. and Šolc, J., On nonobtuse simplicial partitions. SIAM Rev. 51 (2009) 317–335. CrossRef
F. Brezzi and M. Fortin, Mixed and Hybrid Finite Element Methods. Springer-Verlag, Berlin (1991).
S. Cerrai, Second-order PDEs in Finite and Infinite Dimension, Lecture Notes in Mathematics 1762. Springer-Verlag, Berlin (2001).
P. Ciarlet, The Finite Element Method for Elliptic Problems. North-Holland, Amsterdam (1978).
Constantin, P., Nonlinear Fokker–Planck Navier–Stokes systems. Commun. Math. Sci. 3 (2005) 531–544. CrossRef
Da Prato, G. and Lunardi, A., Elliptic operators with unbounded drift coefficients and Neumann boundary condition. J. Differ. Equ. 198 (2004) 35–52. CrossRef
Desvillettes, L. and Villani, C., On the trend to global equilibrium in spatially inhomogeneous entropy-dissipating systems: the linear Fokker–Planck equation. Comm. Pure Appl. Math. 54 (2001) 1–42. 3.0.CO;2-Q>CrossRef
Du, Q., Liu, C. and FENE du, P. Yumbbell models and its several linear and nonlinear closure approximations. Multiscale Model. Simul. 4 (2005) 709–731. CrossRef
E, W., Li, T.J. and Zhang, P.-W., Well-posedness for the dumbbell model of polymeric fluids. Com. Math. Phys. 248 (2004) 409–427. CrossRef
El-Kareh, A.W. and Leal, L.G., Existence of solutions for all Deborah numbers for a non-Newtonian model modified to include diffusion. J. Non-Newton. Fluid Mech. 33 (1989) 257–287. CrossRef
Eppstein, D., Sullivan, J.M. and Üngör, A., Tiling space and slabs with acute tetrahedra. Comput. Geom. 27 (2004) 237–255. CrossRef
Grün, G. and Rumpf, M., Nonnegativity preserving numerical schemes for the thin film equation. Numer. Math. 87 (2000) 113–152.
Heywood, J.G. and Rannacher, R., Finite element approximation of the nonstationary Navier–Stokes problem. I: Regularity of solutions and second-order error estimates for spatial discretization. SIAM J. Numer. Anal. 19 (1982) 275–311. CrossRef
Itoh, J.-I. and Zamfirescu, T., Acute triangulations of the regular dodecahedral surface. European J. Combin. 28 (2007) 1072–1086. CrossRef
Jourdain, B., Lelièvre, T. and Le Bris, C., Existence of solution for a micro-macro model of polymeric fluid: the FENE model. J. Funct. Anal. 209 (2004) 162–193. CrossRef
Jourdain, B., Lelièvre, T., Le Bris, C. and Otto, F., Long-time asymptotics of a multiscle model for polymeric fluid flows. Arch. Rat. Mech. Anal. 181 (2006) 97–148. CrossRef
Knezevic, D. and Süli, E., Spectral Galerkin approximation of Fokker–Planck equations with unbounded drift. ESAIM: M2AN 43 (2009) 445–485. CrossRef
Knezevic, D. and Süli, E., A heterogeneous alternating-direction method for a micro-macro dilute polymeric fluid model. ESAIM: M2AN 43 (2009) 1117–1156. CrossRef
Korotov, S. and Křížek, M., Acute type refinements of tetrahedral partitions of polyhedral domains. SIAM J. Numer. Anal. 39 (2001) 724–733. CrossRef
Korotov, S. and Křížek, M., Global and local refinement techniques yielding nonobtuse tetrahedral partitions. Comput. Math. Appl. 50 (2005) 1105–1113.
A. Kufner, Weighted Sobolev Spaces. Teubner, Stuttgart (1980).
T. Lelièvre, Modèles multi-échelles pour les fluides viscoélastiques. Ph.D. Thesis, École National des Ponts et Chaussées, Marne-la-Vallée, France (2004).
Li, T. and Zhang, P.-W., Mathematical analysis of multi-scale models of complex fluids. Commun. Math. Sci. 5 (2007) 1–51. CrossRef
Li, T., Zhang, H. and Zhang, P.-W., Local existence for the dumbbell model of polymeric fuids. Comm. Partial Differ. Equ. 29 (2004) 903–923. CrossRef
Lin, F.-H., Liu, C. and Zhang, P., On a micro-macro model for polymeric fluids near equilibrium. Comm. Pure Appl. Math. 60 (2007) 838–866. CrossRef
Lions, P.-L. and Masmoudi, N., Global existence of weak solutions to some micro-macro models. C. R. Math. Acad. Sci. Paris 345 (2007) 15–20. CrossRef
L. Lorenzi and M. Bertoldi, Analytical Methods for Markov Semigroups. Chapman & Hall/CRC, Boca Raton (2007).
Lozinski, A., Chauvière, C., Fang, J. and Owens, R.G., Fokker–Planck simulations of fast flows of melts and concentrated polymer solutions in complex geometries. J. Rheol. 47 (2003) 535–561. CrossRef
Lozinski, A., Owens, R.G. and Fang, J., Fokker–Planck-based, A numerical method for modelling non-homogeneous flows of dilute polymeric solutions. J. Non-Newton. Fluid Mech. 122 (2004) 273–286. CrossRef
Masmoudi, N., Well posedness of the FENE dumbbell model of polymeric flows. Comm. Pure Appl. Math. 61 (2008) 1685–1714. CrossRef
Otto, F. and Tzavaras, A., Continuity of velocity gradients in suspensions of rod-like molecules. Comm. Math. Phys. 277 (2008) 729–758. CrossRef
Renardy, M., An existence theorem for model equations resulting from kinetic theories of polymer solutions. SIAM J. Math. Anal. 22 (1991) 1549–151.
Schieber, J.D., Generalized Brownian configuration field for Fokker–Planck equations including center-of-mass diffusion. J. Non-Newton. Fluid Mech. 135 (2006) 179–181. CrossRef
W.H.A. Schilders and E.J.W. ter Maten, Eds., Numerical Methods in Electromagnetics, Handbook of Numerical Analysis XIII. Amsterdam, North-Holland (2005).
J. Simon, Compact sets in the space L p(0,T;B). Ann. Math. Pur. Appl. 146 (1987) 65–96. CrossRef
R. Temam, Navier–Stokes Equations – Theory and Numerical Analysis, Studies in Mathematics and its Applications 2. Third Edition, Amsterdam, North-Holland (1984).
H. Triebel, Interpolation Theory, Function Spaces, Differential Operators. Second Edition, Johann Ambrosius Barth Publ., Heidelberg/Leipzig (1995).
Yu, P., Du, Q. and Liu, C., From micro to macro dynamics via a new closure approximation to the FENE model of polymeric fluids. Multiscale Model. Simul. 3 (2005) 895–917. CrossRef