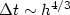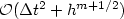Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kemm, Friedemann
2006.
Magnetische Ladungen.
Mathematische Semesterberichte,
Vol. 53,
Issue. 2,
p.
137.
Fuchs, Franz G.
Karlsen, Kenneth H.
Mishra, Siddharta
and
Risebro, Nils H.
2009.
Stable upwind schemes for the magnetic induction equation.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 43,
Issue. 5,
p.
825.
Koley, Ujjwal
Mishra, Siddhartha
Risebro, Nils Henrik
and
Svärd, Magnus
2009.
Higher order finite difference schemes for the magnetic induction equations.
BIT Numerical Mathematics,
Vol. 49,
Issue. 2,
p.
375.
Fuchs, F.G.
Mishra, S.
and
Risebro, N.H.
2009.
Splitting based finite volume schemes for ideal MHD equations.
Journal of Computational Physics,
Vol. 228,
Issue. 3,
p.
641.
Mishra, Siddhartha
and
Svärd, Magnus
2010.
On stability of numerical schemes via frozen coefficients and the magnetic induction equations.
BIT Numerical Mathematics,
Vol. 50,
Issue. 1,
p.
85.
Kemm, Friedemann
2013.
On the origin of divergence errors in MHD simulations and consequences for numerical schemes.
Communications in Applied Mathematics and Computational Science,
Vol. 8,
Issue. 1,
p.
1.
Betancourt, Fernando
and
Rohde, Christian
2016.
Finite-volume schemes for Friedrichs systems with involutions.
Applied Mathematics and Computation,
Vol. 272,
Issue. ,
p.
420.
Yang, He
and
Li, Fengyan
2016.
Stability analysis and error estimates of an exactly divergence-free method for the magnetic induction equations.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 50,
Issue. 4,
p.
965.
Kemm, Friedemann
2016.
Roe-type schemes for shallow water magnetohydrodynamics with hyperbolic divergence cleaning.
Applied Mathematics and Computation,
Vol. 272,
Issue. ,
p.
385.
Sarkar, Tanmay
2017.
Interior penalty discontinuous Galerkin method for magnetic induction equation with resistivity.
Applied Mathematics and Computation,
Vol. 314,
Issue. ,
p.
212.
Kemm, Friedemann
2018.
Facetten der Mathematik.
p.
219.
Chandrashekar, Praveen
2019.
A Global Divergence Conforming DG Method for Hyperbolic Conservation Laws with Divergence Constraint.
Journal of Scientific Computing,
Vol. 79,
Issue. 1,
p.
79.
Sarkar, Tanmay
and
Chandrashekar, Praveen
2019.
Stabilized discontinuous Galerkin scheme for the magnetic induction equation.
Applied Numerical Mathematics,
Vol. 137,
Issue. ,
p.
116.
Sarkar, Tanmay
2020.
A Priori Error Analysis of a Discontinuous Galerkin Scheme for the Magnetic Induction Equation.
Computational Methods in Applied Mathematics,
Vol. 20,
Issue. 1,
p.
121.
Mabuza, Sibusiso
Shadid, John N.
Cyr, Eric C.
Pawlowski, Roger P.
and
Kuzmin, Dmitri
2020.
A linearity preserving nodal variation limiting algorithm for continuous Galerkin discretization of ideal MHD equations.
Journal of Computational Physics,
Vol. 410,
Issue. ,
p.
109390.
Sarkar, Tanmay
2023.
Optimal error estimates of an IPDG scheme for the resistive magnetic induction equation.
Partial Differential Equations and Applications,
Vol. 4,
Issue. 4,
Dao, Tuan Anh
Nazarov, Murtazo
and
Tomas, Ignacio
2024.
A structure preserving numerical method for the ideal compressible MHD system.
Journal of Computational Physics,
Vol. 508,
Issue. ,
p.
113009.