Tracking with prescribed transient behaviour

Abstract

Universal tracking control is investigated in the context of a class S of M-input, M-output dynamical systems modelled by functional differential equations. The class encompasses a wide variety of nonlinear and infinite-dimensional systems and contains – as a prototype subclass – all finite-dimensional linear single-input single-output minimum-phase systems with positive high-frequency gain. 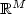The control objective is to ensure that, for an arbitrary $\mathbb{R}^M$-valued reference signal r of class W^1,∞ (absolutely continuous and bounded with essentially bounded derivative) and every system of class S, the tracking error e between plant output and reference signal evolves within a prespecified performance envelope or funnel in the sense that ${\varphi}(t)\| e(t)\| < 1$ for all t ≥ 0, where φ a prescribed real-valued function of class W^1,∞ with the property that φ(s) > 0 for all s > 0 and $\liminf_{s\rightarrow\infty}{\varphi}(s)>0$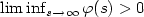. 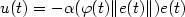A simple (neither adaptive nor dynamic) error feedback control of the form $u(t)=- \alpha ({\varphi}(t)\|e(t)\|)e(t)$ is introduced which achieves the objective whilst maintaining boundedness of the control and of the scalar gain $\alpha ({\varphi}(\cdot )\|e(\cdot )\|)$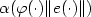.