Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Neff, P.
Fischle, A.
and
Münch, I.
2008.
Symmetric Cauchy stresses do not imply symmetric Biot strains in weak formulations of isotropic hyperelasticity with rotational degrees of freedom.
Acta Mechanica,
Vol. 197,
Issue. 1-2,
p.
19.
Neff, Patrizio
2008.
IUTAM Symposium on Theoretical, Computational and Modelling Aspects of Inelastic Media.
Vol. 11,
Issue. ,
p.
129.
NEFF, PATRIZIO
CHEŁMIŃSKI, KRZYSZTOF
and
ALBER, HANS-DIETER
2009.
NOTES ON STRAIN GRADIENT PLASTICITY: FINITE STRAIN COVARIANT MODELLING AND GLOBAL EXISTENCE IN THE INFINITESIMAL RATE-INDEPENDENT CASE.
Mathematical Models and Methods in Applied Sciences,
Vol. 19,
Issue. 02,
p.
307.
Neff, Patrizio
and
Münch, Ingo
2009.
Simple shear in nonlinear Cosserat elasticity: bifurcation and induced microstructure.
Continuum Mechanics and Thermodynamics,
Vol. 21,
Issue. 3,
p.
195.
Neff, P.
and
Jeong, J.
2009.
A new paradigm: the linear isotropic Cosserat model with conformally invariant curvature energy.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 89,
Issue. 2,
p.
107.
Neff, Patrizio
Jeong, Jena
and
Ramézani, Hamidréza
2009.
Subgrid interaction and micro-randomness – Novel invariance requirements in infinitesimal gradient elasticity.
International Journal of Solids and Structures,
Vol. 46,
Issue. 25-26,
p.
4261.
Neff, Patrizio
2010.
Poly-, Quasi- and Rank-One Convexity in Applied Mechanics.
Vol. 516,
Issue. ,
p.
301.
Tambača, Josip
and
Velčić, Igor
2010.
Semicontinuity theorem in the micropolar elasticity.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 16,
Issue. 2,
p.
337.
Velčić, Igor
and
Tambača, Josip
2010.
Relaxation Theorem and Lower-Dimensional Models in Micropolar Elasticity.
Mathematics and Mechanics of Solids,
Vol. 15,
Issue. 8,
p.
812.
Tambača, Josip
and
Velčić, Igor
2010.
Existence theorem for nonlinear micropolar elasticity.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 16,
Issue. 1,
p.
92.
Jeong, Jena
and
Ramézani, Hamidréza
2010.
Enhanced numerical study of infinitesimal non-linear Cosserat theory based on the grain size length scale assumption.
Computer Methods in Applied Mechanics and Engineering,
Vol. 199,
Issue. 45-48,
p.
2892.
Münch, I.
Neff, P.
and
Wagner, W.
2011.
Transversely isotropic material: nonlinear Cosserat versus classical approach.
Continuum Mechanics and Thermodynamics,
Vol. 23,
Issue. 1,
p.
27.
Neff, Patrizio
Pauly, Dirk
and
Witsch, Karl‐Josef
2012.
Maxwell meets Korn: A new coercive inequality for tensor fields in with square‐integrable exterior derivative.
Mathematical Methods in the Applied Sciences,
Vol. 35,
Issue. 1,
p.
65.
Lankeit, Johannes
Neff, Patrizio
and
Pauly, Dirk
2013.
Uniqueness of Integrable Solutions ∇ζ = Gζ, ζ∣Γ = 0 for Integrable Tensor‐Coefficients G and Applications to Elasticity.
PAMM,
Vol. 13,
Issue. 1,
p.
361.
Lankeit, Johannes
Neff, Patrizio
and
Pauly, Dirk
2013.
Uniqueness of integrable solutions to $${\nabla \zeta=G \zeta, \zeta|_\Gamma = 0}$$ for integrable tensor coefficients G and applications to elasticity.
Zeitschrift für angewandte Mathematik und Physik,
Vol. 64,
Issue. 6,
p.
1679.
Lankeit, Johannes
Neff, Patrizio
and
Pauly, Dirk
2013.
Unique continuation for first-order systems with integrable coefficients and applications to elasticity and plasticity.
Comptes Rendus. Mathématique,
Vol. 351,
Issue. 5-6,
p.
247.
Del Vescovo, Dionisio
and
Giorgio, Ivan
2014.
Dynamic problems for metamaterials: Review of existing models and ideas for further research.
International Journal of Engineering Science,
Vol. 80,
Issue. ,
p.
153.
Bîrsan, Mircea
and
Neff, Patrizio
2014.
Existence of minimizers in the geometrically non-linear 6-parameter resultant shell theory with drilling rotations.
Mathematics and Mechanics of Solids,
Vol. 19,
Issue. 4,
p.
376.
Neff, Patrizio
Nakatsukasa, Yuji
and
Fischle, Andreas
2014.
A Logarithmic Minimization Property of the Unitary Polar Factor in the Spectral and Frobenius Norms.
SIAM Journal on Matrix Analysis and Applications,
Vol. 35,
Issue. 3,
p.
1132.
Neff, Patrizio
Ghiba, Ionel-Dumitrel
Madeo, Angela
Placidi, Luca
and
Rosi, Giuseppe
2014.
A unifying perspective: the relaxed linear micromorphic continuum.
Continuum Mechanics and Thermodynamics,
Vol. 26,
Issue. 5,
p.
639.
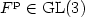 be the plastic deformation from the multiplicative decomposition in elasto-plasticity. We show that the geometric dislocation density tensor of Gurtin in the form ${\rm Curl}[{F^{\rm p}}]\cdot (F^{\rm p})^T$
be the plastic deformation from the multiplicative decomposition in elasto-plasticity. We show that the geometric dislocation density tensor of Gurtin in the form ${\rm Curl}[{F^{\rm p}}]\cdot (F^{\rm p})^T$ 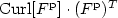 applied to rotations controls the gradient in the sense that pointwise $ \forall R \in C^1(\mathbb{R}^3, {\rm SO}(3)): \Arrowvert {\rm Curl}[R] \cdot R^T \Arrowvert_{\mathbb{M}^{3\times3}}^2 \ge \frac{1}{2} \Arrowvert{\rm D}R\Arrowvert_{\mathbb{R}^{27}}^2$
applied to rotations controls the gradient in the sense that pointwise $ \forall R \in C^1(\mathbb{R}^3, {\rm SO}(3)): \Arrowvert {\rm Curl}[R] \cdot R^T \Arrowvert_{\mathbb{M}^{3\times3}}^2 \ge \frac{1}{2} \Arrowvert{\rm D}R\Arrowvert_{\mathbb{R}^{27}}^2$ 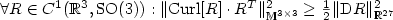 .This result complements rigidity results[Friesecke, James and Müller, Comme Pure Appl. Math.55 (2002) 1461–1506; John, Comme Pure Appl. Math.14 (1961) 391–413; Reshetnyak, Siberian Math. J.8 (1967) 631–653)] as well as an associated linearized theorem saying that $ \forall A \in C^1(\mathbb{R}^3, \mathfrak{so}(3)): \Arrowvert {\rm Curl}[A]\Arrowvert_{\mathbb{M}^{3\times3}}^2 \ge \frac{1}{2} \Arrowvert{\rm D}A\Arrowvert_{\mathbb{R}^{27}}^2 = \Arrowvert\nabla{\rm axl}[A]\Arrowvert_{\mathbb{R}^9}^2$
.This result complements rigidity results[Friesecke, James and Müller, Comme Pure Appl. Math.55 (2002) 1461–1506; John, Comme Pure Appl. Math.14 (1961) 391–413; Reshetnyak, Siberian Math. J.8 (1967) 631–653)] as well as an associated linearized theorem saying that $ \forall A \in C^1(\mathbb{R}^3, \mathfrak{so}(3)): \Arrowvert {\rm Curl}[A]\Arrowvert_{\mathbb{M}^{3\times3}}^2 \ge \frac{1}{2} \Arrowvert{\rm D}A\Arrowvert_{\mathbb{R}^{27}}^2 = \Arrowvert\nabla{\rm axl}[A]\Arrowvert_{\mathbb{R}^9}^2$ 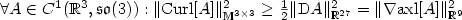 .
.