Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-29T01:07:02.760Z Has data issue: false hasContentIssue false
  • English
  • Français

Weighted Birkhoff ergodic theorem with oscillating weights

Published online by Cambridge University Press:  25 September 2017

AI-HUA FAN
AI-HUA FAN*
Affiliation:
School of Mathematics and Statistics, Central China Normal University, 430079, Wuhan, China LAMFA, UMR 7352 CNRS, University of Picardie, 33 rue Saint Leu, 80039 Amiens, France email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider sequences of Davenport type or Gelfond type and prove that sequences of Davenport exponent larger than 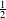$\frac{1}{2}$ are good sequences of weights for the ergodic theorem, and that the ergodic sums weighted by a sequence of strong Gelfond property are well controlled almost everywhere. We prove that for any $q$-multiplicative sequence, the Gelfond property implies the strong Gelfond property and that sequences realized by dynamical systems can be fully oscillating and have the Gelfond property.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 5 , May 2019 , pp. 1275 - 1289
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

El Abdalaoui, H., Kulaga-Przymus, J., Lemanczyk, M. and de la Rue, T.. The Chowla and the Sarnak conjectures from ergodic theory point of view. Discrete Contin. Dyn. Syst. 37(6) (2017), 2899–2944.Google Scholar
Abramov, L. M.. Metric automorphisms with quasi-discrete spectrum. Izv. Akad. Nauk 26 (1962), 513–530.Google Scholar
Baladi, V.. Positive Transfer Operators and Decay Of Correlations (Advanced Series in Nonlinear Dynamics, 16) . World Scientific, Singapore, 2000.Google Scholar
Bourgain, J.. Pointwise ergodic theorem for arithmetic sets. Publ. Math. Inst. Hautes Études Sci. 69 (1989), 5–41.Google Scholar
Cohen, G. and Lin, M.. Extensions of the Menchoff–Rademacher theorem with applications to ergodic theory. Israel J. Math. 148(1) (2005), 41–86.Google Scholar
Cuny, C.. On the a.s. convergence of the one-sided ergodic Hilbert transform. Ergod. Th. & Dynam. Sys. 29 (2009), 1781–1788.Google Scholar
Cuny, C. and Fan, A.-H.. Study of almost everywhere convergence of series by means of martingale methods. Stochastic Process. Appl. 127 (2017), 2725–2750.Google Scholar
Davenport, H.. On some infinite series involving arithmetical functions (II). Q. J. Math. Oxford 8 (1937), 313–320.Google Scholar
Davenport, H., Erdös, P. and LeVeque, W. J.. On Weyl’s criterion for uniform distribution. Michigan Math. J. 10 (1963), 311–314.Google Scholar
Durand, F. and Schneider, D.. Ergodic averages with deterministic weights. Ann. Inst. Fourier 52(2) (2002), 561–583.Google Scholar
Eisner, T.. A polynomial version of Sarnak’s conjecture. C. R. Math. Acad. Sci. Paris 353 (2015), 569–572.Google Scholar
Fan, A.-H.. Oscillating sequences of higher orders and topological systems of quasi-discrete spectrum. Preprint, 2016.Google Scholar
Fan, A.-H.. Topological Wiener–Wintner ergodic theorem with polynomial weights. Preprint, 2016.Google Scholar
Fan, A.-H.. Almost everywhere convergence of ergodic series. Ergod. Th. & Dynam. Sys. 37(2) (2017), 490–511.Google Scholar
Fan, A.-H.. Fully oscillating sequences and weighted multiple ergodic limit. C. R. Acad. Sci., to appear.Google Scholar
Fan, A.-H. and Jiang, Y. P.. On Ruelle–Perron–Frobenius operators II. Comm. Math. Phys. 223(1) (2001), 143–159.Google Scholar
Fan, A.-H. and Jiang, Y. P.. Oscillating sequences, minimal mean attractability and minimal mean-Lyapunov-stability. Ergod. Th. & Dynam. Sys., doi:10.1017/etds.2016.121, Published online: 14 March 2017, pp. 1–36.Google Scholar
Gelfond, A. O.. Sur les nombres qui ont des propriétés additives et multiplicatives données. Acta Arith. 13 (1968), 259–265.Google Scholar
Hahn, F. and Parry, W.. Minimal dynamical systems with quasi-discrete spectrum. J. Lond. Math. Soc. (2) 40 (1965), 309–323.Google Scholar
Kahane, J. P.. Some Random Series of Functions (Cambridge Studies in Advanced Mathematics, 5) , 2nd edn. Cambridge University Press, 1985.Google Scholar
Konieczny, J.. Gowers norms for the Thue–Morse and Rudin–Shapiro sequences. Preprint, 2016, arXiv:1611.09985.Google Scholar
Krengel, U.. Ergodic Theorems. Walter de Gruyter, Berlin, 1982.Google Scholar
Lesigne, E.. Spectre quasi-discret et théorème ergodique de Wiener–Wintner pour les polymômes. Ergod. Th. & Dynam. Sys. 13 (1993), 676–684.Google Scholar
Lesigne, E. and Mauduit, Ch.. Propriétés ergodiques des suites q-multiplicatives. Compos. Math. 100 (1996), 131–169.Google Scholar
Lesigne, E., Mauduit, Ch. and Mossé, B.. Le théorème ergodique le long d’une suite q-multiplicative. Compos. Math. 93 (1994), 49–79.Google Scholar
Mauduit, Ch., Rivat, J. and Sárközy, A.. On digits of sumsets. Canad. J. Math., online (2016).Google Scholar
Móricz, F.. Moment inequalities and the strong law of large numbers. Z. Wahrscheinlichkeitsth. Verw. Geb. 35 (1976), 299–314.Google Scholar
Sarnak, P.. Three Lectures on the Möbius Function, Randomness and Dynamics, IAS Lecture Notes, 2009; http://publications.ias.edu/sites/default/files/MobiusFunctionsLectures(2).pdf.Google Scholar
Sarnak, P.. Möbius randomness and dynamics. Not. S. Afr. Math. Soc. 43 (2012), 89–97.Google Scholar
Tao, T.. Higher Order Fourier Analysis (Graduate Studies in Mathematics, 142) . American Mathematical Society, Providence, RI, 2012.Google Scholar