Hostname: page-component-6bf8c574d5-w79xw Total loading time: 0 Render date: 2025-02-25T02:43:21.175Z Has data issue: false hasContentIssue false
  • English
  • Français

(Uniform) convergence of twisted ergodic averages

Published online by Cambridge University Press:  13 April 2015

TANJA EISNER  and
BEN KRAUSE
TANJA EISNER
Affiliation:
Institute of Mathematics, University of Leipzig, PO Box 100 920, 04009 Leipzig, Germany email [email protected]
BEN KRAUSE
Affiliation:
UCLA Math Sciences Building, Los Angeles, CA 90095-1555, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $T$ be an ergodic measure-preserving transformation on a non-atomic probability space 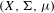$(X,\unicode[STIX]{x1D6F4},\unicode[STIX]{x1D707})$. We prove uniform extensions of the Wiener–Wintner theorem in two settings: for averages involving weights coming from Hardy field functions $p$,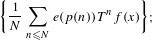

$$\begin{eqnarray}\displaystyle \bigg\{\frac{1}{N}\mathop{\sum }_{n\leq N}e(p(n))T^{n}f(x)\bigg\}; & & \displaystyle \nonumber\end{eqnarray}$$
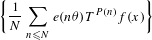and for ‘twisted’ polynomial ergodic averages,
$$\begin{eqnarray}\displaystyle \bigg\{\frac{1}{N}\mathop{\sum }_{n\leq N}e(n\unicode[STIX]{x1D703})T^{P(n)}f(x)\bigg\} & & \displaystyle \nonumber\end{eqnarray}$$
 for certain classes of badly approximable $\unicode[STIX]{x1D703}\in [0,1]$. We also give an elementary proof that the above twisted polynomial averages converge pointwise 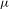$\unicode[STIX]{x1D707}$-almost everywhere for 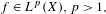$f\in L^{p}(X),p>1,$ and arbitrary 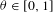$\unicode[STIX]{x1D703}\in [0,1]$.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 7 , October 2016 , pp. 2172 - 2202
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Assani, I.. Wiener Wintner Ergodic Theorems. World Scientific, River Edge, NJ, 2003.CrossRefGoogle Scholar
Assani, I., Duncan, D. and Moore, R.. Pointwise characteristic factors for Wiener Wintner double recurrence theorem. Preprint, 2014, arXiv:1402.7094.Google Scholar
Assani, I. and Moore, R.. Extension of Wiener–Wintner double recurrence theorem to polynomials II. Preprint, 2014, arXiv:1409.0463.Google Scholar
Assani, I. and Presser, K.. A survey of the return times theorem. Ergodic Theory and Dynamical Systems, Proceedings of the 2011–2012, UNC-Chapel Hill Workshops. Ed. Assani, I.. Walter de Gruyter, Berlin, 2014.Google Scholar
Bernstein, S. N.. Leçons sur les Propriétés Extrémales et la Meilleure Approximation des Fonctions Analytiques d’une Variable Réele. Gauthier-Villars et Cie, Paris, 1926.Google Scholar
Birkhoff, G. D.. Proof of the ergodic theorem. Proc. Natl. Acad. Sci. USA 17 (1931), 656–660.CrossRefGoogle ScholarPubMed
Boshernitzan, M. D.. Uniform distribution and Hardy fields. J. Anal. Math. 62 (1994), 225–240.Google Scholar
Boshernitzan, M., Kolesnik, G., Quas, A. and Wierdl, M.. Ergodic averaging sequences. J. Anal. Math. 95 (2005), 63–103.CrossRefGoogle Scholar
Boshernitzan, M. and Wierdl, M.. Ergodic theorems along sequences and Hardy fields. Proc. Natl. Acad. Sci. USA 93 (1996), 8205–8207.CrossRefGoogle ScholarPubMed
Bourgain, J.. On the maximal ergodic theorem for certain subsets of the integers. Israel J. Math. 61(1) (1988), 39–72.CrossRefGoogle Scholar
Bourgain, J.. Pointwise ergodic theorems for arithmetic sets. Publ. Math. Inst. Hautes Études Sci. 69 (1989), 5–45, With an appendix by the author, Harry Furstenberg, Yitzhak Katznelson and Donald S. Ornstein.Google Scholar
Bourgain, J.. Double recurrence and almost sure convergence. J. Reine Angew. Math. 404 (1990), 140–161.Google Scholar
Calderón, A.. Ergodic theory and translation invariant operators. Proc. Natl. Acad. Sci. USA 59 (1968), 349–353.CrossRefGoogle ScholarPubMed
Eisner, T., Farkas, B., Haase, M. and Nagel, R.. Operator Theoretic Aspects of Ergodic Theory (Graduate Texts in Mathematics) . Springer, to appear.CrossRefGoogle Scholar
Eisner, T. and Tao, T.. Large values of the Gowers–Host–Kra seminorms. J. Anal. Math. 117 (2012), 133–186.CrossRefGoogle Scholar
Eisner, T. and Zorin-Kranich, P.. Uniformity in the Wiener–Wintner theorem for nilsequences. Discrete Contin. Dyn. Syst. 33 (2013), 3497–3516.CrossRefGoogle Scholar
Falconer, K.. Fractal Geometry: Mathematical Foundations and Applications, 2nd edn. Wiley, Hoboken, NJ, 2003.CrossRefGoogle Scholar
Frantzikinakis, N.. Uniformity in the polynomial Wiener–Wintner theorem. Ergod. Th. & Dynam. Sys. 26 (2006), 1061–1071.Google Scholar
Frantzikinakis, N.. Equidistribution of sparse sequences on nilmanifolds. J. Anal. Math. 109 (2009), 353–395.Google Scholar
Frantzikinakis, N.. Multiple recurrence and convergence for Hardy sequences of polynomial growth. J. Anal. Math. 112 (2010), 79–135.CrossRefGoogle Scholar
Frantzikinakis, N., Johnson, M., Lesigne, E. and Wierdl, M.. Powers of sequences and convergence of ergodic averages. Ergod. Th. & Dynam. Sys. 30 (2010), 1431–1456.CrossRefGoogle Scholar
Frantzikinakis, N. and Wierdl, M.. A Hardy field extension of Szemerédi’s theorem. Adv. Math. 222 (2009), 1–43.CrossRefGoogle Scholar
Host, B. and Kra, B.. Nonconventional ergodic averages and nilmanifolds. Ann. of Math. (2) 161(1) (2005), 397–488.CrossRefGoogle Scholar
Host, B. and Kra, B.. Uniformity seminorms on l ∞ and applications. J. Anal. Math. 108 (2009), 219–276.CrossRefGoogle Scholar
Hua, L.-K.. Introduction to Number Theory, 2nd edn. Springer, Berlin, 1982.Google Scholar
Hutchinson, J.-E.. Fractals and self-similarity. Indiana Univ. Math. J. 30 (1981), 713–747.CrossRefGoogle Scholar
Jenkinson, O.. On the density of Hausdorff dimensions of bounded type continued fraction sets: the Texan conjecture. Stoch. Dyn. 4 (2004), 63–76.CrossRefGoogle Scholar
Kesseböhmer, M. and Zhu, S.. Dimension sets for infinite IFSs: the Texan conjecture. J. Number Theory 116 (2006), 230–246.CrossRefGoogle Scholar
Khintchine, A. Ya.. Continued Fractions. Noordhoff, Groningen, 1963, English transl. by P. Wynn.Google Scholar
Krause, B.. Polynomial ergodic averages converge rapidly: variations on a theorem of Bourgain. Preprint, 2014, arXiv:1402.1803v1.Google Scholar
Krause, B. and Zorin-Kranich, P.. A random pointwise ergodic theorem with Hardy field weights. Preprint, 2014, arXiv:1410.0806v1.Google Scholar
Kuipers, L. and Niederreiter, H.. Uniform Distribution of Sequences (Pure and Applied Mathematics) . Wiley, New York, 1974.Google Scholar
Lesigne, E.. Spectre quasi-discret et théorème ergodique de Wiener–Wintner pour les polynômes. Ergod. Th. & Dynam. Sys. 13 (1993), 767–784.Google Scholar
Mirek, M. and Trojan, B.. Discrete maximal functions in higher dimensions and applications to ergodic theory. Preprint, 2014, arXiv:1405.5566.Google Scholar
Montgomery, H. L.. Harmonic analysis as found in analytic number theory. Twentieth Century Harmonic Analysis. A Celebration (Proceedings of the NATO Advanced Study Institute Held in Il Ciocco, July 2–15). Ed. Byrnes, J. S.. Kluwer, Dordrecht, 2001, pp. 271–293.Google Scholar
Rosenblatt, J. and Wierdl, M.. Pointwise ergodic theorems via harmonic analysis. Ergodic Theory and its Connections with Harmonic Analysis (Alexandria, 1993) (London Mathematical Society Lecture Note Series, 205) . Cambridge University Press, Cambridge, 1995, pp. 3–151.CrossRefGoogle Scholar
Stein, E. M.. Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals. Monographs in Harmonic Analysis, III (Princeton Mathematical Series, 43) . Princeton University Press, Princeton, NJ, 1993.Google Scholar
Tao, T.. An Epsilon of Room, I: Real Analysis. Pages from Year Three of a Mathematical Blog (Graduate Studies in Mathematics, 117) . American Mathematical Society, Providence, RI, 2010.Google Scholar
Vaughan, R. C.. The Hardy–Littlewood Method (Cambridge Tracts in Mathematics, 125) , 2nd edn. Cambridge University Press, Cambridge, 1997.CrossRefGoogle Scholar
Wiener, N. and Wintner, A.. Harmonic analysis and ergodic theory. Amer. J. Math. 63 (1941), 415–426.CrossRefGoogle Scholar