Article contents
Typical properties of periodic Teichmüller geodesics: Lyapunov exponents
Published online by Cambridge University Press: 17 November 2021
Abstract
Consider a component
 ${\cal Q}$
of a stratum in the moduli space of area-one abelian differentials on a surface of genus g. Call a property
${\cal Q}$
of a stratum in the moduli space of area-one abelian differentials on a surface of genus g. Call a property
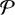 ${\cal P}$
for periodic orbits of the Teichmüller flow on
${\cal P}$
for periodic orbits of the Teichmüller flow on
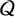 ${\cal Q}$
typical if the growth rate of orbits with property
${\cal Q}$
typical if the growth rate of orbits with property
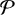 ${\cal P}$
is maximal. We show that the following property is typical. Given a continuous integrable cocycle over the Teichmüller flow with values in a vector bundle
${\cal P}$
is maximal. We show that the following property is typical. Given a continuous integrable cocycle over the Teichmüller flow with values in a vector bundle
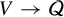 $V\to {\cal Q}$
, the logarithms of the eigenvalues of the matrix defined by the cocycle and the orbit are arbitrarily close to the Lyapunov exponents of the cocycle for the Masur–Veech measure.
$V\to {\cal Q}$
, the logarithms of the eigenvalues of the matrix defined by the cocycle and the orbit are arbitrarily close to the Lyapunov exponents of the cocycle for the Masur–Veech measure.
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 1
- Cited by



