Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gogolev, Andrey
and
Shi, Yi
2023.
Joint integrability and spectral rigidity for Anosov diffeomorphisms.
Proceedings of the London Mathematical Society,
Vol. 127,
Issue. 6,
p.
1693.
Obata, Davi
2023.
Open sets of partially hyperbolic skew products having a unique SRB measure.
Advances in Mathematics,
Vol. 427,
Issue. ,
p.
109136.
Brown, Aaron
Hertz, Federico Rodriguez
and
Wang, Zhiren
2023.
Smooth ergodic theory of $ \mathbb {Z}^d $-actions.
Journal of Modern Dynamics,
Vol. 19,
Issue. 0,
p.
455.
Mongez, Juan
and
Pacifico, Maria
2024.
Finite measures of maximal entropy for an open set of partially hyperbolic diffeomorphisms.
Transactions of the American Mathematical Society,
Crovisier, Sylvain
Obata, Davi
and
Poletti, Mauricio
2024.
Uniqueness of u-Gibbs Measures for Hyperbolic Skew Products on $$\mathbb {T}^4$$.
Communications in Mathematical Physics,
Vol. 405,
Issue. 9,
SAGHIN, RADU
2025.
On invariant holonomies between centers.
Ergodic Theory and Dynamical Systems,
Vol. 45,
Issue. 1,
p.
274.
Mongez, Juan Carlos
and
Pacifico, Maria José
2025.
Robustness and uniqueness of equilibrium states for certain partially hyperbolic systems*
.
Nonlinearity,
Vol. 38,
Issue. 3,
p.
035020.
LIANG, CHAO
SAGHIN, RADU
YANG, FAN
and
YANG, JIAGANG
2025.
Shub’s example revisited.
Ergodic Theory and Dynamical Systems,
Vol. 45,
Issue. 3,
p.
894.
Burguet, David
and
Yang, Dawei
2025.
SRB Measures for Mostly Expanding Partially Hyperbolic Diffeomorphisms via the Variational Approach.
International Mathematics Research Notices,
Vol. 2025,
Issue. 6,
 $C^{1+\beta }$
diffeomorphisms are uniformly bi-Lipschitz and, in fact,
$C^{1+\beta }$
diffeomorphisms are uniformly bi-Lipschitz and, in fact, 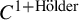 $C^{1+\mathrm {H}\ddot{\rm o}\mathrm {lder}}$
. This verifies the ergodicity of suitably center-bunched, essentially accessible, partially hyperbolic
$C^{1+\mathrm {H}\ddot{\rm o}\mathrm {lder}}$
. This verifies the ergodicity of suitably center-bunched, essentially accessible, partially hyperbolic 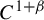 $C^{1+\beta }$
diffeomorphisms and verifies that the Ledrappier–Young entropy formula holds for
$C^{1+\beta }$
diffeomorphisms and verifies that the Ledrappier–Young entropy formula holds for 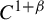 $C^{1+\beta }$
diffeomorphisms of compact manifolds.
$C^{1+\beta }$
diffeomorphisms of compact manifolds.


