Article contents
Pure point/continuous decomposition of translation-bounded measures and diffraction
Published online by Cambridge University Press: 10 July 2018
Abstract
In this work we consider translation-bounded measures over a locally compact Abelian group 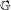 $\mathbb{G}$, with a particular interest in their so-called diffraction. Given such a measure
$\mathbb{G}$, with a particular interest in their so-called diffraction. Given such a measure  $\unicode[STIX]{x1D714}$, its diffraction
$\unicode[STIX]{x1D714}$, its diffraction 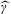 $\widehat{\unicode[STIX]{x1D6FE}}$ is another measure on the Pontryagin dual
$\widehat{\unicode[STIX]{x1D6FE}}$ is another measure on the Pontryagin dual 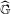 $\widehat{\mathbb{G}}$, whose decomposition into the sum
$\widehat{\mathbb{G}}$, whose decomposition into the sum  $\widehat{\unicode[STIX]{x1D6FE}}=\widehat{\unicode[STIX]{x1D6FE}}_{\text{p}}+\widehat{\unicode[STIX]{x1D6FE}}_{\text{c}}$ of its atomic and continuous parts is central in diffraction theory. The problem we address here is whether the above decomposition of
$\widehat{\unicode[STIX]{x1D6FE}}=\widehat{\unicode[STIX]{x1D6FE}}_{\text{p}}+\widehat{\unicode[STIX]{x1D6FE}}_{\text{c}}$ of its atomic and continuous parts is central in diffraction theory. The problem we address here is whether the above decomposition of 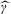 $\widehat{\unicode[STIX]{x1D6FE}}$ lifts to
$\widehat{\unicode[STIX]{x1D6FE}}$ lifts to  $\unicode[STIX]{x1D714}$ itself, that is to say, whether there exists a decomposition
$\unicode[STIX]{x1D714}$ itself, that is to say, whether there exists a decomposition 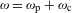 $\unicode[STIX]{x1D714}=\unicode[STIX]{x1D714}_{\text{p}}+\unicode[STIX]{x1D714}_{\text{c}}$, where
$\unicode[STIX]{x1D714}=\unicode[STIX]{x1D714}_{\text{p}}+\unicode[STIX]{x1D714}_{\text{c}}$, where  $\unicode[STIX]{x1D714}_{\text{p}}$ and
$\unicode[STIX]{x1D714}_{\text{p}}$ and 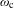 $\unicode[STIX]{x1D714}_{\text{c}}$ are translation-bounded measures having diffraction
$\unicode[STIX]{x1D714}_{\text{c}}$ are translation-bounded measures having diffraction 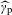 $\widehat{\unicode[STIX]{x1D6FE}}_{\text{p}}$ and
$\widehat{\unicode[STIX]{x1D6FE}}_{\text{p}}$ and 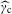 $\widehat{\unicode[STIX]{x1D6FE}}_{\text{c}}$, respectively. Our main result here is the almost sure existence, in a sense to be made precise, of such a decomposition. It will also be proved that a certain uniqueness property holds for the above decomposition. Next, we will be interested in the situation where translation-bounded measures are weighted Meyer sets. In this context, it will be shown that the decomposition, whether it exists, also consists of weighted Meyer sets. We complete this work by discussing a natural generalization of the considered problem.
$\widehat{\unicode[STIX]{x1D6FE}}_{\text{c}}$, respectively. Our main result here is the almost sure existence, in a sense to be made precise, of such a decomposition. It will also be proved that a certain uniqueness property holds for the above decomposition. Next, we will be interested in the situation where translation-bounded measures are weighted Meyer sets. In this context, it will be shown that the decomposition, whether it exists, also consists of weighted Meyer sets. We complete this work by discussing a natural generalization of the considered problem.
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2018
References
- 2
- Cited by


