Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-27T22:23:35.738Z Has data issue: false hasContentIssue false
  • English
  • Français

Projections of fractal percolations

Published online by Cambridge University Press:  11 September 2013

MICHAŁ RAMS  and
KÁROLY SIMON
MICHAŁ RAMS
Affiliation:
Institute of Mathematics, Polish Academy of Sciences, ul. Śniadeckich 8, 00-956 Warsaw, Poland email [email protected]
KÁROLY SIMON
Affiliation:
Institute of Mathematics, Technical University of Budapest, H-1529 B.O. Box 91, Hungary email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

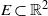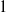In this paper we study the radial and orthogonal projections and the distance sets of the random Cantor sets $E\subset { \mathbb{R} }^{2} $, which are called Mandelbrot percolation or percolation fractals. We prove that the following assertion holds almost surely: if the Hausdorff dimension of $E$ is greater than $1$ then the orthogonal projection to every line, the radial projection with every centre, and the distance set from every point contain intervals.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 35 , Issue 2 , April 2015 , pp. 530 - 545
Copyright
© Cambridge University Press, 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Athreya, K. B. and Ney, P. E.. Branching Processes. Springer, Berlin, 1972.Google Scholar
Chayes, J. T., Chayes, L. and Durrett, R.. Connectivity properties of Mandelbrot’s percolation process. Probab. Theory Related Fields 77 (1988), 307–324.Google Scholar
Dekking, F. M. and Meester, R. W. J.. On the structure of Mandelbrot’s percolation process and other random Cantor sets. J. Stat. Phys. 58 (1990), 1109–1126.Google Scholar
Dekking, F. M. and Simon, K.. On the size of the algebraic difference of two random Cantor sets. Random Structures Algorithms 32 (2008), 205–222.CrossRefGoogle Scholar
Falconer, K. J.. Random fractals. Math. Proc. Cambridge Philos. Soc. 100 (1986), 559–582.CrossRefGoogle Scholar
Falconer, K. J. and Grimmett, G. R.. On the geometry of random Cantor sets and fractal percolation. J. Theoret. Probab. 5 (1992), 465–485.Google Scholar
Falconer, K. J. and Grimmett, G. R.. On the geometry of random Cantor sets and fractal percolation. Correction. J. Theoret. Probab. 7 (1994), 209–210.Google Scholar
Graf, S.. Statistically self-similar fractals. Probab. Theory Related Fields 74 (1987), 357–392.Google Scholar
Hawkes, J.. Trees generated by a simple branching process. J. Lond. Math. Soc. 24 (1981), 373–384.Google Scholar
Hoeffding, W.. Probability inequalities for sums of bounded random variables. J. Amer. Statist. Assoc. 58 (1963), 13–30.Google Scholar
Mandelbrot, B. B.. The Fractal Geometry of Nature. Freeman, San Francisco, CA, 1983.Google Scholar
Marstrand, J. M.. Some fundamental geometrical properties of plane sets of fractional dimensions. Proc. Lond. Math. Soc. 4 (1954), 257–302.Google Scholar
Mauldin, R. D. and Williams, S. C.. Random recursive constructions: asymptotic geometric and topological properties. Trans. Amer. Math. Soc. 295 (1986), 325–346.Google Scholar
Moreira, C. G. and Yoccoz, J.-C.. Stable intersections of regular Cantor sets with large Hausdorff dimensions. Ann. of Math. (2) 154 (1) (2001), 45–96.Google Scholar
Palis, J. and Takens, F.. Fractal dimensions and infinitely many attractors. Hyperbolicity and Sensitive Chaotic Dynamics at Homoclinic Bifurcations (Cambridge Studies in Advanced Mathematics, 35). Cambridge University Press, Cambridge, 1993.Google Scholar
Peres, Y. and Shmerkin, P.. Resonance between Cantor sets. Ergod. Th. & Dynam. Sys. 29 (2009), 201–221.Google Scholar
Peyrière, J.. Mandelbrot random beadsets and birthprocesses with interaction. IBM Research Report RC-7417, 1978.Google Scholar