Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-27T22:04:43.193Z Has data issue: false hasContentIssue false
  • English
  • Français

On the number of fixed points of a sofic shift-flip system

Published online by Cambridge University Press:  20 August 2013

YOUNG-ONE KIM  and
SIEYE RYU
YOUNG-ONE KIM
Affiliation:
Department of Mathematical Sciences and Research Institute of Mathematics, Seoul National University, Seoul 151-747, Korea email [email protected]@snu.ac.kr
SIEYE RYU
Affiliation:
Department of Mathematical Sciences and Research Institute of Mathematics, Seoul National University, Seoul 151-747, Korea email [email protected]@snu.ac.kr
Get access Rights & Permissions [Opens in a new window]

Abstract

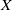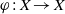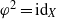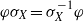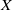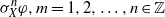If $X$ is a sofic shift and $\varphi : X\rightarrow X$ is a homeomorphism such that ${\varphi }^{2} = {\text{id} }_{X} $ and $\varphi {\sigma }_{X} = { \sigma }_{X}^{- 1} \varphi $, the number of points in $X$ that are fixed by ${ \sigma }_{X}^{m} $ and ${ \sigma }_{X}^{n} \varphi , m= 1, 2, \ldots , n\in \mathbb{Z} $, is expressed in terms of a finite number of square matrices: the matrices are obtained from Krieger’s joint state chain of a sofic shift which is conjugate to 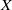$X$.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 35 , Issue 2 , April 2015 , pp. 482 - 498
Copyright
© Cambridge University Press, 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Artin, M. and Mazur, B.. On periodic points. Ann. of Math. 81 (1965), 82–99.Google Scholar
Bowen, R.. On Axiom A Diffeomorphisms (AMS-CBMS Regional Conference, 35). American Mathematical Society, Providence, RI, 1978.Google Scholar
Berstel, J. and Reutenauer, C.. Zeta functions of formal languages. Trans. Amer. Math. Soc. 321 (1990), 533–546.CrossRefGoogle Scholar
Berstel, J. and Reutenauer, C.. Another proof of Soittola’s theorem. Theoret. Comput. Sci. 393 (2008), 196–203.Google Scholar
Eilenberg, S.. Automata, Languages, and Machines. Vol. A. Academic Press, New York, 1974.Google Scholar
Krieger, W.. On sofic systems I. Israel J. Math. 48 (1984), 305–330.Google Scholar
Kim, Y.-O., Lee, J. and Park, K. K.. A zeta function for flip systems. Pacific J. Math. 209 (2003), 289–301.Google Scholar
Lind, D.. A zeta function for ℤd-actions. Ergodic Theory and ℤd-actions (London Mathematical Society Lecture Note Series, 228). Eds. Pollicott, M. and Schmidt, K.. Cambridge University Press, Cambridge, 1996, pp. 433–450.Google Scholar
Lind, D. and Marcus, B.. Symbolic Dynamics and Coding. Cambridge University Press, Cambridge, 1995.Google Scholar
Manning, A.. Axiom A diffeomorphisms have rational zeta functions. Bull. Lond. Math. Soc. 3 (1971), 215–220.Google Scholar
Reutenauer, C.. $N$-rationality of zeta functions. Adv. Appl. Math. 18 (1997), 1–17.Google Scholar
Soittola, M.. Positive rational sequences. Theoret. Comput. Sci. 2 (1976), 317–322.Google Scholar