Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-27T04:13:23.810Z Has data issue: false hasContentIssue false
  • English
  • Français

Homotopical complexity of a billiard flow on the 3D flat torus with two cylindrical obstacles

Published online by Cambridge University Press:  07 September 2017

CALEB C. MOXLEY  and
NANDOR J. SIMANYI
CALEB C. MOXLEY
Affiliation:
Birmingham–Southern College, 900 Arkadelphia Road, Birmingham, AL 35254, USA email [email protected]
NANDOR J. SIMANYI
Affiliation:
The University of Alabama at Birmingham, Department of Mathematics, 1300 University Blvd., Suite 490B, Birmingham, AL 35294, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the homotopical rotation vectors and the homotopical rotation sets for the billiard flow on the unit flat torus with two disjoint and orthogonal toroidal (cylindrical) scatterers removed from it. The natural habitat for these objects is the infinite cone erected upon the Cantor set 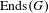$\text{Ends}(G)$ of all ‘ends’ of the hyperbolic group 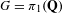$G=\unicode[STIX]{x1D70B}_{1}(\mathbf{Q})$. An element of 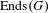$\text{Ends}(G)$ describes the direction in (the Cayley graph of) the group 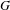$G$ in which the considered trajectory escapes to infinity, whereas the height function $s$ ($s\geq 0$) of the cone gives us the average speed at which this escape takes place. The main results of this paper claim that the orbits can only escape to infinity at a speed not exceeding 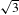$\sqrt{3}$ and, in any direction $e\in \text{Ends}(\unicode[STIX]{x1D70B}_{1}({\mathcal{Q}}))$, the escape is feasible with any prescribed speed $s$, 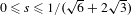$0\leq s\leq 1/(\sqrt{6}+2\sqrt{3})$. This means that the radial upper and lower bounds for the rotation set $R$ are actually pretty close to each other. Furthermore, we prove the convexity of the set 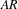$\mathit{AR}$ of constructible rotation vectors, and that the set of rotation vectors of periodic orbits is dense in 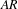$\mathit{AR}$. We also provide effective lower and upper bounds for the topological entropy of the studied billiard flow.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 4 , April 2019 , pp. 1071 - 1081
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Blokh, A. and Misiurewicz, M.. Entropy and over-rotation numbers for interval maps. Proc. Steklov Inst. Math. 216(1) (1997), 229–235.Google Scholar
Blokh, A., Misiurewicz, M. and Simanyi, N.. Rotation sets of billiards with one obstacle. Comm. Math. Phys. 266 (2006), 239–265.Google Scholar
Boyland, P.. New dynamical invariants on hyperbolic manifolds. Israel J. Math. 119 (2000), 253–289.Google Scholar
Bridson, M. R. and Haefliger, A.. Metric Spaces of Non-positive Curvature (Grundlehren der mathematischen Wissenschaften, 319) . Springer, Berlin, 1999.Google Scholar
Coornaert, M. and Papadopoulos, A.. Symbolic Dynamics and Hyperbolic Groups. Springer, New York, 1993.Google Scholar
Franks, J.. Realizing rotation vectors for torus homeomorphisms. Trans. Amer. Math. Soc. 311(1) (1989), 107–115.Google Scholar
Franks, J. and Misiurewicz, M.. Rotation sets of toral flows. Proc. Amer. Math. Soc. 109(1) (1990), 243–249.Google Scholar
Geller, W. and Misiurewicz, M.. Rotation and entropy. Trans. Amer. Math. Soc. 351(7) (1999), 2927–2948.Google Scholar
Jenkinson, O.. Directional entropy of rotation sets. C. R. Acad. Sci. Paris Sér. I Math. 332(10) (2001), 921–926.Google Scholar
Jenkinson, O.. Rotation, entropy, and equilibrium states. Trans. Amer. Math. Soc. 353(9) (2001), 3713–3739.Google Scholar
Kwapisz, J.. Every convex polygon with rational vertices is a rotation set. Ergod. Th. & Dynam. Sys. 12(2) (1992), 333–339.Google Scholar
Mañé, R.. On the topological entropy of geodesic flows. J. Differential Geom. 45(1) (1997), 74–93.Google Scholar
Manning, A.. Topological entropy for geodesic flows. Ann. of Math. (2) 110(3) (1979), 567–573.Google Scholar
Misiurewicz, M.. Rotation intervals for a class of maps of the real line into itself. Ergod. Th. & Dynam. Sys. 6(1) (1986), 117–132.Google Scholar
Misiurewicz, M.. Persistent Rotation Intervals of Old Maps (Banach Center Publications, 23) . Panstwowe Wydawnictwo Naukowe, Warsaw, 1989.Google Scholar
Misiurewicz, M. and Ziemian, K.. Rotation sets for maps of tori. J. Lond. Math. Soc. (2) 40(2) (1989), 490–506.Google Scholar
Morse, M.. A fundamental class of geodesics on any closed surface of genus greater than one. Trans. Amer. Math. Soc. 26 (1924), 25–60.Google Scholar
Moxley, C. and Simanyi, N.. Homotopical complexity of a 3D billiard flow. Proc. Conf. Dynamical Systems, Ergodic Theory, and Probability (Contemporary Mathematics) . Eds. Blokh, A., Bunimovich, I., Jung, P., Oversteegen, L. and Sinai, Ya.. 2017, to appear.Google Scholar
Passeggi, A.. Rational polygons as rotation sets of generic homeomorphisms of the two torus. J. Lond. Math. Soc. (2) 89(1) (2014), 235–254.Google Scholar
Poincaré, H.. Oeuvres Completes. Vol. 1. Gauthier-Villars, Paris, 1952, pp. 137–158.Google Scholar
Schwartzman, S.. Asymptotic cycles. Ann. of Math. (2) 66 (1957), 270–284.Google Scholar
Ziemian, K.. Rotation sets of subshifts of finite type. Fund. Math. 146(2) (1995), 189–201.Google Scholar