Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-09T01:34:24.641Z Has data issue: false hasContentIssue false
  • English
  • Français

Finitely many physical measures for sectional-hyperbolic attracting sets and statistical stability

Part of: Dynamical systems with hyperbolic behavior

Published online by Cambridge University Press:  30 October 2020

VITOR ARAUJO Open the ORCID record for VITOR ARAUJO [Opens in a new window]
VITOR ARAUJO*
Affiliation:
Instituto de Matemática e Estatística, Universidade Federal da Bahia, Av. Ademar de Barros s/n, 40170-110Salvador, Brazil (e-mail: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that a sectional-hyperbolic attracting set for a Hölder-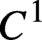 $C^{1}$ vector field admits finitely many physical/SRB measures whose ergodic basins cover Lebesgue almost all points of the basin of topological attraction. In addition, these physical measures depend continuously on the flow in the $C^{1}$ topology, that is, sectional-hyperbolic attracting sets are statistically stable. To prove these results we show that each central-unstable disk in a neighborhood of this class of attracting sets is eventually expanded to contain a ball whose inner radius is uniformly bounded away from zero.

Keywords

sectional hyperbolicityphysical/SRB measuresergodic basinstatistical stabilitytopological basin

MSC classification

Primary: 37D25: Nonuniformly hyperbolic systems (Lyapunov exponents, Pesin theory, etc.)
Secondary: 37D30: Partially hyperbolic systems and dominated splittings 37D20: Uniformly hyperbolic systems (expanding, Anosov, Axiom A, etc.)
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 41 , Issue 9 , September 2021 , pp. 2706 - 2733
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Afraimovich, V. S., Bykov, V. V. and Shil’nikov, L. P.. On the appearence and structure of the Lorenz attractor. Dokl. Acad. Sci. USSR 234 (1977), 336–339.Google Scholar
Alves, J. and Soufi, M.. Statistical stability of geometric Lorenz attractors. Fund. Math. 224(3) (2014), 219–231.CrossRefGoogle Scholar
Araujo, V., Galatolo, S. and Pacifico, M. J.. Decay of correlations for maps with uniformly contracting fibers and logarithm law for singular hyperbolic attractors. Math. Z. 276(3–4) (2014), 1001–1048.CrossRefGoogle Scholar
Araujo, V. and Melbourne, I.. Existence and smoothness of the stable foliation for sectional hyperbolic attractors. Bull. Lond. Math. Soc. 49(2) (2017), 351–367.CrossRefGoogle Scholar
Araujo, V. and Melbourne, I.. Mixing properties and statistical limit theorems for singular hyperbolic flows without a smooth stable foliation. Adv. Math. 349 (2019), 212–245.CrossRefGoogle Scholar
Araujo, V., Melbourne, I. and Varandas, P.. Rapid mixing for the Lorenz attractor and statistical limit laws for their time-1 maps. Comm. Math. Phys. 340(3) (2015), 901–938.CrossRefGoogle Scholar
Araujo, V. and Pacifico, M. J.. Three-dimensional flows . Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge (A Series of Modern Surveys in Mathematics, 53). Springer, Berlin, 2010. With a foreword by Marcelo Viana.Google Scholar
Araujo, V., Pacifico, M. J., Pujals, E. R. and Viana, M.. Singular-hyperbolic attractors are chaotic. Trans. Amer. Math. Soc. 361 (2009), 2431–2485.CrossRefGoogle Scholar
Araujo, V., Souza, A. and Trindade, E.. Upper large deviations bound for singular-hyperbolic attracting sets. J. Dynam. Differential Equations 31(2) (2019), 601–652.CrossRefGoogle Scholar
Araujo, V. and Varandas, P.. Robust exponential decay of correlations for singular-flows. Comm. Math. Phys. 311 (2012), 215–246.CrossRefGoogle Scholar
Bahsoun, W. and Ruziboev, M.. On the statistical stability of Lorenz attractors with a ${c}^{1+\alpha }$ stable foliation. Ergod. Th. & Dynam. Sys. 39(12) (2018), 3169–3184.CrossRefGoogle Scholar
Bálint, P. and Melbourne, I.. Statistical properties for flows with unbounded roof function, including the Lorenz attractor. J. Stat. Phys. 172(4) (2018), 1101–1126.CrossRefGoogle Scholar
Benedicks, M. and Viana, M.. Solution of the basin problem for Hénon-like attractors. Invent. Math. 143(2) (2001), 375–434.CrossRefGoogle Scholar
Bonatti, C., Díaz, L. J. and Viana, M.. Dynamics beyond Uniform Hyperbolicity: A Global Geometric and Probabilistic Perspective ( Encyclopaedia of Mathematical Sciences , 102). Springer, Berlin, 2005.Google Scholar
Bonatti, C., Pumariño, A. and Viana, M.. Lorenz attractors with arbitrary expanding dimension. C. R. Acad. Sci. Paris Sér. I Math. 325(8) (1997), 883–888.CrossRefGoogle Scholar
Bowen, R.. Entropy-expansive maps. Trans. Amer. Math. Soc. 164 (1972), 323–331.CrossRefGoogle Scholar
Bowen, R.. Equilibrium States and the Ergodic Theory of Anosov Diffeomorphisms ( Lecture Notes in Mathematics, 470 ). Springer, Berlin, 1975.CrossRefGoogle Scholar
Bowen, R. and Ruelle, D.. The ergodic theory of Axiom A flows. Invent. Math. 29 (1975), 181–202.CrossRefGoogle Scholar
Crovisier, S. and Yang, D.. Robust transitivity of singular hyperbolic attractors. Math. Z. (2020), doi:10.1007/s00209-020-02618-1.CrossRefGoogle Scholar
Crovisier, S., Yang, D. and Zhang, J.. Empirical measures of partially hyperbolic attractors. Comm. Math. Phys. 375(1) (2020), 725–764.CrossRefGoogle Scholar
Dumortier, F., Kokubu, H. and Oka, H.. A degenerate singularity generating geometric Lorenz attractors. Ergodic Th. & Dynam. Sys. 15(5) (1995), 833–856.CrossRefGoogle Scholar
Falconer, K.. Fractal Geometry: Mathematical Foundations and Applications. John Wiley & Sons, New York, 1990.Google Scholar
Galatolo, S. and Pacifico, M. J.. Lorenz like flows: exponential decay of correlations for the Poincaré map, logarithm law, quantitative recurrence. Ergod. Th. & Dynam. Sys. 30 (2010), 703–1737.CrossRefGoogle Scholar
Guckenheimer, J. and Williams, R. F.. Structural stability of Lorenz attractors. Publ. Math. Inst. Hautes Études Sci. 50 (1979), 59–72.CrossRefGoogle Scholar
Holland, M. and Melbourne, I.. Central limit theorems and invariance principles for Lorenz attractors. J. Lond. Math. Soc. (2) 76(2) (2007), 345–364.CrossRefGoogle Scholar
Jordan, T., Naudot, V. and Young, T.. Higher order Birkhoff averages. Dyn. Syst. 24(3) (2009), 299–313.CrossRefGoogle Scholar
Kisaka, M., Kokubu, H. and Oka, H.. Supplement to homoclinic doubling bifurcation in vector fields. Dynamical Systems, Santiago, 1990 (Pitman Research Notes in Mathematics Series 285). Longman Scientific & Technical, Harlow, 1993, pp. 92–116.Google Scholar
Leplaideur, R. and Yang, D.. SRB measure for higher dimensional singular partially hyperbolic flows. Ann. Inst. Fourier 67(2) (2017), 2703–2717.CrossRefGoogle Scholar
Lorenz, E. N.. Deterministic nonperiodic flow. J. Atmosph. Sci. 20 (1963), 130–141.2.0.CO;2>CrossRefGoogle Scholar
Luzzatto, S., Melbourne, I. and Paccaut, F.. The Lorenz attractor is mixing. Comm. Math. Phys. 260(2) (2005), 393–401.CrossRefGoogle Scholar
Mañé, R.. Ergodic Theory and Differentiable Dynamics. Springer, New York, 1987.CrossRefGoogle Scholar
Metzger, R. and Morales, C.. Sectional-hyperbolic systems. Ergod. Th. & Dynam. Sys. 28 (2008), 1587–1597.CrossRefGoogle Scholar
Metzger, R. J. and Morales, C. A.. Stochastic stability of sectional-anosov flows. Preprint, 2015, arXiv:1505.01761.Google Scholar
Morales, C.. Lorenz attractor through saddle-node bifurcations. Ann. Inst. H. Poincaré Anal. Non Linéaire 13 (1996), 589–617.CrossRefGoogle Scholar
Morales, C. and Pujals, E.. Singular strange attractors on the boundary of Morse-Smale systems. Ann. Sci. Éc. Norm. Supér. 30 (1997), 693–717.CrossRefGoogle Scholar
Morales, C. A.. The explosion of singular-hyperbolic attractors. Ergod. Th. & Dynam. Sys. 24(2) (2004), 577–591.CrossRefGoogle Scholar
Morales, C. A.. Examples of singular-hyperbolic attracting sets. Dyn. Syst. 22(3) (2007), 339–349.CrossRefGoogle Scholar
Morales, C. A., Pacifico, M. J. and Pujals, E. R.. Robust transitive singular sets for 3-flows are partially hyperbolic attractors or repellers. Ann. Math. (2) 160(2) (2004), 375–432.CrossRefGoogle Scholar
Morales, C. A., Pacifico, M. J. and Martin, B. S.. Expanding Lorenz attractors through resonant double homoclinic loops. SIAM J. Math. Anal. 36(6) (2005), 1836–1861.CrossRefGoogle Scholar
Pacifico, M. J., Yang, F., and Yang, J.. Entropy theory for sectional hyperbolic flows. Preprint, 2019, arXiv:1901.07436.Google Scholar
Palis, J. and de Melo, W.. Geometric Theory of Dynamical Systems. Springer, New York, 1982.CrossRefGoogle Scholar
Pesin, Y. B.. Lectures on Partial Hyperbolicity and Stable Ergodicity ( Zurich Lectures in Advanced Mathematics , 1). European Mathematical Society, Zurich, 2004.CrossRefGoogle Scholar
Pugh, C. and Shub, M.. Ergodicity of Anosov actions. Invent. Math. 15 (1972), 1–23.CrossRefGoogle Scholar
Robinson, C.. Homoclinic bifurcation to a transitive attractor of Lorenz type. Nonlinearity 2(4) (1989), 495–518.CrossRefGoogle Scholar
Robinson, C.. Homoclinic bifurcation to a transitive attractor of Lorenz type. II. SIAM J. Math. Anal. 23(5) (1992), 1255–1268.CrossRefGoogle Scholar
Robinson, C.. Nonsymmetric Lorenz attractors from a homoclinic bifurcation. SIAM J. Math. Anal., 32(1) (2000), 119–141.CrossRefGoogle Scholar
Ruelle, D.. A measure associated with Axiom A attractors. Amer. J. Math. 98 (1976), 619–654.CrossRefGoogle Scholar
Ruelle, D.. An inequality for the entropy of differentiable maps. Bol. Soc. Bras. Mat. 9 (1978), 83–87.CrossRefGoogle Scholar
Rychlik, M. R.. Lorenz attractors through Šil’nikov-type bifurcation. I. Ergod. Th. & Dynam. Sys. 10(4) (1990), 793–821.CrossRefGoogle Scholar
Sataev, E. A.. Some properties of singular hyperbolic attractors. Sb. Math. 200(1) (2009), 35.CrossRefGoogle Scholar
Sataev, E. A.. Invariant measures for singular hyperbolic attractors. Sb. Math. 201(3) (2010) 419.CrossRefGoogle Scholar
Sinai, Y.. Gibbs measures in ergodic theory. Russian Math. Surveys 27 (1972), 21–69.CrossRefGoogle Scholar
Smale, S.. Differentiable dynamical systems. Bull. Amer. Math. Soc. 73 (1967), 747–817.CrossRefGoogle Scholar
Smania, D. and Vidarte, J.. Existence of ${c}^k$ -invariant foliations for Lorenz-type maps. J. Dynam. Differential Equations 30(1) (2018), 227–255.CrossRefGoogle Scholar
Takens, F.. Heteroclinic attractors: time averages and moduli of topological conjugacy. Bull. Braz. Math. Soc. 25 (1995), 107–120.CrossRefGoogle Scholar
Yang, D.. On the historical behavior of singular hyperbolic attractors. Proc. Amer. Math. Soc. 148(4) (2019), 1641–1644.CrossRefGoogle Scholar