Article contents
Ergodicity of Iwasawa continued fractions via markable hyperbolic geodesics
Published online by Cambridge University Press: 28 March 2022
Abstract
We prove the convergence and ergodicity of a wide class of real and higher-dimensional continued fraction algorithms, including folded and
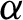 $\alpha $
-type variants of complex, quaternionic, octonionic, and Heisenberg continued fractions, which we combine under the framework of Iwasawa continued fractions. The proof is based on the interplay of continued fractions and hyperbolic geometry, the ergodicity of geodesic flow in associated modular manifolds, and a variation on the notion of geodesic coding that we refer to as geodesic marking. As a corollary of our study of markable geodesics, we obtain a generalization of Serret’s tail-equivalence theorem for almost all points. The results are new even in the case of some real and complex continued fractions.
$\alpha $
-type variants of complex, quaternionic, octonionic, and Heisenberg continued fractions, which we combine under the framework of Iwasawa continued fractions. The proof is based on the interplay of continued fractions and hyperbolic geometry, the ergodicity of geodesic flow in associated modular manifolds, and a variation on the notion of geodesic coding that we refer to as geodesic marking. As a corollary of our study of markable geodesics, we obtain a generalization of Serret’s tail-equivalence theorem for almost all points. The results are new even in the case of some real and complex continued fractions.
Keywords
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
- 2
- Cited by



