Published online by Cambridge University Press: 07 July 2022
Let
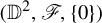 $(\mathbb {D}^2,\mathscr {F},\{0\})$
be a singular holomorphic foliation on the unit bidisc
$(\mathbb {D}^2,\mathscr {F},\{0\})$
be a singular holomorphic foliation on the unit bidisc
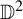 $\mathbb {D}^2$
defined by the linear vector field
$\mathbb {D}^2$
defined by the linear vector field
 $$ \begin{align*} z \frac{\partial}{\partial z}+ \unicode{x3bb} w \frac{\partial}{\partial w}, \end{align*} $$
$$ \begin{align*} z \frac{\partial}{\partial z}+ \unicode{x3bb} w \frac{\partial}{\partial w}, \end{align*} $$
where
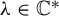 $\unicode{x3bb} \in \mathbb {C}^*$
. Such a foliation has a non-degenerate singularity at the origin
$\unicode{x3bb} \in \mathbb {C}^*$
. Such a foliation has a non-degenerate singularity at the origin
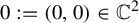 ${0:=(0,0) \in \mathbb {C}^2}$
. Let T be a harmonic current directed by
${0:=(0,0) \in \mathbb {C}^2}$
. Let T be a harmonic current directed by
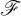 $\mathscr {F}$
which does not give mass to any of the two separatrices
$\mathscr {F}$
which does not give mass to any of the two separatrices
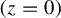 $(z=0)$
and
$(z=0)$
and
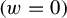 $(w=0)$
. Assume
$(w=0)$
. Assume
 $T\neq 0$
. The Lelong number of T at
$T\neq 0$
. The Lelong number of T at
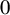 $0$
describes the mass distribution on the foliated space. In 2014 Nguyên (see [16]) proved that when
$0$
describes the mass distribution on the foliated space. In 2014 Nguyên (see [16]) proved that when
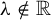 $\unicode{x3bb} \notin \mathbb {R}$
, that is, when
$\unicode{x3bb} \notin \mathbb {R}$
, that is, when
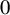 $0$
is a hyperbolic singularity, the Lelong number at
$0$
is a hyperbolic singularity, the Lelong number at
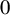 $0$
vanishes. Suppose the trivial extension
$0$
vanishes. Suppose the trivial extension
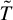 $\tilde {T}$
across
$\tilde {T}$
across
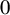 $0$
is
$0$
is
 $dd^c$
-closed. For the non-hyperbolic case
$dd^c$
-closed. For the non-hyperbolic case
 $\unicode{x3bb} \in \mathbb {R}^*$
, we prove that the Lelong number at
$\unicode{x3bb} \in \mathbb {R}^*$
, we prove that the Lelong number at
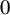 $0$
:
$0$
:
(1) is strictly positive if
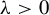 $\unicode{x3bb}>0$
;
$\unicode{x3bb}>0$
;
(2) vanishes if
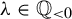 $\unicode{x3bb} \in \mathbb {Q}_{<0}$
;
$\unicode{x3bb} \in \mathbb {Q}_{<0}$
;
(3) vanishes if
 $\unicode{x3bb} <0$
and T is invariant under the action of some cofinite subgroup of the monodromy group.
$\unicode{x3bb} <0$
and T is invariant under the action of some cofinite subgroup of the monodromy group.