Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-27T04:20:06.092Z Has data issue: false hasContentIssue false
  • English
  • Français

Convergence for moving averages

Published online by Cambridge University Press:  19 September 2008

Alexandra Bellow ,
Roger Jones  and
Joseph Rosenblatt
Alexandra Bellow
Affiliation:
Mathematics Department, Northwestern University, Evanston, IL 60201, USA
Roger Jones
Affiliation:
Mathematics Department, DePaul University, 2323 N. Seminary, Chicago, IL 60614, USA
Joseph Rosenblatt
Affiliation:
Mathematics Department, Ohio State University, Columbus, OH 43210, USA
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Assume T is an ergodic measure preserving point transformation from a probability space onto itself. Let be a sequence of pairs of positive integers, and define the sequence of averaging operators 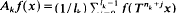. Necessary and sufficient conditions are given forthis sequence of averages to converge almost everywhere. Weighted versions are also considered.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 10 , Issue 1 , March 1990 , pp. 43 - 62
Copyright
Copyright © Cambridge University Press 1990

References

REFERENCES

[1]Akcoglu, M. & del Junco, A.. Convergence of averages of point transformations. Proc. Amer. Math. Soc. 49 (1975), 265–266.Google Scholar
[2]Bellow, A. & Losert, V.. On sequences of density zero in ergodic theory. Contemp. Math. 28 (1984), 49–60.CrossRefGoogle Scholar
[3]Bellow, A. & Losert, V.. The weighted pointwise ergodic theorem and the individual ergodic the along subsequences. Trans. Amer. Math. Soc. 288 (1985), 307–345.CrossRefGoogle Scholar
[4]Calderón, A. P.. Ergodic theory and translation-invariant operators. Proc. Nat. Acad. Sci. 59 (1968) 349–353.CrossRefGoogle ScholarPubMed
[5]de Guzman, M.. Real Variable Methods in Fourier Analysis, North-Holland: Math. Studies 46, 1988.Google Scholar
[6]del Junco, A. & Rosenblatt, J.. ‘Counterexamples in ergodic theory and number theory. Math., 247 (1979), 185–197.Google Scholar
[7]Nagel, A. & Stein, E. M.. On certain maximal functions and approach regions. Adv. Math. 54 (1984) 83–106.CrossRefGoogle Scholar
[8]Rosenblatt, J.. Ergodic group actions. Arch. Math. 47 (1986), 263–269.CrossRefGoogle Scholar
[9]Sawyer, S.. Maximal inequalities of weak type. Ann. Math. 84 (1966), 157–174.CrossRefGoogle Scholar
[10]Schwartz, M.. Polynomially moving ergodic averages. To appear in Proc. Amer. Math. Soc.Google Scholar
[11]Stein, E. M.. On limits of sequences of operators. Ann. of Math 74 (1961), 140–170.CrossRefGoogle Scholar
[12]Sueiro, J.. A note on maximal operators of Hardy-Littlewood type. Math. Proc. Camb. Phil 102 (1987), 131–134.CrossRefGoogle Scholar
[13]Zygmund, A.. Trigonometric Series (Volume II), Cambridge University Press: London, 1968.Google Scholar