Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T13:59:58.404Z Has data issue: false hasContentIssue false
  • English
  • Français

Compact metric spaces, Fredholm modules, and hyperfiniteness

Published online by Cambridge University Press:  19 September 2008

A. Connes
A. Connes
Affiliation:
IHES, Bures-sur-Yvette, 91440, France
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

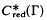We show that the existence of a finitely summable unbounded Fredholm module (h, D) on a C* algebra A implies the existence of a trace state on A and that no such module exists on the C* algebra of a non amenable discrete group. Both for the needs of non commutative differential geometry and of analysis in infinite dimension we are led to the better notion of the θ-summable Fredholm module.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 9 , Issue 2 , June 1989 , pp. 207 - 220
Copyright
Copyright © Cambridge University Press 1989

References

REFERENCES

[1]Baaj, S. & Julg, P.. Théorie bivariante de Kasparov et opérateurs non bornés dans les C*-modules Hilbertiens. C.R. Acad. Sci. Paris, Série I, 296 (1983), 875–878.Google Scholar
[2]Baum, P. & Connes, A.. Geometric K-theory for Lie groups and foliations. Preprint I.H.E.S. (1982).Google Scholar
[3]Bedos, E. & Harpe, P. de la. Moyennabilité intérieure des groupes, définitions et exemples. Preprint, Genève.Google Scholar
[4]Connes, A. & Krieger, W.. Measure space automorphisms, the normalizer of their full groups and approximate finiteness. J. Funct. Analysis 29 (1977), 336.CrossRefGoogle Scholar
[5]Connes, A., Feldman, J. & Weiss, B.. An amenable equivalence relation is generated by a single transformation. Ergod. Th. & Dynam. Sys. 1 (1981), 431–450.CrossRefGoogle Scholar
[6]Connes, A., C* algebres et géométrie différentielle, C.R. Acad. Sci. Paris, Série I, 290 (1980), 599, 605.Google Scholar
[7]Connes, A.. Non commutative differential geometry, Publ. IHES 62 (1985), 41–144.CrossRefGoogle Scholar
[8]Connes, A.. Classification of injective factors. Ann. Math. 104 (1976), 73–115.CrossRefGoogle Scholar
[9]Connes, A. & Rieffel, M.. Yang Mills for the non commutative two tori. Contemp. Math. Operator Algebras and Math. Physics (1986), 237–267.Google Scholar
[10]Connes, A. & Moscovici, H.. Transgression du caractère de Chern et cohomologie cyclique. C.R. Acad. Sc. Paris, Série I, 303 (1986), 913–918.Google Scholar
[11]Delorme, P.. 1-cohomologie des représentations unitaires des groupes de Lie semi simples et résolubles. Bull. Soc. Math. France 105 (1977), 281–336.Google Scholar
[12]Dixmier, J.. Les Algèbres d'opérateurs dans l'espace Hilbertien 2ème édition (Gauthier Villars: Paris, 1969).Google Scholar
[13]Dye, H.. On groups of measure preserving transformations I. Amer. J. Math. 81 (1959), 119–159;CrossRefGoogle Scholar
II Amer. J. Math. 85 (1963), 551–576.CrossRefGoogle Scholar
[14]Gilkey, P.. Invariance theory, the heat equation and the Atiyah Singer Index theorem. Math. Lecture Series II (Publish or Perish Inc., 1984).Google Scholar
[15]Gromov, M.. Groups of polynomial growth and expanding maps. Publ. IHES 53 (1981), 53–78.CrossRefGoogle Scholar
[16]Hörmander, L.. On the index of pseudo differential operators. Elliptische Differentialgleischungen Band II, Koll. Berlin (1969).Google Scholar
[17]Jaffe, A., Lesniewski, A. & Weitsman, J.. Index of a family of Dirac operators on loop space. Preprint Harvard (1987).CrossRefGoogle Scholar
[18]Kasparov, G.. K. functor and extensions of C* algebras. Izv. Akad. Nauk. SSSR, Ser. Mat. 44 (1980), 571–636.Google Scholar
[19]Kasparov, G.. K. theory, group C* algebras and higher signatures. Conspectus, Chernogolovka (1983), to appear in Inventiones Math.Google Scholar
[20]Kazhdan, D.. Relations entre I'espace dual d'un groupe et la structure de ses sous groupes fermés. Funkt. Anal. i. Priloj. 1 (1967) 71–74.Google Scholar
[21]Kostant, B.. On the existence and irreducibility of certain series of representations. Bull. A.M.S. 75 (1969), 627–642.CrossRefGoogle Scholar
[22]Miscenko, A. S.. Infinite dimensional representations of discrete groups and higher signatures. Math. U.S.S.R. Izv. 8 (1974), 85–112.CrossRefGoogle Scholar
[23]Ornstein, D. & Weiss, B.. Ergodic theory of amenable group actions I, the Rohlin lemma. Bull. AMS. 2 (1980), 161.CrossRefGoogle Scholar
[24]Powers, R. T. & Stormer, E.. Free states of the canonical anticommutation relations. Commun. Math. Phys. 16 (1970), 1–33.CrossRefGoogle Scholar
[25]Quillen, D.. Superconnections and the Chern character. Topology 24 (1985), 89–95.CrossRefGoogle Scholar
[26]Sakai, S.. C* and W* algebras. Ergebnisse der Mathematik un ihrer Grenzgebiete, Band 60.Google Scholar
[27]Teleman, N.. The index of signature operators on Lipschitz manifolds. Publ. Math. IHES 58 (1983), 39–78.CrossRefGoogle Scholar
[28]Wang, S.. On the first cohomology group of discrete groups with property T. Proc. Amer. Math. Soc. t. 42 (1974), 621–624.Google Scholar
[29]Witten, E.. Supersymmetry and Morse theory. J. Diff. Geom. 17 (4) (1982), 661–692.Google Scholar