Article contents
A proof of the Kuramoto conjecture for a bifurcation structure of the infinite-dimensional Kuramoto model
Published online by Cambridge University Press: 11 October 2013
Abstract
The Kuramoto model is a system of ordinary differential equations for describing synchronization phenomena defined as coupled phase oscillators. In this paper, a bifurcation structure of the infinite-dimensional Kuramoto model is investigated. A purpose here is to prove the bifurcation diagram of the model conjectured by Kuramoto in 1984; if the coupling strength 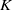 $K$ between oscillators, which is a parameter of the system, is smaller than some threshold
$K$ between oscillators, which is a parameter of the system, is smaller than some threshold 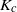 ${K}_{c} $, the de-synchronous state (trivial steady state) is asymptotically stable, while if
${K}_{c} $, the de-synchronous state (trivial steady state) is asymptotically stable, while if  $K$ exceeds
$K$ exceeds  ${K}_{c} $, a non-trivial stable solution, which corresponds to the synchronization, bifurcates from the de-synchronous state. One of the difficulties in proving the conjecture is that a certain non-selfadjoint linear operator, which defines a linear part of the Kuramoto model, has the continuous spectrum on the imaginary axis. Hence, the standard spectral theory is not applicable to prove a bifurcation as well as the asymptotic stability of the steady state. In this paper, the spectral theory on a space of generalized functions is developed with the aid of a rigged Hilbert space to avoid the continuous spectrum on the imaginary axis. Although the linear operator has an unbounded continuous spectrum on a Hilbert space, it is shown that it admits a spectral decomposition consisting of a countable number of eigenfunctions on a space of generalized functions. The semigroup generated by the linear operator will be estimated with the aid of the spectral theory on a rigged Hilbert space to prove the linear stability of the steady state of the system. The center manifold theory is also developed on a space of generalized functions. It is proved that there exists a finite-dimensional center manifold on a space of generalized functions, while a center manifold on a Hilbert space is of infinite dimension because of the continuous spectrum on the imaginary axis. These results are applied to the stability and bifurcation theory of the Kuramoto model to obtain a bifurcation diagram conjectured by Kuramoto.
${K}_{c} $, a non-trivial stable solution, which corresponds to the synchronization, bifurcates from the de-synchronous state. One of the difficulties in proving the conjecture is that a certain non-selfadjoint linear operator, which defines a linear part of the Kuramoto model, has the continuous spectrum on the imaginary axis. Hence, the standard spectral theory is not applicable to prove a bifurcation as well as the asymptotic stability of the steady state. In this paper, the spectral theory on a space of generalized functions is developed with the aid of a rigged Hilbert space to avoid the continuous spectrum on the imaginary axis. Although the linear operator has an unbounded continuous spectrum on a Hilbert space, it is shown that it admits a spectral decomposition consisting of a countable number of eigenfunctions on a space of generalized functions. The semigroup generated by the linear operator will be estimated with the aid of the spectral theory on a rigged Hilbert space to prove the linear stability of the steady state of the system. The center manifold theory is also developed on a space of generalized functions. It is proved that there exists a finite-dimensional center manifold on a space of generalized functions, while a center manifold on a Hilbert space is of infinite dimension because of the continuous spectrum on the imaginary axis. These results are applied to the stability and bifurcation theory of the Kuramoto model to obtain a bifurcation diagram conjectured by Kuramoto.
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2013
References
- 59
- Cited by


