Article contents
Gibbs measures for foliated bundles with negatively curved leaves
Published online by Cambridge University Press: 15 December 2016
Abstract
In this paper we develop a notion of Gibbs measure for the geodesic flow tangent to a foliated bundle over a compact negatively curved base. We also develop a notion of  $F$-harmonic measure and prove that there exists a natural bijective correspondence between these two concepts. For projective foliated bundles with
$F$-harmonic measure and prove that there exists a natural bijective correspondence between these two concepts. For projective foliated bundles with 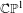 $\mathbb{C}\mathbb{P}^{1}$-fibers without transverse invariant measure, we show the uniqueness of these measures for any Hölder potential on the base. In that case we also prove that
$\mathbb{C}\mathbb{P}^{1}$-fibers without transverse invariant measure, we show the uniqueness of these measures for any Hölder potential on the base. In that case we also prove that  $F$-harmonic measures are realized as weighted limits of large balls tangent to the leaves and that their conditional measures on the fibers are limits of weighted averages on the orbits of the holonomy group.
$F$-harmonic measures are realized as weighted limits of large balls tangent to the leaves and that their conditional measures on the fibers are limits of weighted averages on the orbits of the holonomy group.
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2016
References
- 4
- Cited by


