No CrossRef data available.
Published online by Cambridge University Press: 02 May 2023
This article analyzes the theoretical properties of the hybrid test for superior predictive ability. A simple example reveals that the test may not be size-controlled at common significance levels with rejection rates exceeding  $11\%$ at a
$11\%$ at a 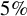 $5\%$ nominal level. Generalizing this observation, the main results show the pointwise asymptotic invalidity of the hybrid test under reasonable conditions. Monte Carlo simulations support these theoretical findings.
$5\%$ nominal level. Generalizing this observation, the main results show the pointwise asymptotic invalidity of the hybrid test under reasonable conditions. Monte Carlo simulations support these theoretical findings.
I would like to thank the Editor, the Co-Editor, and the two anonymous referees for their helpful comments on the earlier version. I am indebted to Ivan Canay for his invaluable guidance and support. Thanks also go to Yoon-Jae Whang who guided my Master’s thesis, from which this project originated, as well as Joel Horowitz, Eric Auerbach, Myungkou Shin, Yong Cai, and participants of the Econometrics Reading Group at Northwestern for their helpful comments. I would also like to thank Carl Hallmann for proofreading the article. All errors are my own.