Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T07:09:24.736Z Has data issue: false hasContentIssue false
  • English
  • Français

IDENTIFICATION AND ESTIMATION IN A CORRELATED RANDOM COEFFICIENTS TRANSFORMATION MODEL

Published online by Cambridge University Press:  04 June 2021

Zhengyu Zhang ,
Zequn Jin  and
Beili Mu
Zhengyu Zhang
Affiliation:
Shanghai University of Finance and Economics
Zequn Jin*
Affiliation:
Shanghai University of Finance and Economics
Beili Mu
Affiliation:
Chinabond Pricing Center, China Central Depository & Clearing Co., LTD.
*
Address correspondence to Zequn Jin, School of Economics, Shanghai University of Finance and Economics, No. 777, Guoding Road, Shanghai, P.R. China; e-mail: [email protected].
Get access Rights & Permissions [Opens in a new window]

Abstract

This study examines identification and estimation in a correlated random coefficients (CRC) model with an unknown transformation of the dependent variable, namely 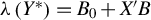$\lambda \left (Y^{*}\right)=B_{0}+X^{\prime }B$, where the latent outcome 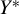$Y^{*}$ may be subject to a certain kind of censoring mechanism, $\lambda (\cdot)$ is an unknown, one-to-one monotone function, and the random coefficients 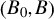$\left (B_{0},B\right)$ are allowed to be correlated with one or several components of X. Under a conditional median independence plus a conditional median zero restriction, the mean of B is shown to be identified up to scale. Moreover, we show the derivative of the median structural function (MSF) is point identified. This derivative of MSF resembles the marginal treatment effect introduced by Heckman and Vytlacil (2005, Econometrica 73, 669–738).

It generalizes the usual average treatment effect in a linear CRC model and coincides with 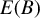$E(B)$ when 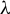$\lambda $ is equal to the identity function; it is invariant to both location and scale normalization on the coefficients. We develop estimators for the identified parameters and derive asymptotic properties for the derivative of MSF. An empirical example using the U.K. Family Expenditure Survey is provided.

Type
ARTICLES
Information
Econometric Theory , Volume 38 , Issue 4 , August 2022 , pp. 621 - 688
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

We are grateful to the editor Peter C.B. Phillips, the co-editor Arthur Lewbel, and four anonymous referees for their insightful comments. This research is supported by the National Science Foundation of China (71873080), the Key Project of the National Science Foundation of China (71833004), the Fundamental Research Funds for the Central Universities (2021110070), and the 2018 Program for Innovative Research Team of the Shanghai University of Finance and Economics.

References

REFERENCES

Abrevaya, J. (1999) Rank estimation of a transformation model with observed truncation. Econometrics Journal 2(2), 292–305.10.1111/1368-423X.00034CrossRefGoogle Scholar
Abrevaya, J. (2003) Pairwise-difference rank estimation of the transformation model. Journal of Business and Economic Statistics 21(3), 437–447.10.1198/073500103288619070CrossRefGoogle Scholar
Asparouhova, E., Golanski, R., Kasprzyk, K., & Asparouhov, S. T. (2002) Rank estimators for a transformation model. Econometric Theory 18(5), 1099–1120.10.1017/S0266466602185045CrossRefGoogle Scholar
Blundell, R., Chen, X., & Kristensen, D. (2007) Semi-nonparametric IV estimation of shape-invariant Engel curves. Econometrica 75(6), 1613–1669.10.1111/j.1468-0262.2007.00808.xCrossRefGoogle Scholar
Box, G. E. P. & Cox, D. R. (1964) An analysis of transformations. Journal of the Royal Statistical Society 26(2), 211–252.Google Scholar
Buchinsky, M. (1995) Quantile regression, Box–Cox transformation model, and the U.S. wage structure, 1963–1987. Journal of Econometrics 65(1), 109–154.10.1016/0304-4076(94)01599-UCrossRefGoogle Scholar
Carroll, R. J., Fan, J., Gijbels, I. & Wand, M. P. (1997) Generalized partially linear single-index models. Journal of the American Statistical Association 92(438), 477–489.10.1080/01621459.1997.10474001CrossRefGoogle Scholar
Cavanagh, C. & Sherman, R. P. (1998) Rank estimators for monotonic index models. Journal of Econometrics 84(2), 351–381.10.1016/S0304-4076(97)00090-0CrossRefGoogle Scholar
Chen, K., Jin, Z., & Ying, Z. (2002) Semiparametric analysis of transformation models with censored data. Biometrika 89(3), 659–668.10.1093/biomet/89.3.659CrossRefGoogle Scholar
Chen, S. (2002) Distribution-free estimation of the Box–Cox regression model with censoring. Econometric Theory 28(3), 680–695.CrossRefGoogle Scholar
Chen, S. (2010) An integrated maximum score estimator for a generalized censored quantile regression model. Journal of Econometrics 155(1), 90–98.10.1016/j.jeconom.2009.09.020CrossRefGoogle Scholar
Chen, X., Linton, O. & Van Keilegom, I. (2003) Estimation of semiparametric models when the criterion function is not smooth. Econometrica 71(5), 1591–1608.CrossRefGoogle Scholar
Chernozhukov, V., Fernández-Val, I., & Melly, B. (2013) Inference on counterfactual distributions. Econometrica 81(6), 2205–2268.Google Scholar
Chesher, A. (2003) Identification in nonseparable models. Econometrica 71(5), 1405–1441.CrossRefGoogle Scholar
Chiappori, P. A., Komunjer, I. & Kristensen, D. (2015) Nonparametric identification and estimation of transformation models. Journal of Econometrics 188(1), 22–39.CrossRefGoogle Scholar
Feve, F. & Florens, J. P. (2010) The practice of non-parametric estimation by solving inverse problems: The example of transformation models. Econometrics Journal 13(3), S1–S27.CrossRefGoogle Scholar
Florens, J. P., Heckman, J. J., Meghir, C. & Vytlacil, E. (2008) Identification of treatment effects using control functions in model with continuous, endogenous treatment and heterogeneous effects. Econometrica 76, 1191–1206.Google Scholar
Florens, J. P. & Sokullu, S. (2017) Nonparametric estimation of semiparametric transformation models. Econometric Theory 33(4), 35.CrossRefGoogle Scholar
Gine, E. & Zinn, J. (1990) Bootstrapping general empirical measures. The Annals of Probability 18(2), 851–869.CrossRefGoogle Scholar
Hall, P. (1991) On convergence rates of suprema. Probability Theory and Related Fields 89(4), 447–455.CrossRefGoogle Scholar
Heckman, J. & Vytlacil, E. (1998) Instrumental variables methods for the correlated random coefficient model: Estimating the average rate of return to schooling when the return is correlated with schooling. The Journal of Human Resources 33(4), 974–987.CrossRefGoogle Scholar
Heckman, J. & Vytlacil, E. (2005) Structural equations, treatment effects, and econometric policy evaluation. Econometrica 73(3), 669–738.CrossRefGoogle Scholar
Heckman, J. & Vytlacil, E. (2007) Econometric evaluation of social programs, Part I: Causal models, structural models and econometric policy evaluation. In Heckman, J. J. & Leamer, E. E. (eds.), Handbook of Econometrics, vol. 6, pp. 4779–4874. Elsevier.CrossRefGoogle Scholar
Hoderlein, S. & Sherman, R. (2015) Identification and estimation in a correlated random coefficients binary response model. Journal of Econometrics 188, 135–149.CrossRefGoogle Scholar
Horowitz, J. L. (1992) A smoothed maximum score estimator for the binary response model. Econometrica 60(3), 505–531.CrossRefGoogle Scholar
Horowitz, J. L. (1996) Semiparametric estimation of a regression model with an unknown transformation of the dependent variable. Econometrica 64, 103–137. CrossRefGoogle Scholar
Horowitz, J. L. (1998) Semiparametric Methods in Econometrics. Springer, New York.Google Scholar
Horowitz, J. L. (2001) Nonparametric estimation of a generalized additive model with an unknown link function. Econometrica 69(2), 499–513.CrossRefGoogle Scholar
Imbens, G. & Newey, W. (2009) Identification and estimation of triangular simultaneous equations models without additivity. Econometrica 77(5), 1481–1512.Google Scholar
Jacho-Chávez, D., Lewbel, A. & Linton, O. (2010) Identification and nonparametric estimation of a transformed additively separable model. Journal of Econometrics 156(2), 392–407. CrossRefGoogle Scholar
Khan, S., & Tamer, E. (2007) Partial rank estimation of duration models with general forms of censoring. Journal of Econometrics 136(1), 251–280.CrossRefGoogle Scholar
Lewbel, A. & Pendakur, K. (2017) Unobserved preference heterogeneity in demand using generalized random coefficients. Journal of Political Economy 125(4), 1100–1148.CrossRefGoogle Scholar
Li, Q. & Racine, J. S. (2007) Nonparametric Econometrics: Theory and Practice. Princeton University Press.Google Scholar
Linton, O., Sperlich, S., & Van Keilegom, I. (2008) Estimation of a semiparametric transformation model. Annals of Statistics 36(2), 686–718.CrossRefGoogle Scholar
Manski, C. F. (1975) Maximum score estimation of the stochastic utility model of choice. Journal of Econometrics 3(3), 205–228.CrossRefGoogle Scholar
Manski, C. F. (1985) Semiparametric analysis of discrete response: Asymptotic properties of the maximum score estimator. Journal of Econometrics 27(3), 313–333.CrossRefGoogle Scholar
Masten, M. & Torgovitsky, A. (2016) Identification of instrumental variable correlated random coefficients models. Review of Economics and Statistics 98(5), 1001–1005.CrossRefGoogle Scholar
Moon, J.M. (2013) Sieve Extremum Estimation of Transformation Models. Technical report, UCSD, Working paper. Google Scholar
Newey, W. K. & McFadden, D. (1994) Large sample estimation and hypothesis testing. In Engle, R. & McFadden, D. (eds.), Handbook of Econometrics, vol. 4. Elsevier Science.Google Scholar
Powell, J. L., Stock, J. H., & Stoker, T. M. (1989) Semiparametric estimation of index coefficients. Econometrica 57(6), 1403–1430.CrossRefGoogle Scholar
Sherman, R. P. (1994a) U-processes in the analysis of a generalized semiparametric regression estimator. Econometric Theory 10(2), 372–395.CrossRefGoogle Scholar
Sherman, R. P. (1994b) Maximal inequalities for degenerate U-processes with applications to optimization estimators. Annals of Statistics 22(1), 439–459.CrossRefGoogle Scholar
Shin, Y. (2010) Local rank estimation of transformation models with functional coefficients. Econometric Theory 26(6), 1807–1819.CrossRefGoogle Scholar
Vanhems, A. & Van Keilegom, I. (2018) Estimation of a semiparametric transformation model in the presence of endogeneity. Econometric Theory 35(1), 73–110.CrossRefGoogle Scholar
Wooldridge, J. (1997) On two stage least squares estimation of the average treatment effect in a random coefficient model. Economics Letters 56, 129–133.CrossRefGoogle Scholar
Wooldridge, J. (2003) Further results on instrumental variables estimation of average treatment effects in the correlated random coefficient model. Economics Letters 79, 185–191.CrossRefGoogle Scholar
Wooldridge, J. (2008) Instrumental variables estimation of the average treatment effect in the correlated random coefficient model. In Millimet, D., Smith, J., & Vytlacil, E. (eds.), Advances in Econometrics: Modeling and Evaluating Treatment Effects in Econometrics, vol. 21, pp. 93–116. Emerald Group Publishing Limited.Google Scholar
Xu, X. & Lee, L. F. (2015) A spatial autoregressive model with a nonlinear transformation of the dependent variable. Journal of Econometrics 186(1), 1–18.CrossRefGoogle Scholar
Zhang, Z. (2016) Semiparametric estimation of partially linear transformation models under conditional quantile restriction. Econometric Theory 32(2), 458–497.CrossRefGoogle Scholar