Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-27T11:07:56.413Z Has data issue: false hasContentIssue false
  • English
  • Français

ESTIMATION OF THE KRONECKER COVARIANCE MODEL BY QUADRATIC FORM

Published online by Cambridge University Press:  17 December 2020

Oliver B. Linton  and
Haihan Tang
Oliver B. Linton
Affiliation:
University of Cambridge
Haihan Tang*
Affiliation:
Fudan University
*
Address correspondence to Haihan Tang, Fanhai International School of Finance, Fudan University, 220 Handan Road, Yangpu District, Shanghai, 200433, China; e-mail: [email protected].
Get access Rights & Permissions [Opens in a new window]

Abstract

We propose a new estimator, the quadratic form estimator, of the Kronecker product model for covariance matrices. We show that this estimator has good properties in the large dimensional case (i.e., the cross-sectional dimension n is large relative to the sample size T). In particular, the quadratic form estimator is consistent in a relative Frobenius norm sense provided 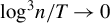${\log }^3n/T\to 0$ . We obtain the limiting distributions of the Lagrange multiplier and Wald tests under both the null and local alternatives concerning the mean vector 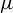$\mu $ . Testing linear restrictions of $\mu $ is also investigated. Finally, our methodology is shown to perform well in finite sample situations both when the Kronecker product model is true and when it is not true.

Type
ARTICLES
Information
Econometric Theory , Volume 38 , Issue 5: YALE 2018 CONFERENCE IN HONOR OF PETER C. B. PHILLIPS: PART I , October 2022 , pp. 1014 - 1067
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

We thank the Editor Peter C.B. Phillips, the Co-Editor Yuichi Kitamura, and two anonymous referees for detailed comments, which greatly improved the article. For useful discussions, we are grateful to Liang Jiang, Chen Wang, Tengyao Wang, and the participants at the Celebration of Peter C.B. Phillips’ Forty Years at Yale held at Yale University on October 19-20, 2018 and the CUHK Workshop on Econometrics 2019. Any remaining errors are our own. Oliver B. Linton thanks the Cambridge INET and the Keynes Fund for financial support. Haihan Tang is sponsored by the National Natural Science Foundation of China (grant number 71903034) and Shanghai Pujiang Program (grant number 2019PJC015).

References

REFERENCES

Akdemir, D. & Gupta, A. K. (2011) Array variate random variables with multiway kronecker delta covariance matrix structure. Journal of Algebraic Statistics 2, 98–113.CrossRefGoogle Scholar
Anderson, T. W. (1984) An Introduction to Multivariate Statistical Analysis. Wiley.Google Scholar
Bai, J. (2009) Panel data models with interactive fixed effects. Econometrica 77, 1229–1279.Google Scholar
Bai, Z. & Saranadasa, H. (1996) Effect of high dimension: By an example of a two sample problem. Statistica Sinica 6, 311–329.Google Scholar
Bickel, P. J. & Levina, E. (2008) Covariance regularization by thresholding. Annals of Statistics 36, 2577–2604.CrossRefGoogle Scholar
Billingsley, P. (1995) Probability and Measure. Wiley.Google Scholar
Brillinger, D. R. (1962) A note on the rate of convergence of a mean. Biometrika 49, 574–576.CrossRefGoogle Scholar
Bühlmann, P. & van de Geer, S. (2011) Statistics for High-Dimensional Data. Springer.CrossRefGoogle Scholar
Campbell, D. T. & O’Connell, E. J. (1967) Method factors in multitrait-multimethod matrices: Multiplicative rather than additive? Multivariate Behaviorial Research 2, 409–426.CrossRefGoogle Scholar
Cho, J. S. & Phillips, P. C. B. (2018) Pythagorean generalization of testing the equality of two symmetric positive definite matrices. Journal of Econometrics 202, 45–56.CrossRefGoogle Scholar
Chudik, A. & Pesaran, M. H. (2013) Large Panel Data Models with Cross-Sectional Dependence: A Survey, Working Paper.CrossRefGoogle Scholar
Cudeck, R. (1988) Multiplicative models and MTMM matrices. Journal of Educational Statistics 13, 131–147.Google Scholar
Estienne, F., Matthijs, N., Massart, D., Ricoux, P., & Leibovici, D. (2001) Multi-way modelling of high-dimensionality electroencephalographic data. Chemometrics and Intelligent Laboratory Systems 58, 59–72.CrossRefGoogle Scholar
Fama, E. F. & French, K. R. (1993) Common risk factors in the returns on stocks and bonds. Journal of Financial Economics 33, 3–56.CrossRefGoogle Scholar
Fan, J., Fan, Y., & Lv, J. (2008) High dimensional covariance matrix estimation using a factor model. Journal of Econometrics 147, 186–197.CrossRefGoogle Scholar
Fan, J., Liao, Y., & Liu, H. (2016) An overview of the estimation of large covariance and precision matrices. The Econometrics Journal 19, 1–32.CrossRefGoogle Scholar
Fan, J., Liao, Y., & Mincheva, M. (2011) High-dimensional covariance matrix estimation in approximate factor models. Annals of Statistics 39, 3320–3356.CrossRefGoogle ScholarPubMed
Fan, J., Liao, Y., & Mincheva, M. (2013) Large covariance estimation by thresholding principal orthogonal complements. Journal of the Royal Statistical Society: Series B (Statistical Methodology) 75, 603–680.CrossRefGoogle ScholarPubMed
Fan, J., Liao, Y., & Yao, J. (2015) Power enhancement in high-dimensional cross-sectional tests. Econometrica 83, 1497–1541.CrossRefGoogle ScholarPubMed
Filipiak, K., Klein, D., & Vojtkova, E. (2018) The properties of partial trace and block trace operators of partitioned matrices. Electronic Journal of Linear Algebra 33, 3–15.CrossRefGoogle Scholar
Hafner, C. M., Linton, O. B., & Tang, H. (2020) Estimation of a multiplicative correlation structure in the large dimensional case. Journal of Econometrics 217, 431–470.CrossRefGoogle Scholar
Hidalgo, J. & Schafgans, M. (2017) Inference Without Smoothing for Large Panels with Cross-Sectional and Temporal Dependence. Working Paper.Google Scholar
Hoff, P. D. (2011) Separable covariance arrays via the tucker product, with applications to multivariate relational data. Bayesian Analysis 6, 179–196.CrossRefGoogle Scholar
Hoff, P. D. (2015) Multilinear tensor regression for longitudinal relational data The Annals of Applied Statistics 9, 1169–1193.CrossRefGoogle ScholarPubMed
Hoff, P. D. (2016) Equivariant and scale-free tucker decomposition models Bayesian Analysis 11, 627–648.CrossRefGoogle Scholar
Kelejian, H. H. & Prucha, I. R. (2001) On the asymptotic distribution of the moran i test statistic with applications Journal of Econometrics 104, 219–257.CrossRefGoogle Scholar
Knopp, K. (1947) Theory and Application of Infinite Series. Blackie and Son.Google Scholar
Kroonenberg, P. M. (2008) Applied Multiway Data Analysis. Wiley.CrossRefGoogle Scholar
Ledoit, O. & Wolf, M. (2003) Improved estimation of the covariance matrix of stock returns with an application to portfolio selection. Journal of Empirical Finance 10, 603–621.CrossRefGoogle Scholar
Ledoit, O. & Wolf, M. (2004) A well-conditioned estimator for large-dimensional covariance matrices. Journal of Multivariate Analysis 88, 365–411.CrossRefGoogle Scholar
Ledoit, O. & Wolf, M. (2012) Nonlinear shrinkage estimation of large dimensional covariance matrices. Annals of Statistics 40, 1024–1060.CrossRefGoogle Scholar
Ledoit, O. & Wolf, M. (2015) Spectrum estimation: A unified framework for covariance matrix estimation and PCA in large dimensions. Journal of Multivariate Analysis 139, 360–384.CrossRefGoogle Scholar
Ledoit, O. & Wolf, M. (2017) Direct Nonlinear Shrinkage Estimation of Large-Dimensional Covariance Matrices. Working Paper.CrossRefGoogle Scholar
Loh, W.-L. & Lam, T.-K. (2000) Estimating structured correlation matrices in smooth Gaussian random field models. Annals of Statistics 28, 880–904.Google Scholar
Magnus, J. R. & Neudecker, H. (1986) Symmetry, 0-1 matrices and Jacobians a review. Econometric Theory 2, 157–190.CrossRefGoogle Scholar
Markowitz, H. (1952) Portfolio selection. The Journal of Finance 7, 77–91.Google Scholar
Park, J. Y. & Phillips, P. C. B. (1988) On the formulation of wald tests of nonlinear restrictions. Econometrica 56, 1065–1083.Google Scholar
Pesaran, M. H. and Yamagata, T. (2012) Testing CAPM with a Large Number of Assets. Working Paper.CrossRefGoogle Scholar
Phillips, P. C. B. & Moon, H. R. (1999) Linear regression limit theory for nonstationary panel data. Econometrica 67, 1057–1111.CrossRefGoogle Scholar
Robinson, P. M. (1998) Inference-without-smoothing in the presence of nonparametric autocorrelation. Econometrica 66, 1163–1182.CrossRefGoogle Scholar
Saikkonen, P. & Lutkepohl, H. (1996) Infinite-order cointegrated vector autoregressive processes. Econometric Theory 12, 814–844.CrossRefGoogle Scholar
Swain, A. J. (1975) Analysis of Parametric Structures for Variance Matrices. PhD Thesis, University of Adelaide.Google Scholar
Verhees, J. & Wansbeek, T. J. (1990) A multimode direct product model for covariance structure analysis. British Journal of Mathematical and Statistical Psychology 43, 231–240.CrossRefGoogle Scholar