Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-25T05:21:42.592Z Has data issue: false hasContentIssue false
  • English
  • Français

The volume of singular Kähler–Einstein Fano varieties

Part of: Local theory Complex manifolds Surfaces and higher-dimensional varieties Algebraic groups

Published online by Cambridge University Press:  29 April 2018

Yuchen Liu
Yuchen Liu*
Affiliation:
Department of Mathematics, Princeton University, Princeton, NJ, 08544-1000, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that the anti-canonical volume of an $n$-dimensional Kähler–Einstein 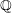$\mathbb{Q}$-Fano variety is bounded from above by certain invariants of the local singularities, namely 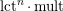$\operatorname{lct}^{n}\cdot \operatorname{mult}$ for ideals and the normalized volume function for real valuations. This refines a recent result by Fujita. As an application, we get sharp volume upper bounds for Kähler–Einstein Fano varieties with quotient singularities. Based on very recent results by Li and the author, we show that a Fano manifold is K-semistable if and only if a de Fernex–Ein–Mustaţă type inequality holds on its affine cone.

Keywords

Fano varietiessingularitiesK-stabilityKähler–Einstein metrics

MSC classification

Primary: 14J45: Fano varieties 14B05: Singularities
Secondary: 14L24: Geometric invariant theory 32Q15: Kähler manifolds
Type
Research Article
Information
Compositio Mathematica , Volume 154 , Issue 6 , June 2018 , pp. 1131 - 1158
Copyright
© The Author 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batyrev, V. V., Toric Fano threefolds , Izv. Akad. Nauk SSSR Ser. Mat. 45 (1981), 704–717; 927 (Russian).Google Scholar
Berman, R., K-polystability of ℚ-Fano varieties admitting Kähler–Einstein metrics , Invent. Math. 203 (2016), 973–1025.Google Scholar
Berman, R. and Berndtsson, B., The projective space has maximal volume among all toric Kähler–Einstein manifolds, Preprint (2011), arXiv:1112.4445.Google Scholar
Berman, R. and Berndtsson, B., The volume of Kähler–Einstein Fano varieties and convex bodies , J. Reine Angew. Math. 723 (2017), 127–152.Google Scholar
Berman, R., Boucksom, S. and Jonsson, M., A variational approach to the Yau–Tian–Donaldson conjecture, Preprint (2015), arXiv:1509.04561.Google Scholar
Berman, R., Boucksom, S., Eyssidieux, P., Guedj, V. and Zeriahi, A., Kähler–Einstein metrics and the Kähler–Ricci flow on log Fano varieties, J. Reine Angew. Math., to appear. Preprint (2011), arXiv:1111.7158.Google Scholar
Blum, H., Existence of valuations with smallest normalized volume , Compos. Math. 154 (2018), 820–849.CrossRefGoogle Scholar
Boucksom, S. and Chen, H., Okounkov bodies of filtered linear series , Compos. Math. 147 (2011), 1205–1229.CrossRefGoogle Scholar
Boucksom, S., de Fernex, T., Favre, C. and Urbinati, S., Valuation spaces and multiplier ideals on singular varieties , in Recent advances in algebraic geometry, London Mathematical Society Lecture Note Series, vol. 417 (Cambridge University Press, Cambridge, 2015), 29–51.Google Scholar
Boucksom, S., Favre, C. and Jonsson, M., A refinement of Izumi’s theorem , in Valuation theory in interaction, EMS Series of Congress Reports (European Mathematical Society, Zürich, 2014), 55–81.Google Scholar
Boucksom, S., Hisamoto, T. and Jonsson, M., Uniform K-stability, Duistermaat–Heckman measures and singularities of pairs , Ann. Inst. Fourier (Grenoble) 67 (2017), 743–841.Google Scholar
Boucksom, S., Küronya, A., Maclean, C. and Szemberg, T., Vanishing sequences and Okounkov bodies , Math. Ann. 361 (2015), 811–834.CrossRefGoogle Scholar
Campana, F., Connexité rationnelle des variétés de Fano , Ann. Sci. Éc. Norm. Supér. (4) 25 (1992), 539–545 (French).Google Scholar
Cheltsov, I., Log canonical thresholds of del Pezzo surfaces , Geom. Funct. Anal. 18 (2008), 1118–1144.Google Scholar
Cheltsov, I. and Kosta, D., Computing 𝛼-invariants of singular del Pezzo surfaces , J. Geom. Anal. 24 (2014), 798–842.Google Scholar
Cutkosky, S. D., Multiplicities associated to graded families of ideals , Algebra Number Theory 7 (2013), 2059–2083.Google Scholar
de Fernex, T., Ein, L. and Mustaţă, M., Bounds for log canonical thresholds with applications to birational rigidity , Math. Res. Lett. 10 (2003), 219–236.Google Scholar
de Fernex, T., Ein, L. and Mustaţă, M., Multiplicities and log canonical threshold , J. Algebraic Geom. 13 (2004), 603–615.Google Scholar
de Fernex, T., Ein, L. and Mustaţă, M., Log canonical thresholds on varieties with bounded singularities , in Classification of algebraic varieties, EMS Series of Congress Reports (European Mathematical Society, Zürich, 2011), 221–257.Google Scholar
de Fernex, T., Küronya, A. and Lazarsfeld, R., Higher cohomology of divisors on a projective variety , Math. Ann. 337 (2007), 443–455.Google Scholar
de Fernex, T. and Mustaţă, M., The volume of a set of arcs on a variety , Rev. Roumaine Math. Pures Appl. 60 (2015), 375–401.Google Scholar
Ding, W.-Y., Remarks on the existence problem of positive Kähler–Einstein metrics , Math. Ann. 282 (1988), 463–471.CrossRefGoogle Scholar
Ding, W. Y. and Tian, G., Kähler–Einstein metrics and the generalized Futaki invariant , Invent. Math. 110 (1992), 315–335.Google Scholar
Dolgachev, I., Weighted projective varieties , in Group actions and vector fields (Vancouver, B.C., 1981), Lecture Notes in Mathematics, vol. 956 (Springer, Berlin, 1982), 34–71.Google Scholar
Donaldson, S., Scalar curvature and stability of toric varieties , J. Differential Geom. 62 (2002), 289–349.CrossRefGoogle Scholar
Ein, L., Lazarsfeld, R. and Smith, K. E., Uniform approximation of Abhyankar valuation ideals in smooth function fields , Amer. J. Math. 125 (2003), 409–440.CrossRefGoogle Scholar
Fujita, K., Optimal bounds for the volumes of Kähler–Einstein Fano manifolds, Amer. J. Math., to appear. Preprint (2015), arXiv:1508.04578.Google Scholar
Fujita, K., A valuative criterion for uniform K-stability of ℚ-Fano varieties, J. Reine Angew. Math., to appear. Preprint (2016), arXiv:1602.00901.Google Scholar
Ghigi, A. and Kollár, J., Kähler–Einstein metrics on orbifolds and Einstein metrics on spheres , Comment. Math. Helv. 82 (2007), 877–902.CrossRefGoogle Scholar
Ishii, S., Extremal functions and prime blow-ups , Comm. Algebra 32 (2004), 819–827.Google Scholar
Izumi, S., A measure of integrity for local analytic algebras , Publ. Res. Inst. Math. Sci. 21 (1985), 719–735.Google Scholar
Jeffres, T. D., Singular set of some Kähler orbifolds , Trans. Amer. Math. Soc. 349 (1997), 1961–1971.CrossRefGoogle Scholar
Jonsson, M. and Mustaţă, M., Valuations and asymptotic invariants for sequences of ideals , Ann. Inst. Fourier (Grenoble) 62 (2012), 2145–2209.Google Scholar
Kollár, J., Shafarevich maps and automorphic forms, Porter Lectures (Princeton University Press, Princeton, NJ, 1995).Google Scholar
Kollár, J., Rational curves on algebraic varieties, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge/A Series of Modern Surveys in Mathematics, vol. 32 (Springer, Berlin, 1996).Google Scholar
Kollár, J., Miyaoka, Y. and Mori, S., Rational connectedness and boundedness of Fano manifolds , J. Differential Geom. 36 (1992), 765–779.CrossRefGoogle Scholar
Kollár, J. and Mori, S., Birational geometry of algebraic varieties, Cambridge Tracts in Mathematics, vol. 134 (Cambridge University Press, Cambridge, 1998).CrossRefGoogle Scholar
Lazarsfeld, R., Positivity in algebraic geometry. I. Classical setting: line bundles and linear series, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge/A Series of Modern Surveys in Mathematics, vol. 48 (Springer, Berlin, 2004).Google Scholar
Lazarsfeld, R. and Mustaţă, M., Convex bodies associated to linear series , Ann. Sci. Éc. Norm. Supér. (4) 42 (2009), 783–835.Google Scholar
Li, C., Minimizing normalized volumes of valuations, Math. Z., to appear. Preprint (2015),arXiv:1511.08164.Google Scholar
Li, C., K-semistability is equivariant volume minimization , Duke Math. J. 166 (2017), 3147–3218.Google Scholar
Li, C. and Liu, Y., Kähler–Einstein metrics and volume minimizations, Adv. Math., to appear. Preprint (2016), arXiv:1602.05094.Google Scholar
Li, C. and Xu, C., Special test configuration and K-stability of Fano varieties , Ann. of Math. (2) 180 (2014), 197–232.Google Scholar
Li, C. and Xu, C., Stability of valuations and Kollár components, Preprint (2016),arXiv:1604.05398.Google Scholar
Liu, Y. and Zhuang, Z., Characterization of projective spaces by Seshadri constants, Math. Z., to appear. Preprint (2016), arXiv:1607.05743.Google Scholar
Mabuchi, T. and Mukai, S., Stability and Einstein–Kähler metric of a quartic del Pezzo surface , in Einstein metrics and Yang-Mills connections (Sanda, 1990), Lecture Notes in Pure and Applied Mathematics, vol. 145 (Dekker, New York, 1993).Google Scholar
Molien, T., Über die Invarianten der linearen Substitutionsgruppe , Sitzungsber. König. Preuss. Akad. Wiss (1897), 1152–1156.Google Scholar
Mustaţă, M., On multiplicities of graded sequences of ideals , J. Algebra 256 (2002), 229–249.Google Scholar
Odaka, Y., Spotti, C. and Sun, S., Compact moduli spaces of Del Pezzo surfaces and Kähler–Einstein metrics , J. Differential Geom. 102 (2016), 127–172.Google Scholar
Ramanujam, C. P., On a geometric interpretation of multiplicity , Invent. Math. 22 (1973/74), 63–67.CrossRefGoogle Scholar
Rees, D., Izumi’s theorem , in Commutative algebra (Berkeley, CA, 1987), Mathematical Sciences Research Institute Publications, vol. 15 (Springer, New York, 1989), 407–416.Google Scholar
Shi, Y., On the 𝛼-invariants of cubic surfaces with Eckardt points , Adv. Math. 225 (2010), 1285–1307.Google Scholar
Tian, G., On Calabi’s conjecture for complex surfaces with positive first Chern class , Invent. Math. 101 (1990), 101–172.Google Scholar
Tian, G., Kähler–Einstein metrics with positive scalar curvature , Invent. Math. 130 (1997), 1–37.Google Scholar
Witt Nyström, D., Test configurations and Okounkov bodies , Compos. Math. 148 (2012), 1736–1756.Google Scholar
Won, J., Slope of a del Pezzo surface with du Val singularities , Bull. Lond. Math. Soc. 45 (2013), 402–410.Google Scholar