Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-13T11:32:58.125Z Has data issue: false hasContentIssue false
  • English
  • Français

Stability and the Fourier–Mukai transform II

Part of: Families, fibrations

Published online by Cambridge University Press:  01 January 2009

Kōta Yoshioka
Kōta Yoshioka*
Affiliation:
Department of Mathematics, Faculty of Science, Kobe University, Kobe, 657-8501, Japan (email: [email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

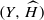We consider the problem of preservation of stability under the Fourier–Mukai transform ℱℰ:D(X)→D(Y ) on an abelian surface and a K3 surface. If Y is the moduli space of μ-stable sheaves on X with respect to a polarization H, we have a canonical polarization on Y and we have a correspondence between (X,H) and . We show that the stability with respect to these polarizations is preserved under ℱℰ, if the degree of stable sheaves on X is sufficiently large.

Keywords

moduli of stable sheavesFourier–Mukai functor

MSC classification

Secondary: 14D20: Algebraic moduli problems, moduli of vector bundles
Type
Research Article
Information
Compositio Mathematica , Volume 145 , Issue 1 , January 2009 , pp. 112 - 142
Copyright
Copyright © Foundation Compositio Mathematica 2009

References

[1]Bartocci, C., Bruzzo, U. and Hernández Ruipérez, D., A Fourier–Mukai transform for stable bundles on K3 surfaces, J. Reine Angew. Math. 486 (1997), 1–16.Google Scholar
[2]Bridgeland, T., Equivalences of triangulated categories and Fourier–Mukai transforms, Bull. London Math. Soc. 31 (1999), 25–34, math.AG/9809114.CrossRefGoogle Scholar
[3]Bridgeland, T., Stability conditions on K3 surfaces, Duke Math. J. 141 (2008), 241–291, math.AG/0307164.CrossRefGoogle Scholar
[4]Huybrechts, D., Derived and abelian equivalence of K3 surfaces, J. Algebraic Geom. 17 (2008), 375–400, math.AG/0604150.CrossRefGoogle Scholar
[5]Huybrechts, D. and Stellari, P., Equivalences of twisted K3 surfaces, Math. Ann. 332 (2005), 901–936, math.AG/0409030.CrossRefGoogle Scholar
[6]Mukai, S., Semi-homogeneous vector bundles on an Abelian variety, J. Math. Kyoto Univ. 18 (1978), 239–272.Google Scholar
[7]Mukai, S., On the moduli space of bundles on K3 surfaces. I, in Vector Bundles on Algebraic Varieties (Bombay, 1984), Tata Inst. Fund. Res. Stud. Math., vol. 11 (Tata Inst. Fund. Res., Bombay, 1987), 341–413.Google Scholar
[8]Orlov, D., Equivalences of derived categories and K3 surfaces, Algebraic geometry, 7. J. Math. Sci. (New York) 84 (1997), 1361–1381, alg-geom/9606006.CrossRefGoogle Scholar
[9]Onishi, N. and Yoshioka, K., Singularities on the 2-dimensional moduli spaces of stable sheaves on K3 surfaces, Internat. J. Math. 14 (2003), 837–864, math.AG/0208241.CrossRefGoogle Scholar
[10]Terakawa, H., The k-very ampleness and k-spannedness on polarized abelian surfaces, Math. Nachr. 195 (1998), 237–250.CrossRefGoogle Scholar
[11]Yoshioka, K., Some examples of Mukai’s reflections on K3 surfaces, J. Reine Angew. Math. 515 (1999), 97–123.CrossRefGoogle Scholar
[12]Yoshioka, K., Irreducibility of moduli spaces of vector bundles on K3 surfaces, math.AG/9907001.Google Scholar
[13]Yoshioka, K., Moduli spaces of stable sheaves on abelian surfaces, Math. Ann. 321 (2001), 817–884, math.AG/0009001.CrossRefGoogle Scholar
[14]Yoshioka, K., A note on Fourier–Mukai transform, math.AG/0112267 v. 2.Google Scholar
[15]Yoshioka, K., Twisted stability and Fourier–Mukai transform, part I, Compositio Math. 138 (2003), 261–288, math.AG/0106118; part II: Manuscripta Math. 110 (2003), 433–465.CrossRefGoogle Scholar
[16]Yoshioka, K., Stability and the Fourier–Mukai transform I, Math. Z. 245 (2003), 657–665.CrossRefGoogle Scholar
[17]Yoshioka, K., Moduli of twisted sheaves on a projective variety, Adv. Stud. Pure Math. 45 (2006), 1–30, math.AG/0411538.Google Scholar