Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-14T13:22:32.737Z Has data issue: false hasContentIssue false
  • English
  • Français

Some non-finitely generated Cox rings

Part of: Curves Special varieties Birational geometry

Published online by Cambridge University Press:  22 December 2015

José Luis González  and
Kalle Karu
José Luis González
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC V6T 1Z2, Canada email [email protected] Current address:Department of Mathematics, Yale University, New Haven, CT 06511, USA
Kalle Karu
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC V6T 1Z2, Canada email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

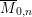We give a large family of weighted projective planes, blown up at a smooth point, that do not have finitely generated Cox rings. We then use the method of Castravet and Tevelev to prove that the moduli space $\overline{M}_{0,n}$ of stable $n$-pointed genus-zero curves does not have a finitely generated Cox ring if $n$ is at least $13$.

Keywords

Cox ringsmoduli spaces of curvesMori dream spacesweighted projective planes

MSC classification

Primary: 14M25: Toric varieties, Newton polyhedra 14E30: Minimal model program (Mori theory, extremal rays) 14H10: Families, moduli (algebraic)
Type
Research Article
Information
Compositio Mathematica , Volume 152 , Issue 5 , May 2016 , pp. 984 - 996
Copyright
© The Authors 2015 

References

Birkar, C., Cascini, P., Hacon, C. and McKernan, J., Existence of minimal models for varieties of log general type, J. Amer. Math. Soc. 23 (2010), 405–468.Google Scholar
Castravet, A.-M., The Cox ring of M0, 6, Trans. Amer. Math. Soc. 361 (2009), 3851–3878.Google Scholar
Castravet, A.-M. and Tevelev, J., M0, n is not a Mori dream space, Duke Math. J. 164 (2015), 1641–1667; MR 3352043.CrossRefGoogle Scholar
Cutkosky, S. D., Symbolic algebras of monomial primes, J. Reine Angew. Math. 416 (1991), 71–89; MR 1099946 (92b:13009).Google Scholar
Fulton, W., Introduction to toric varieties, Annals of Mathematics Studies, vol. 131 (Princeton University Press, Princeton, NJ, 1993); MR 1234037 (94g:14028).Google Scholar
Goto, S., Nishida, K. and Watanabe, K., Non-Cohen–Macaulay symbolic blow-ups for space monomial curves and counterexamples to Cowsik’s question, Proc. Amer. Math. Soc. 120 (1994), 383–392; MR 1163334 (94d:13005).Google Scholar
Hu., Y. and Keel, S., Mori dream spaces and GIT, Michigan Math. J. 48 (2000), 331–348; Dedicated to William Fulton on the occasion of his 60th birthday; MR 1786494 (2001i:14059).CrossRefGoogle Scholar
Okawa, S., On images of Mori dream spaces, Math. Ann. (2015), doi:10.1007/s00208-015-1245-5.Google Scholar