Article contents
Rational points on hyperelliptic curves having a marked non-Weierstrass point
Published online by Cambridge University Press: 09 October 2017
Abstract
In this paper, we consider the family of hyperelliptic curves over 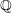 $\mathbb{Q}$ having a fixed genus
$\mathbb{Q}$ having a fixed genus  $n$ and a marked rational non-Weierstrass point. We show that when
$n$ and a marked rational non-Weierstrass point. We show that when 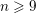 $n\geqslant 9$, a positive proportion of these curves have exactly two rational points, and that this proportion tends to one as
$n\geqslant 9$, a positive proportion of these curves have exactly two rational points, and that this proportion tends to one as  $n$ tends to infinity. We study rational points on these curves by first obtaining results on the 2-Selmer groups of their Jacobians. In this direction, we prove that the average size of the 2-Selmer groups of the Jacobians of curves in our family is bounded above by 6, which implies a bound of
$n$ tends to infinity. We study rational points on these curves by first obtaining results on the 2-Selmer groups of their Jacobians. In this direction, we prove that the average size of the 2-Selmer groups of the Jacobians of curves in our family is bounded above by 6, which implies a bound of 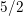 $5/2$ on the average rank of these Jacobians. Our results are natural extensions of Poonen and Stoll [Most odd degree hyperelliptic curves have only one rational point, Ann. of Math. (2) 180 (2014), 1137–1166] and Bhargava and Gross [The average size of the 2-Selmer group of Jacobians of hyperelliptic curves having a rational Weierstrass point, in Automorphic representations and
$5/2$ on the average rank of these Jacobians. Our results are natural extensions of Poonen and Stoll [Most odd degree hyperelliptic curves have only one rational point, Ann. of Math. (2) 180 (2014), 1137–1166] and Bhargava and Gross [The average size of the 2-Selmer group of Jacobians of hyperelliptic curves having a rational Weierstrass point, in Automorphic representations and $L$-functions, Tata Inst. Fundam. Res. Stud. Math., vol. 22 (Tata Institute of Fundamental Research, Mumbai, 2013), 23–91], where the analogous results are proved for the family of hyperelliptic curves with a marked rational Weierstrass point.
$L$-functions, Tata Inst. Fundam. Res. Stud. Math., vol. 22 (Tata Institute of Fundamental Research, Mumbai, 2013), 23–91], where the analogous results are proved for the family of hyperelliptic curves with a marked rational Weierstrass point.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Authors 2017
References
- 13
- Cited by


