Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-24T10:31:15.291Z Has data issue: false hasContentIssue false
  • English
  • Français

Random triangular groups at density $1/3$

Part of: Graph theory Probabilistic methods in group theory Special aspects of infinite or finite groups

Published online by Cambridge University Press:  27 November 2014

Sylwia Antoniuk ,
Tomasz Łuczak  and
Jacek Świa̧tkowski
Sylwia Antoniuk
Affiliation:
Adam Mickiewicz University, Faculty of Mathematics and Computer Science, ul. Umultowska 87, 61-614 Poznań, Poland email [email protected]
Tomasz Łuczak
Affiliation:
Adam Mickiewicz University, Faculty of Mathematics and Computer Science, ul. Umultowska 87, 61-614 Poznań, Poland email [email protected]
Jacek Świa̧tkowski
Affiliation:
Instytut Matematyczny, Uniwersytet Wrocławski, pl. Grunwaldzki 2/4, 50-384 Wrocław, Poland email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

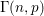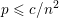Let ${\rm\Gamma}(n,p)$ denote the binomial model of a random triangular group. We show that there exist constants $c,C>0$ such that if $p\leqslant c/n^{2}$, then asymptotically almost surely (a.a.s.) 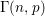${\rm\Gamma}(n,p)$ is free, and if 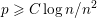$p\geqslant C\log n/n^{2}$, then a.a.s. ${\rm\Gamma}(n,p)$ has Kazhdan’s property (T). Furthermore, we show that there exist constants 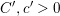$C^{\prime },c^{\prime }>0$ such that if 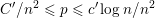$C^{\prime }/n^{2}\leqslant p\leqslant c^{\prime }\log n/n^{2}$, then a.a.s. 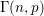${\rm\Gamma}(n,p)$ is neither free nor has Kazhdan’s property (T).

Keywords

random groupKazhdan’s propertyspectral gaprandom graph

MSC classification

Primary: 20P05: Probabilistic methods in group theory
Secondary: 20F05: Generators, relations, and presentations 05C80: Random graphs
Type
Research Article
Information
Compositio Mathematica , Volume 151 , Issue 1 , January 2015 , pp. 167 - 178
Copyright
© The Author(s) 2014 

References

Bollobás, B., Random graphs (Cambridge University Press, Cambridge, 2001).CrossRefGoogle Scholar
Coja-Oghlan, A., On the Laplacian eigenvalues of G (n, p), Combin. Probab. Comput. 16 (2007), 923–946.Google Scholar
Courant, R., Über die Eigenwerte bei den Differentialgleichungen der mathematischen Physik, Math. Z. 7 (1920), 1–57.CrossRefGoogle Scholar
Fischer, E., Über quadratische Formen mit reellen Koeffizienten, Monatsh. Math. Phys. 16 (1905), 234–249.Google Scholar
Janson, S., Łuczak, T. and Ruciński, A., Random graphs (Wiley, New York, 2000).Google Scholar
Kotowski, M. and Kotowski, M., Random groups and property (T): Żuk’s theorem revisited, J. Lond. Math. Soc. (2) 88 (2013), 396–416.Google Scholar
Ollivier, Y., Sharp phase transition theorems for hyperbolicity of random groups, Geom. Funct. Anal. 14 (2004), 595–679.CrossRefGoogle Scholar
Ollivier, Y., A January 2005 invitation to random groups, Ensaios Matemáticos [Mathematical Surveys], vol. 10 (Sociedade Brasileira de Matemática, Rio de Janeiro, 2005).Google Scholar
Ollivier, Y., Some small cancellation properties of random groups, Internat. J. Algebra Comput. 17 (2007), 37–51.Google Scholar
Schmidt-Pruzan, J. and Shamir, E., Component structure in the evolution of random hypergraphs, Combinatorica 5 (1985), 81–94.CrossRefGoogle Scholar
Żuk, A., Property (T) and Kazhdan constants for discrete groups, Geom. Funct. Anal. 13 (2003), 643–670.Google Scholar