Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-14T01:12:39.419Z Has data issue: false hasContentIssue false
  • English
  • Français

Poincaré–Lelong equation via the Hodge–Laplace heat equation

Part of: Parabolic equations and systems Partial differential equations Complex manifolds Global differential geometry

Published online by Cambridge University Press:  09 September 2013

Lei Ni  and
Luen-Fai Tam
Lei Ni
Affiliation:
Department of Mathematics, University of California at San Diego, La Jolla, CA 92093, USA email [email protected]
Luen-Fai Tam
Affiliation:
The Institute of Mathematical Sciences and Department of Mathematics, The Chinese University of Hong Kong, Shatin, Hong Kong, China email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

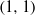In this paper, we develop a method of solving the Poincaré–Lelong equation, mainly via the study of the large time asymptotics of a global solution to the Hodge–Laplace heat equation on $(1, 1)$-forms. The method is effective in proving an optimal result when 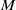$M$ has nonnegative bisectional curvature. It also provides an alternate proof of a recent gap theorem of the first author.

Keywords

Poincaré–Lelong equationHodge-Laplacian heat equationKähler manifoldsConvex exhaustion

MSC classification

Secondary: 53C21: Methods of Riemannian geometry, including PDE methods; curvature restrictions 32Q15: Kähler manifolds 35B40: Asymptotic behavior of solutions 35K05: Heat equation
Type
Research Article
Information
Compositio Mathematica , Volume 149 , Issue 11 , November 2013 , pp. 1856 - 1870
Copyright
© The Author(s) 2013 

References

Evans, L., Partial differential equations, Graduate Studies in Mathematics, vol. 19, second edition (American Mathematical Society, Providence, RI, 2010).Google Scholar
Fan, X.-Q., On the existence of solutions of Poisson equation and Poincaré–Lelong equation, Manuscripta Math. 120 (2006), 435–467.Google Scholar
Goldberg, S. I. and Kobayashi, S., Holomorphic bisectional curvature, J. Differential Geom. 1 (1967), 225–233.Google Scholar
Ladyenskaja, O. A., Solonnikov, V. A. and Ural’ceva, N. N., Linear and quasi-linear equations of parabolic type, Translations of Mathematical Monographs, vol. 23 (American Mathematical Society, Providence, RI, 1967).Google Scholar
Li, P. and Tam, L.-F., The heat equation and harmonic maps of complete manifolds, Invent. Math. 105 (1991), 1–46.Google Scholar
Lichnerowicz, A., Geometry of groups of transformations, ed. Cole, M. (Noordhoff International, Leyden, 1977).Google Scholar
Mok, N., Siu, Y.-T. and Yau, S.-T., The Poincaré–Lelong equation on complete Kähler manifolds, Compositio Math. 44 (1981), 183–218.Google Scholar
Morrey, C. B., Multiple integrals in the calculus of variations, Die Grundlehren der mathematischen Wissenschaften, vol. 130 (Springer, New York, NY, 1966).Google Scholar
Ni, L., Vanishing theorems on complete Kähler manifolds and their applications, J. Differential Geom. 50 (1998), 89–122.Google Scholar
Ni, L., The Poisson equation and Hermitian–Einstein metrics on holomorphic vector bundles over complete noncompact Kähler manifolds., Indiana Univ. Math. J. 51 (2002), 679–704.Google Scholar
Ni, L., A monotonicity formula on complete Kähler manifolds with nonnegative bisectional curvature, J. Amer. Math. Soc. 17 (2004), 909–946.Google Scholar
Ni, L., An optimal gap theorem, Invent. Math. 189 (2012), 737–761; (Erratum to appear, also available at http://math.ucsd.edu/~lni/academic/addendum-2.pdf).Google Scholar
Ni, L. and Niu, Y. Y., Sharp differential estimates of Li–Yau–Hamilton type for positive $(p, p)$-forms on Kähler manifolds, Comm. Pure Appl. Math. 64 (2011), 920–974.Google Scholar
Ni, L., Shi, Y.-G. and Tam, L.-F., Poisson equation, Poincaré–Lelong equation and curvature decay on complete Kähler manifolds, J. Differential Geom. 57 (2001), 339–388.Google Scholar
Ni, L. and Tam, L.-F., Plurisubharmonic functions and the structure of complete Kähler manifolds with nonnegative curvature, J. Differential Geom. 64 (2003), 457–524.Google Scholar
Wilking, B., A Lie algebraic approach to Ricci flow invariant curvature condition and Harnack inequalities, J. Reine Angew. Math. 679 (2013), 223–247.Google Scholar