Article contents
A partial converse to the Andreotti–Grauert theorem
Published online by Cambridge University Press: 19 November 2018
Abstract
Let 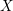 $X$ be a smooth projective manifold with
$X$ be a smooth projective manifold with 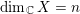 $\dim _{\mathbb{C}}X=n$. We show that if a line bundle
$\dim _{\mathbb{C}}X=n$. We show that if a line bundle 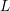 $L$ is
$L$ is 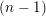 $(n-1)$-ample, then it is
$(n-1)$-ample, then it is 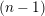 $(n-1)$-positive. This is a partial converse to the Andreotti–Grauert theorem. As an application, we show that a projective manifold
$(n-1)$-positive. This is a partial converse to the Andreotti–Grauert theorem. As an application, we show that a projective manifold 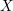 $X$ is uniruled if and only if there exists a Hermitian metric
$X$ is uniruled if and only if there exists a Hermitian metric 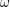 $\unicode[STIX]{x1D714}$ on
$\unicode[STIX]{x1D714}$ on 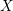 $X$ such that its Ricci curvature
$X$ such that its Ricci curvature 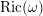 $\text{Ric}(\unicode[STIX]{x1D714})$ has at least one positive eigenvalue everywhere.
$\text{Ric}(\unicode[STIX]{x1D714})$ has at least one positive eigenvalue everywhere.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author 2018
References
- 8
- Cited by


