Article contents
Nonabelian Hodge theory for klt spaces and descent theorems for vector bundles
Published online by Cambridge University Press: 07 February 2019
Abstract
We generalise Simpson’s nonabelian Hodge correspondence to the context of projective varieties with Kawamata log terminal (klt) singularities. The proof relies on a descent theorem for numerically flat vector bundles along birational morphisms. In its simplest form, this theorem asserts that given any klt variety  $X$ and any resolution of singularities, any vector bundle on the resolution that appears to come from
$X$ and any resolution of singularities, any vector bundle on the resolution that appears to come from  $X$ numerically, does indeed come from
$X$ numerically, does indeed come from 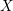 $X$. Furthermore, and of independent interest, a new restriction theorem for semistable Higgs sheaves defined on the smooth locus of a normal, projective variety is established.
$X$. Furthermore, and of independent interest, a new restriction theorem for semistable Higgs sheaves defined on the smooth locus of a normal, projective variety is established.
- Type
- Research Article
- Information
- Copyright
- © The Authors 2019
Footnotes
DG was partially supported by the DFG-Collaborative Research Center SFB/TR 45. SK gratefully acknowledges support through a joint fellowship of the Freiburg Institute of Advanced Studies (FRIAS) and the University of Strasbourg Institute for Advanced Study (USIAS). BT was partially supported by the DFG-Research Training Group GK1821. Research was partially completed while SK and TP were visiting the National University of Singapore in 2017. TP was supported by the DFG project ‘Zur Positivität in der komplexen Geometrie’.
References
- 9
- Cited by


