Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T04:19:22.784Z Has data issue: false hasContentIssue false
  • English
  • Français

Monoidal categorification and quantum affine algebras

Part of: Categories with structure Arithmetic rings and other special rings Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  27 April 2020

Masaki Kashiwara ,
Myungho Kim ,
Se-jin Oh  and
Euiyong Park
Masaki Kashiwara
Affiliation:
Kyoto University Institute for Advanced Study, Research Institute for Mathematical Sciences, Kyoto University, Kyoto 606-8502, Japan Korea Institute for Advanced Study, Seoul 02455, Korea email [email protected]
Myungho Kim
Affiliation:
Department of Mathematics, Kyung Hee University, Seoul 02447, Korea email [email protected]
Se-jin Oh
Affiliation:
Department of Mathematics, Ewha Womans University, Seoul 03760, Korea email [email protected]
Euiyong Park
Affiliation:
Department of Mathematics, University of Seoul, Seoul 02504, Korea email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

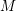We introduce and investigate new invariants of pairs of modules $M$ and 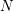$N$ over quantum affine algebras $U_{q}^{\prime }(\mathfrak{g})$ by analyzing their associated 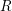$R$-matrices. Using these new invariants, we provide a criterion for a monoidal category of finite-dimensional integrable 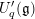$U_{q}^{\prime }(\mathfrak{g})$-modules to become a monoidal categorification of a cluster algebra.

Keywords

quantum affine algebramonoidal categorificationR-matricescluster algebra

MSC classification

Primary: 17B37: Quantum groups (quantized enveloping algebras) and related deformations 13F60: Cluster algebras 18D10: Monoidal categories (= multiplicative categories), symmetric monoidal categories, braided categories
Type
Research Article
Information
Compositio Mathematica , Volume 156 , Issue 5 , May 2020 , pp. 1039 - 1077
Copyright
© The Authors 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The research of M. Kashiwara was supported by Grant-in-Aid for Scientific Research (B) 15H03608, Japan Society for the Promotion of Science. The research of M. Kim was supported by the National Research Foundation of Korea (NRF) Grant funded by the Korea government (MSIP) (NRF-2017R1C1B2007824). The research of S.-j. Oh was supported by the Ministry of Education of the Republic of Korea and the National Research Foundation of Korea (NRF-2019R1A2C4069647). The research of E. Park was supported by the National Research Foundation of Korea (NRF) Grant funded by the Korea Government (MSIP) (NRF-2017R1A1A1A05001058).

References

Akasaka, T. and Kashiwara, M., Finite-dimensional representations of quantum affine algebras, Publ. RIMS Kyoto Univ. 33 (1997), 839–867.CrossRefGoogle Scholar
Berenstein, A. and Zelevinsky, A., Quantum cluster algebras, Adv. Math. 195 (2005), 405–455.CrossRefGoogle Scholar
Buan, A. B. and Marsh, R., Cluster-tilting theory, in Trends in representation theory of algebras and related topics, Contemporary Mathematics, vol. 406 (American Mathematical Society, Providence, RI, 2006), 1–30.Google Scholar
Cautis, S. and Williams, H., Cluster theory of the coherent Satake category, J. Amer. Math. Soc. 32 (2019), 709–778.CrossRefGoogle Scholar
Chari, V., Braid group actions and tensor products, Int. Math. Res. Not. IMRN 2002 (2010), 357–382.10.1155/S107379280210612XCrossRefGoogle Scholar
Chari, V. and Pressley, A., Quantum affine algebras, Commun. Math. Phys. 142 (1991), 261–283.CrossRefGoogle Scholar
Chari, V. and Pressley, A., A guide to quantum groups (Cambridge University Press, 1994).Google Scholar
Date, E. and Okado, M., Calculation of excitation spectra of the spin model related with the vector representation of the quantized affine algebra of type A (1)n, Int. J. Modern Phys. A 9 (1994), 399–417.CrossRefGoogle Scholar
Fock, V. V. and Goncharov, A. B., Cluster X-varieties, amalgamation, and Poisson–Lie groups, in Algebraic geometry and number theory, Progress in Mathematics, vol. 253 (Birkhäuser, Boston, MA, 2006), 27–68.CrossRefGoogle Scholar
Fomin, S. and Reading, N., Generalized cluster complexes and Coxeter combinatorics, Int. Math. Res. Not. IMRN (2005), 2709–2757.CrossRefGoogle Scholar
Fomin, S. and Zelevinsky, A., Cluster algebras I. Foundations, J. Amer. Math. Soc. 15 (2002), 497–529.10.1090/S0894-0347-01-00385-XCrossRefGoogle Scholar
Fomin, S. and Zelevinsky, A., Y-systems and generalized associahedra, Ann. of Math. (2) 158 (2003), 977–1018.CrossRefGoogle Scholar
Frenkel, E. and Hernandez, D., Baxter’s relations and spectra of quantum integrable models, Duke Math. J. 164 (2015), 2407–2460.CrossRefGoogle Scholar
Frenkel, I. and Reshetikhin, N., Quantum affine algebras and holonomic difference equations, Commun. Math. Phys. 146 (1992), 1–60.CrossRefGoogle Scholar
Frenkel, E. and Reshetikhin, N. Yu., The q-characters of representations of quantum affine algebras deformations of W-algebras, Recent developments in quantum affine algebras and related topics, Contemp. Math. 248 (1999), 163–205.CrossRefGoogle Scholar
Fujita, R., Geometric realization of Dynkin quiver type quantum affine Schur–Weyl duality, Int. Math. Res. Not. IMRN (2018), rny226.Google Scholar
Geiß, C., Leclerc, B. and Schröer, J., Cluster structures on quantum coordinate rings, Selecta Math. (N.S.) 19 (2013), 337–397.CrossRefGoogle Scholar
Geiß, C., Leclerc, B. and Schröer, J., Factorial cluster algebras, Doc. Math. 18 (2013), 249–274.Google Scholar
Gekhtman, M., Shapiro, M. and Vainshtein, A., Cluster algebras and Poisson geometry, Mosc. Math. J. 3 (2003), 899–934.CrossRefGoogle Scholar
Ginzburg, V. and Vasserot, E., Langlands reciprocity for affine quantum groups of type A n, Int. Math. Res. Not. IMRN 3 (1993), 67–85.CrossRefGoogle Scholar
Goodearl, K. R. and Yakimov, M. T., The Berenstein–Zelevinsky quantum cluster algebra conjecture, Preprint (2016), arXiv:1602.00498.Google Scholar
Hernandez, D., Simple tensor products, Invent. Math. 181 (2010), 649–675.CrossRefGoogle Scholar
Hernandez, D. and Leclerc, B., Cluster algebras and quantum affine algebras, Duke Math. J. 154 (2010), 265–341.CrossRefGoogle Scholar
Hernandez, D. and Leclerc, B., Monoidal categorifications of cluster algebras of type A and D, in Symmetries, integrable systems and representations, Springer Proceedings in Mathematics and Statistics, vol. 40 (Springer, 2013), 175–193.CrossRefGoogle Scholar
Hernandez, D. and Leclerc, B., A cluster algebra approach to q-characters of Kirillov–Reshetikhin modules, J. Eur. Math. Soc. (JEMS) 18 (2016), 1113–1159.CrossRefGoogle Scholar
Kac, V., Infinite dimensional Lie algebras, third edition (Cambridge University Press, Cambridge, 1990).CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M. and Kim, M., Symmetric quiver Hecke algebras and R-matrices of quantum affine algebras II, Duke Math. J. 164 (2015), 1549–1602.CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M. and Kim, M., Symmetric quiver Hecke algebras and R-matrices of quantum affine algebras, Invent. Math. 211 (2018), 591–685.CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M., Kim, M. and Oh, S.-j., Simplicity of heads and socles of tensor products, Compos. Math. 151 (2015), 377–396.CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M., Kim, M. and Oh, S.-j., Symmetric quiver Hecke algebras and R-matrices of quantum affine algebras III, Proc. Lond. Math. Soc. (3) 111 (2015), 420–444.CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M., Kim, M. and Oh, S.-j., Monoidal categorification of cluster algebras, J. Amer. Math. Soc. 31 (2018), 349–426.CrossRefGoogle Scholar
Kang, S.-J., Kashiwara, M., Misra, K. C., Miwa, T., Nakashima, T. and Nakayashiki, A., Perfect crystals of quantum affine Lie algebras, Duke Math. J. 68 (1992), 499–607.CrossRefGoogle Scholar
Kashiwara, M., Bases cristallines, C. R. Acad. Sci, Paris Sér. I, Math. 311 (1990), 277–280.Google Scholar
Kashiwara, M., On level zero representations of quantum affine algebras, Duke. Math. J. 112 (2002), 117–175.CrossRefGoogle Scholar
Kashiwara, M. and Kim, M., Laurent phenomenon and simple modules of quiver Hecke algebras, Compos. Math. 155 (2019), 2263–2295.CrossRefGoogle Scholar
Kashiwara, M., Kim, M. and Oh, S.-j., Monoidal categories of modules over quantum affine algebras of type A and B, Proc. Lond. Math. Soc. (3) 118 (2019), 43–77.CrossRefGoogle Scholar
Kashiwara, M., Kim, M., Oh, S.-j. and Park, E., Localizations for quiver Hecke algebras, Preprint (2019), arXiv:1901.09319.Google Scholar
Kashiwara, M., Kim, M., Oh, S.-j. and Park, E., Cluster algebra structures on module categories over quantum affine algebras, Preprint (2019), arXiv:1904.01264.Google Scholar
Kashiwara, M., Misra, K. C., Okado, M. and Yamada, D., Perfect crystals for U q(D 4(3)), J. Algebra 317 (2007), 392–423.CrossRefGoogle Scholar
Kashiwara, M. and Oh, S.-j., Categorical relations between Langlands dual quantum affine algebras: doubly laced types, J. Algebraic Combin. 49 (2019), 401–435.CrossRefGoogle Scholar
Kazhdan, D. and Soibelman, Y., Representations of quantum affine algebras, Selecta Math. (N.S.) 1 (1995), 537–595.CrossRefGoogle Scholar
Khovanov, M. and Lauda, A., A diagrammatic approach to categorification of quantum groups I, Represent. Theory 13 (2009), 309–347.CrossRefGoogle Scholar
Khovanov, M. and Lauda, A., A diagrammatic approach to categorification of quantum groups II, Trans. Amer. Math. Soc. 363 (2011), 2685–2700.CrossRefGoogle Scholar
Leclerc, B., Imaginary vectors in the dual canonical basis of U q(n), Transform. Groups 8 (2003), 95–104.CrossRefGoogle Scholar
Lee, K. and Schiffler, R., Positivity for cluster algebras, Ann. of Math. (2) 182 (2015), 73–125.CrossRefGoogle Scholar
Lusztig, G., Canonical bases arising from quantized enveloping algebras, J. Amer. Math. Soc. 3 (1990), 447–498.CrossRefGoogle Scholar
Nakajima, H., Quiver varieties and finite-dimensional representations of quantum affine algebras, J. Amer. Math. Soc. 14 (2001), 145–238.CrossRefGoogle Scholar
Nakajima, H., Quiver varieties and cluster algebras, Kyoto J. Math. 51 (2011), 71–126.CrossRefGoogle Scholar
Oh, S.-j., The denominators of normalized R-matrices of types A 2n-1(2) , A 2n(2) , B n(1) and D n+1(2), Publ. RIMS Kyoto Univ. 51 (2015), 709–744.CrossRefGoogle Scholar
Oh, S.-j. and Scrimshaw, T., Categorical relations between Langlands dual quantum affine algebras: exceptional cases, Comm. Math. Phys. 368 (2019), 295–367.CrossRefGoogle Scholar
Qin, F., Triangular bases in quantum cluster algebras and monoidal categorification conjectures, Duke Math. J. 166 (2017), 2337–2442.CrossRefGoogle Scholar
Rouquier, R., 2-Kac–Moody algebras, Preprint (2008), arXiv:0812.5023v1.Google Scholar
Rouquier, R., Quiver Hecke algebras and 2-Lie algebras, Algebra Colloq. 19 (2012), 359–410.CrossRefGoogle Scholar
Yamane, S., Perfect crystals of U q(G 2(1)), J. Algebra 210 (1998), 440–486.CrossRefGoogle Scholar