Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T12:12:31.022Z Has data issue: false hasContentIssue false
  • English
  • Français

Modular Koszul duality

Part of: Rings and algebras arising under various constructions Special varieties Linear algebraic groups and related topics

Published online by Cambridge University Press:  13 December 2013

Simon Riche ,
Wolfgang Soergel  and
Geordie Williamson
Simon Riche
Affiliation:
Clermont Université, Université Blaise Pascal, Laboratoire de Mathématiques, BP 10448, F-63000 Clermont-Ferrand, France email [email protected] CNRS, UMR 6620, Laboratoire de Mathématiques, F-63177 Aubière, France email [email protected]
Wolfgang Soergel
Affiliation:
Mathematisches Institut, Universität Freiburg, Eckerstraße 1, D-79104 Freiburg, Germany email [email protected]
Geordie Williamson
Affiliation:
Max-Planck-Institut für Mathematik, Vivatsgasse 7, 53111, Bonn, Germany email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

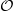We prove an analogue of Koszul duality for category $ \mathcal{O} $ of a reductive group $G$ in positive characteristic 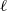$\ell $ larger than 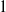$1$ plus the number of roots of 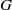$G$. However, there are no Koszul rings, and we do not prove an analogue of the Kazhdan–Lusztig conjectures in this context. The main technical result is the formality of the dg-algebra of extensions of parity sheaves on the flag variety if the characteristic of the coefficients is at least the number of roots of 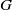$G$ plus 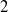$2$.

Keywords

Koszul dualitycategory $ \mathcal{O} $formalityparity sheavesperverse sheaves

MSC classification

Secondary: 14M15: Grassmannians, Schubert varieties, flag manifolds 20G05: Representation theory 16S37: Quadratic and Koszul algebras
Type
Research Article
Information
Compositio Mathematica , Volume 150 , Issue 2 , February 2014 , pp. 273 - 332
Copyright
© The Author(s) 2013 

References

Andersen, H. H., Jantzen, J. C. and Soergel, W., Representations of quantum groups at a pth root of unity and of semisimple groups in characteristic $p$ : independence of $p$ , Astérisque 220 (1994), 321.Google Scholar
Bass, H., Algebraic K-theory (W. A. Benjamin, Inc, New York–Amsterdam, 1968).Google Scholar
Beĭlinson, A. A., On the derived category of perverse sheaves, in K-theory, arithmetic and geometry (Moscow, 1984–1986), Lecture Notes in Mathematics, vol. 1289 (Springer, Berlin, 1987), 27–41.Google Scholar
Beĭlinson, A., Bernstein, J. and Deligne, P., Faisceaux pervers, in Analyse et topologie sur les espaces singuliers, I (Luminy, 1981), Astérisque, vol. 100 (Société Mathématique de France, Paris, 1982), 5–171.Google Scholar
Beĭlinson, A., Ginzburg, V. and Soergel, W., Koszul duality patterns in representation theory, J. Amer. Math. Soc. 9 (1996), 473–527.Google Scholar
Bernstein, J. and Lunts, V., Equivariant sheaves and functors, Lecture Notes in Mathematics, vol. 1578 (Springer, Berlin, 1994).CrossRefGoogle Scholar
Curtis, C. W., A further refinement of the Bruhat decomposition, Proc. Amer. Math. Soc. 102 (1988), 37–42.CrossRefGoogle Scholar
Deligne, P., La conjecture de Weil. I, Publ. Math. Inst. Hautes Études Sci. 43 (1974), 273–307.Google Scholar
Deligne, P., La conjecture de Weil. II, Publ. Math. Inst. Hautes Études Sci. 52 (1980), 137–252.Google Scholar
Deodhar, V. V., On some geometric aspects of Bruhat orderings. I. A finer decomposition of Bruhat cells, Invent. Math. 79 (1985), 499–511.CrossRefGoogle Scholar
Deligne, P., Griffiths, P., Morgan, J. and Sullivan, D., Real homotopy theory of Kähler manifolds, Invent. Math. 29 (1975), 245–274.CrossRefGoogle Scholar
Feit, W., The representation theory of finite groups, North-Holland Mathematical Library, vol. 25 (North-Holland Publishing Co, Amsterdam, 1982).Google Scholar
Fiebig, P., Sheaves on affine Schubert varieties, modular representations, and Lusztig’s conjecture, J. Amer. Math. Soc. 24 (2011), 133–181.CrossRefGoogle Scholar
Fiebig, P., An upper bound on the exceptional characteristics for Lusztig’s character formula, J. Reine Angew. Math. 673 (2012), 1–31.Google Scholar
Fiebig, P. and Williamson, G., Parity sheaves, moment graphs and the  $p$ -smooth locus of Schubert varieties, Ann. Inst. Fourier (Grenoble), to appear, arXiv:1008.0719.Google Scholar
Jantzen, J. C., Representations of algebraic groups, Mathematical Surveys and Monographs, vol. 107, second edition (American Mathematical Society, Providence, RI, 2003).Google Scholar
Juteau, D., Decomposition numbers for perverse sheaves, Ann. Inst. Fourier (Grenoble) 59 (2009), 1177–1229.Google Scholar
Juteau, D., Mautner, C. and Williamson, G., Parity sheaves, Preprint (2009), arXiv:0906.2994.Google Scholar
Kazhdan, D. and Lusztig, G., Schubert varieties and Poincaré duality, in Geometry of the Laplace operator (Proc. Sympos. Pure Math., Univ. Hawaii, Honolulu, Hawaii, 1979), Proc. Sympos. Pure Math., XXXVI (American Mathematical Society, Providence, RI, 1980), 185–203.Google Scholar
Keller, B., Deriving DG categories, Ann. Sci. Éc. Norm. Super. (4) 27 (1994), 63–102.Google Scholar
Kiehl, R. and Weissauer, R., Weil conjectures, perverse sheaves and $l$ ’adic Fourier transform, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], vol. 42 (Springer, Berlin, 2001).Google Scholar
Lam, T. Y., A first course in noncommutative rings, Graduate Texts in Mathematics, vol. 131, second edition (Springer, New York, 2001).Google Scholar
Milne, J. S., Étale cohomology, Princeton Mathematical Series, vol. 33 (Princeton University Press, Princeton, NJ, 1980).Google Scholar
Riche, S., Koszul duality and modular representations of semisimple Lie algebras, Duke Math. J. 154 (2010), 31–134.Google Scholar
Riche, S. and Williamson, G., Formality of modular constructible sheaves on the flag variety, unpublished preprint (2011).Google Scholar
Rickard, J., Morita theory for derived categories, J. Lond. Math. Soc. (2) 39 (1989), 436–456.CrossRefGoogle Scholar
Schnürer, O. M., Equivariant sheaves on flag varieties, Math. Z. 267 (2011), 27–80.Google Scholar
Deligne, P., Séminaire de Géométrie Algébrique du Bois-Marie– Cohomologie étale ( $\mathrm{SGA} ~4\frac{1}{2} $ ), Lecture Notes in Mathematics, vol. 569 (Springer, Berlin, 1977), avec la collaboration de J. F. Boutot, A. Grothendieck, L. Illusie et J. L. Verdier.Google Scholar
Grothendieck, A., Séminaire de Géometrie Algébrique du Bois-Marie 1965–1966– Cohomologie l-adique et fonctions L (SGA 5), Lecture Notes in Mathematics, vol. 589 (Springer, Berlin, 1977).Google Scholar
Soergel, W., $\mathfrak{n}$ -cohomology of simple highest weight modules on walls and purity, Invent. Math. 98 (1989), 565–580.CrossRefGoogle Scholar
Soergel, W., On the relation between intersection cohomology and representation theory in positive characteristic, J. Pure Appl. Algebra 152 (2000), 311–335; Commutative algebra, homological algebra and representation theory (Catania/Genoa/Rome, 1998).Google Scholar
Soergel, W., Modulare Koszul–Dualität, Preprint (2011), arXiv:1109.0563.Google Scholar
Williamson, G. and Braden, T., Modular intersection cohomology complexes on flag varieties, Math. Z. 272 (2012), 697–727.Google Scholar
Yun, Z., Weights of mixed tilting sheaves and geometric Ringel duality, Selecta Math. (N.S.) 14 (2009), 299–320.Google Scholar