Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Milanov, Todor
2014.
Analyticity of the total ancestor potential in singularity theory.
Advances in Mathematics,
Vol. 255,
Issue. ,
p.
217.
Milanov, Todor
2014.
The Eynard–Orantin recursion for the total ancestor potential.
Duke Mathematical Journal,
Vol. 163,
Issue. 9,
Bakalov, Bojko
and
Elsinger, Jason
2015.
Orbifolds of lattice vertex algebras under an isometry of order two.
Journal of Algebra,
Vol. 441,
Issue. ,
p.
57.
Bertola, Marco
and
Yang, Di
2015.
The partition function of the extendedr-reduced Kadomtsev–Petviashvili hierarchy.
Journal of Physics A: Mathematical and Theoretical,
Vol. 48,
Issue. 19,
p.
195205.
Bertola, Marco
Dubrovin, Boris
and
Yang, Di
2016.
Simple Lie Algebras and Topological ODEs.
International Mathematics Research Notices,
p.
rnw285.
Bakalov, Bojko
and
Wheeless, William
2016.
Additional symmetries of the extended bigraded Toda hierarchy.
Journal of Physics A: Mathematical and Theoretical,
Vol. 49,
Issue. 5,
p.
055201.
Safronov, Pavel
2016.
Virasoro constraints in Drinfeld–Sokolov hierarchies.
Selecta Mathematica,
Vol. 22,
Issue. 1,
p.
27.
Milanov, Todor
and
Lewanski, Danilo
2016.
𝒲-Algebra constraints and topological recursion for AN-singularity (with an Appendix by Danilo Lewanski).
International Journal of Mathematics,
Vol. 27,
Issue. 13,
p.
1650110.
Bakalov, Bojko
2016.
Twisted Logarithmic Modules of Vertex Algebras.
Communications in Mathematical Physics,
Vol. 345,
Issue. 1,
p.
355.
Milanov, Todor
Shen, Yefeng
and
Tseng, Hsian-Hua
2016.
Gromov–Witten theory of Fano orbifold curves, Gamma integral structures and ADE-Toda hierarchies.
Geometry & Topology,
Vol. 20,
Issue. 4,
p.
2135.
Bakalov, Bojko
and
Sullivan, McKay
2016.
Twisted logarithmic modules of free field algebras.
Journal of Mathematical Physics,
Vol. 57,
Issue. 6,
Milanov, Todor
2018.
B-Model Gromov-Witten Theory.
p.
539.
Arakawa, Tomoyuki
Creutzig, Thomas
and
Linshaw, Andrew R.
2019.
W-algebras as coset vertex algebras.
Inventiones mathematicae,
Vol. 218,
Issue. 1,
p.
145.
Milanov, Todor
2019.
The period map for quantum cohomology of P2.
Advances in Mathematics,
Vol. 351,
Issue. ,
p.
804.
Casper, W. Riley
and
Yakimov, Milen T.
2020.
Integral operators, bispectrality and growth of Fourier algebras.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2020,
Issue. 766,
p.
151.
Bakalov, Bojko
and
Yadavalli, Anila
2020.
Darboux transformations and Fay identities for the extended bigraded Toda hierarchy.
Journal of Physics A: Mathematical and Theoretical,
Vol. 53,
Issue. 6,
p.
065202.
Cheng, Jipeng
and
Milanov, Todor
2021.
The extended D-Toda hierarchy.
Selecta Mathematica,
Vol. 27,
Issue. 2,
Valeri, Daniele
2021.
Quantum Theory and Symmetries.
p.
181.
Alexandrov, Alexander
and
Milanov, Todor
2021.
Matrix model for the total descendant potential of a simple singularity of type D.
Letters in Mathematical Physics,
Vol. 111,
Issue. 4,
Cheng, Jipeng
and
Milanov, Todor
2021.
The 2-Component BKP Grassmannian and Simple Singularities of Type D
.
International Mathematics Research Notices,
Vol. 2021,
Issue. 23,
p.
17875.
 $\mathcal {W}$-constraints for the total descendant potential of a simple singularity
$\mathcal {W}$-constraints for the total descendant potential of a simple singularity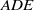 $ADE$. Let
$ADE$. Let 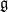 $\mathfrak {g}$ be the corresponding finite-dimensional Lie algebra, and
$\mathfrak {g}$ be the corresponding finite-dimensional Lie algebra, and 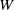 $W$ its Weyl group. The set of
$W$ its Weyl group. The set of 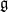 $\mathfrak {g}$-invariants in the basic representation of the affine Kac–Moody algebra
$\mathfrak {g}$-invariants in the basic representation of the affine Kac–Moody algebra  $\hat {\mathfrak {g}}$ is known as a
$\hat {\mathfrak {g}}$ is known as a  $\mathcal {W}$-algebra and is a subalgebra of the Heisenberg vertex algebra
$\mathcal {W}$-algebra and is a subalgebra of the Heisenberg vertex algebra 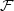 $\mathcal {F}$. Using period integrals, we construct an analytic continuation of the twisted representation of
$\mathcal {F}$. Using period integrals, we construct an analytic continuation of the twisted representation of 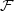 $\mathcal {F}$. Our construction yields a global object, which may be called a
$\mathcal {F}$. Our construction yields a global object, which may be called a  $W$-twisted representation of
$W$-twisted representation of  $\mathcal {F}$. Our main result is that the total descendant potential of the singularity, introduced by Givental, is a highest-weight vector for the
$\mathcal {F}$. Our main result is that the total descendant potential of the singularity, introduced by Givental, is a highest-weight vector for the 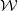 $\mathcal {W}$-algebra.
$\mathcal {W}$-algebra.

